Similar presentations:
Displacement method. The degree of kinematic indeterminacy of the system. Lecture 13
1. Lecture 13 Displacement method. The degree of kinematic indeterminacy of the system
nк n y n лnк
- the degree of kinematic uncertainty determines the number
of independent angular and linear displacements of nodes that determine the
deformation of the system;
ny
– determines the number of independent angular displacements and is
equal to the number of rigid nodes of the system;
nл
– determines the number of independent
linear displacements of nodes and is equal to the degree of geometric
variability of the hinged scheme (the hinged scheme is obtained from a given
system by introducing hinges into all nodes, including the support ones).
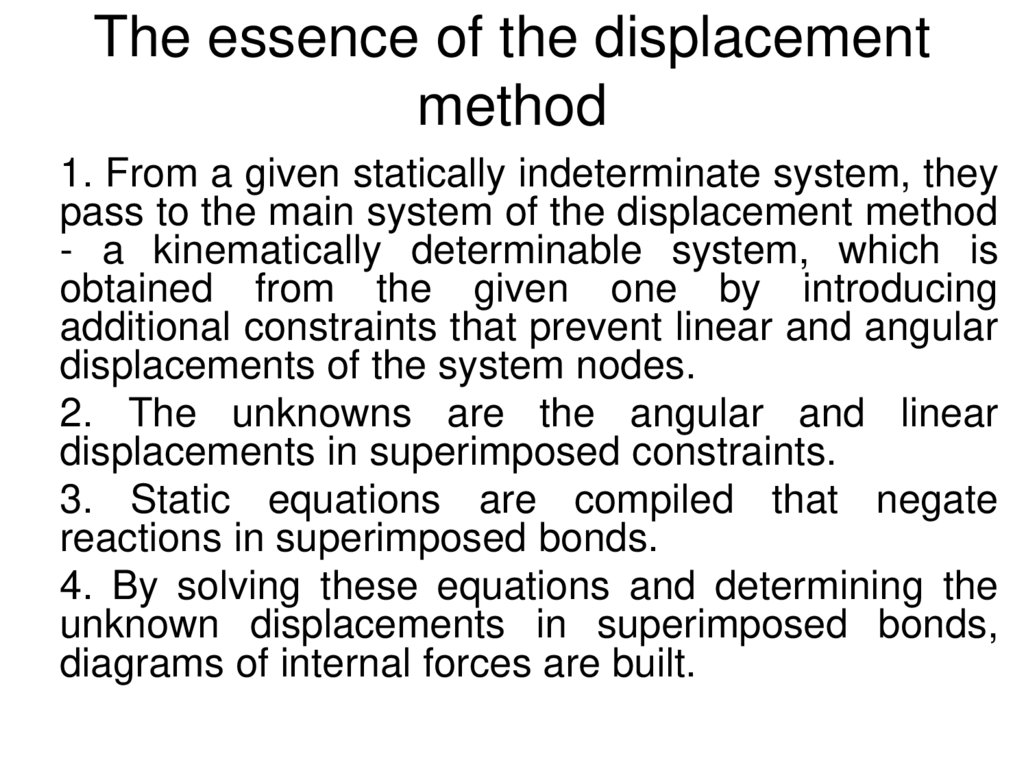
2. The essence of the displacement method
1. From a given statically indeterminate system, theypass to the main system of the displacement method
- a kinematically determinable system, which is
obtained from the given one by introducing
additional constraints that prevent linear and angular
displacements of the system nodes.
2. The unknowns are the angular and linear
displacements in superimposed constraints.
3. Static equations are compiled that negate
reactions in superimposed bonds.
4. By solving these equations and determining the
unknown displacements in superimposed bonds,
diagrams of internal forces are built.
3. Differences between the force method and the displacement method
The basic system of the method of forces is obtainedby removing superfluous bonds; the unknowns are
the reactions of the discarded bonds; The basic
system of the displacement method is obtained by
introducing additional constraints; the unknowns are
displacements in superimposed constraints;
The basic system of the force method is a statically
determinate system; The basic system of the
displacement method is a kinematically determinable
system.
4. Additional links in the main system of the displacement method
Additional angular bonds prevent only the rotation of thenodes, they are schematically depicted as a seal, the
unknown angular displacement is denoted Zi , i.e. angle
of rotation in "i" superimposed link. The number of such
bonds is determined by the value ny. It should be noted
that these embeds differ from the usual embed in that
they only interfere with the rotation of the node and do
not deprive it of linear mobility. They are called sliding
closures.
Additional linear connections prevent only translational
displacements, they are conventionally depicted as a
support rod, an unknown displacement in it is also
denoted Zi, linear offset in "i" superimposed connection.
The number of such bonds is determined by the value n
л
5. The basic system of the displacement method
nк n y n л 2 1 36. Quantity definition (HINGE SCHEME)
Quantity definition n л(HINGE SCHEME)
nл W 1
7.
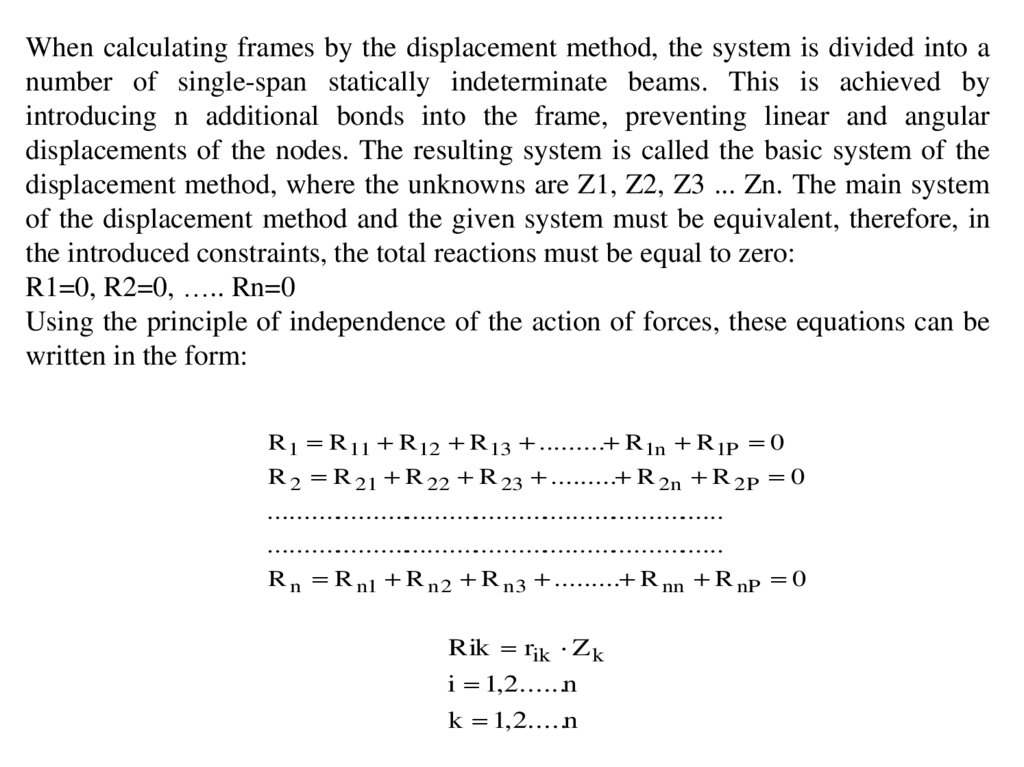
When calculating frames by the displacement method, the system is divided into anumber of single-span statically indeterminate beams. This is achieved by
introducing n additional bonds into the frame, preventing linear and angular
displacements of the nodes. The resulting system is called the basic system of the
displacement method, where the unknowns are Z1, Z2, Z3 ... Zn. The main system
of the displacement method and the given system must be equivalent, therefore, in
the introduced constraints, the total reactions must be equal to zero:
R1=0, R2=0, ….. Rn=0
Using the principle of independence of the action of forces, these equations can be
written in the form:
R 1 R 11 R 12 R 13 ......... R 1n R 1P 0
R 2 R 21 R 22 R 23 ......... R 2n R 2 P 0
..................................................................
..................................................................
R n R n1 R n 2 R n 3 ......... R nn R nP 0
Rik rik Z k
i 1,2......n
k 1,2.....n
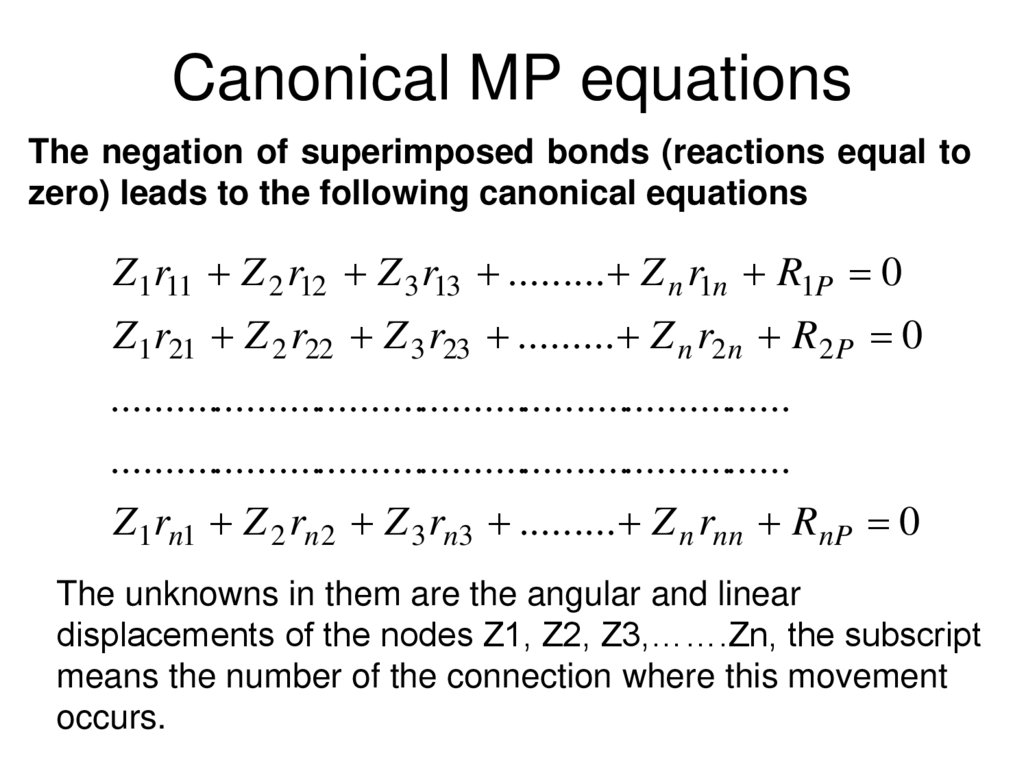
8. Canonical MP equations
The negation of superimposed bonds (reactions equal tozero) leads to the following canonical equations
Z 1 r11 Z 2 r12 Z 3 r13 ......... Z n r1n R1P 0
Z 1 r21 Z 2 r22 Z 3 r23 ......... Z n r2n R2 P 0
..................................................................
..................................................................
Z 1 rn1 Z 2 rn 2 Z 3 rn3 ......... Z n rnn RnP 0
The unknowns in them are the angular and linear
displacements of the nodes Z1, Z2, Z3,…….Zn, the subscript
means the number of the connection where this movement
occurs.
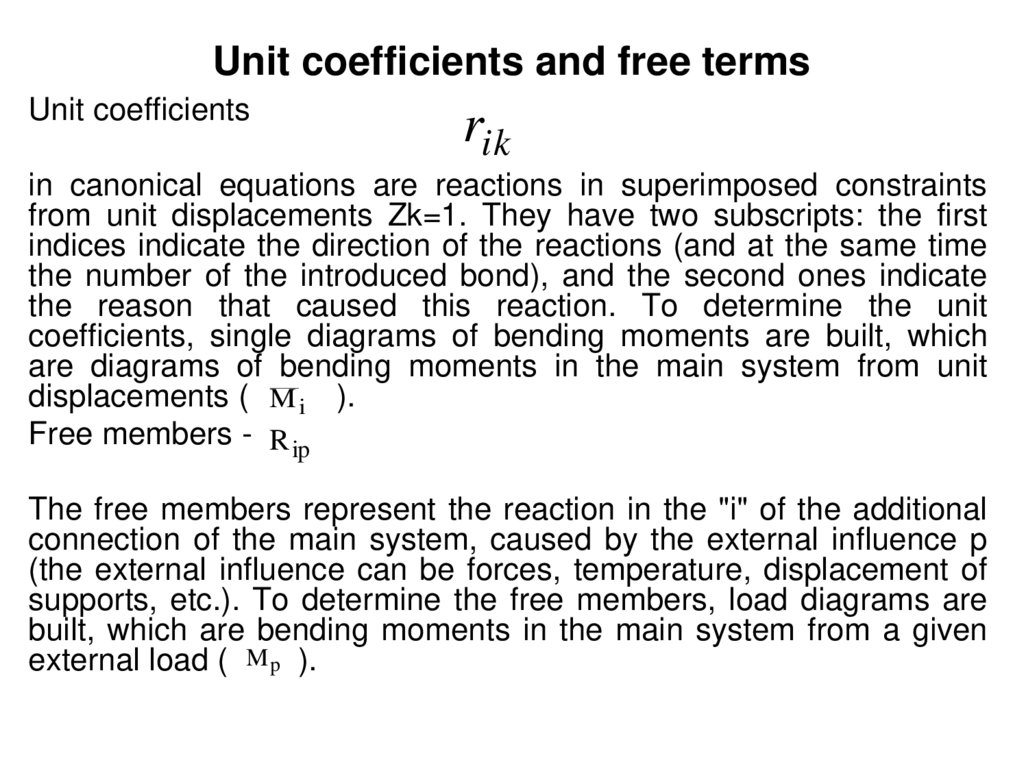
9. Unit coefficients and free terms
Unit coefficientsrik
in canonical equations are reactions in superimposed constraints
from unit displacements Zk=1. They have two subscripts: the first
indices indicate the direction of the reactions (and at the same time
the number of the introduced bond), and the second ones indicate
the reason that caused this reaction. To determine the unit
coefficients, single diagrams of bending moments are built, which
are diagrams of bending moments in the main system from unit
displacements ( M i ).
Free members - R ip
The free members represent the reaction in the "i" of the additional
connection of the main system, caused by the external influence p
(the external influence can be forces, temperature, displacement of
supports, etc.). To determine the free members, load diagrams are
built, which are bending moments in the main system from a given
external load ( M p ).
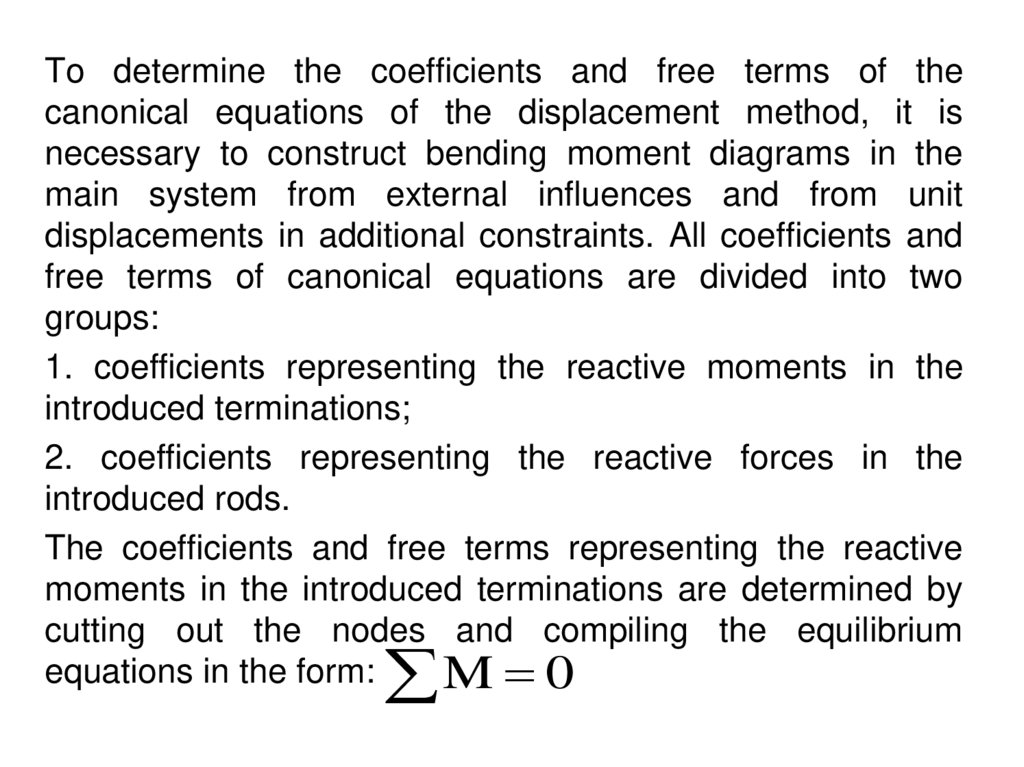
10.
To determine the coefficients and free terms of thecanonical equations of the displacement method, it is
necessary to construct bending moment diagrams in the
main system from external influences and from unit
displacements in additional constraints. All coefficients and
free terms of canonical equations are divided into two
groups:
1. coefficients representing the reactive moments in the
introduced terminations;
2. coefficients representing the reactive forces in the
introduced rods.
The coefficients and free terms representing the reactive
moments in the introduced terminations are determined by
cutting out the nodes and compiling the equilibrium
equations in the form:
M 0
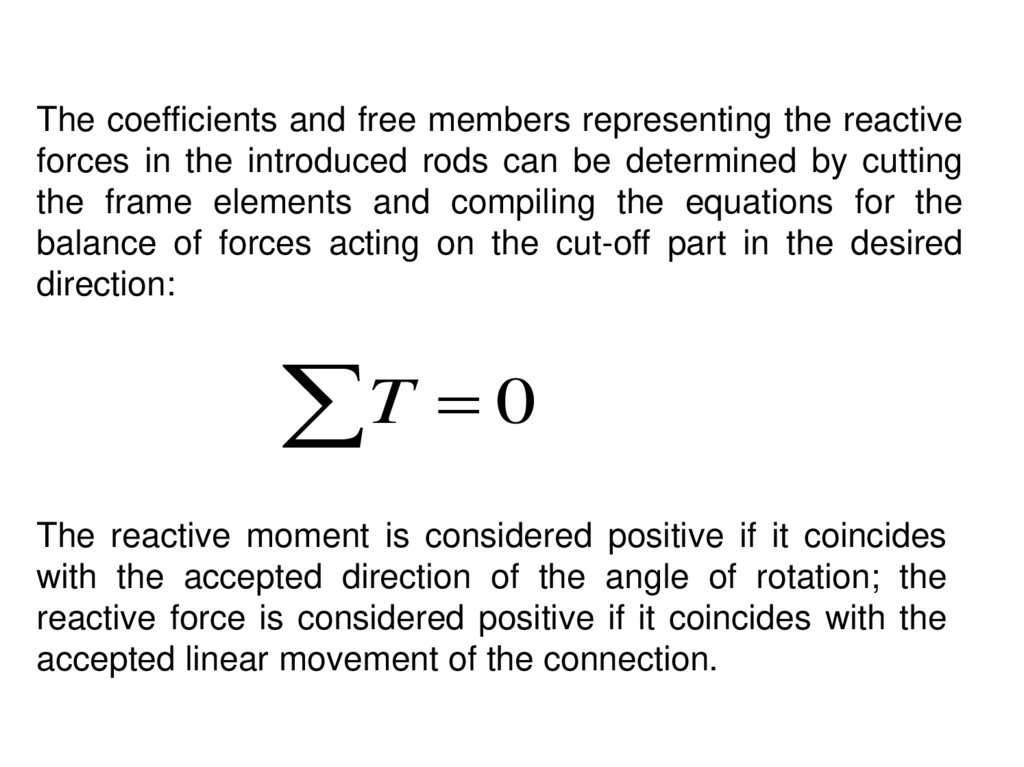
11.
The coefficients and free members representing the reactiveforces in the introduced rods can be determined by cutting
the frame elements and compiling the equations for the
balance of forces acting on the cut-off part in the desired
direction:
T 0
The reactive moment is considered positive if it coincides
with the accepted direction of the angle of rotation; the
reactive force is considered positive if it coincides with the
accepted linear movement of the connection.





 mathematics
mathematics physics
physics








