Similar presentations:
The function of one variable
1.
CALCULAS studies the relationshipsthat exists between one collection of
objects and another
2. Literature: 1, v. І, p. 174-180, 2, part І, p. 40-57, 3, p. 174-181.
Theme: The function of one variableLiterature:
1 , v. І, p. 174-180,
2 , part І, p. 40-57,
3 , p. 174-181.
3.
1.The main definitions2.The different ways of representing of the
functions
3. The main characteristics of behavior of the
function
4. The basic elementary functions
5.The composite function
6.The elementary functions
4.
Given two sets X and YDefinition. A function is a rule which assigns
to each element x of X one and only one
element y of Y.
Notation: y=f(x)
x - the independent variable
y - the dependent variable
The set X - the domain of the function (D(y))
The set of all corresponding values of y - the
range of the function (E(y))
5.
Examples. D(y) - ?1) f(x)=x3-4x+2 (polynomial of the third power)
1
4) y
1
x 2
2) y
x 2
x 2
1
5) y ln
x 4
.
.. .
3)
y x 2
3
6) y
x 4x 4
3
2
x 2 x 3x
2
6. The most important ways of representing of the functions:
- the analytic method;- the tabular method;
- the graphical method
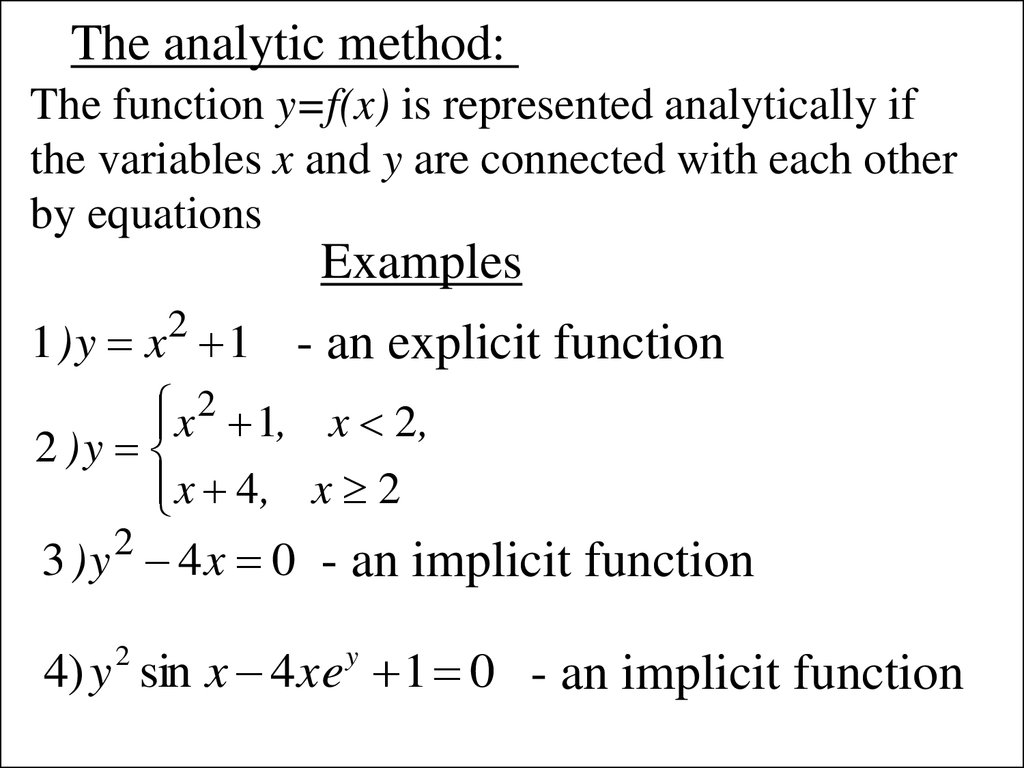
7.
The analytic method:The function y=f(x) is represented analytically if
the variables x and y are connected with each other
by equations
Examples
1 ) y x 2 1 - an explicit function
x 2 1, x 2 ,
2 )y
x 4 , x 2
2
3 ) y 4 x 0 - an implicit function
4) y sin x 4 xe 1 0 - an implicit function
2
y
8.
5) The demand function:400
p
q 3
q - price, p – demand
6) Cost function V(x), income function D(x),
profit function P(x),
where x – the volume of production
9.
The tabular method:x
y
x1
y1
x2
…
y2
…
xn
yn
10.
The graphical method:y
y=f(x)
x
11.
The main ways of the graph transformations1) Right-left translation:
Example: y=(x-1)2
12.
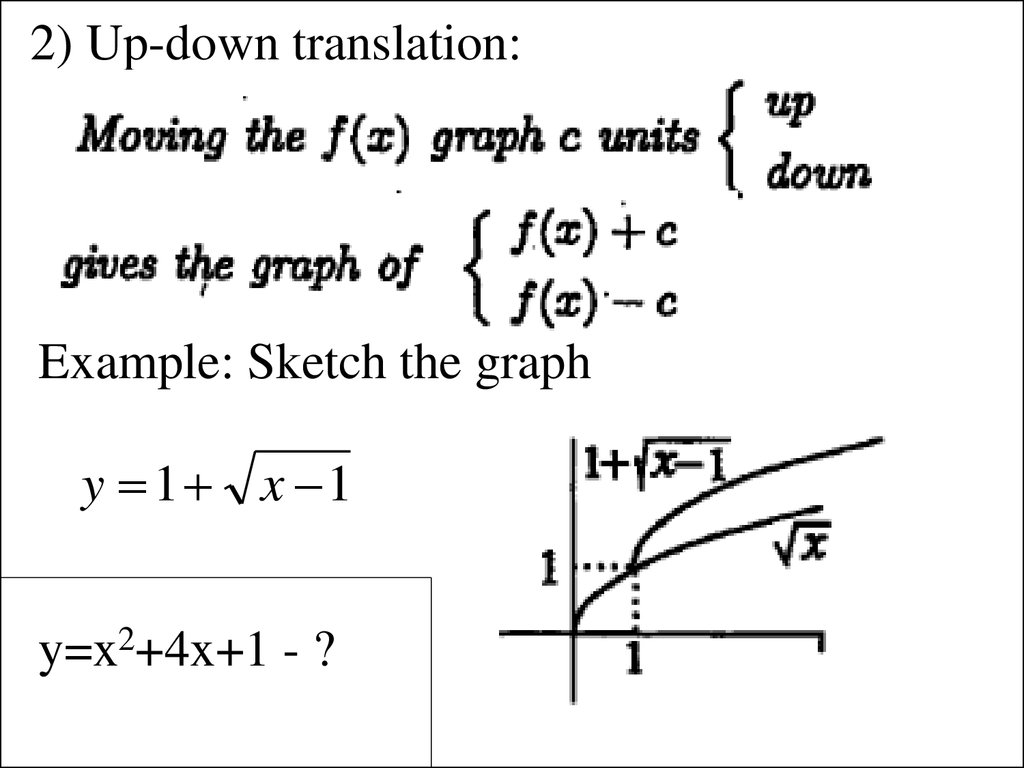
2) Up-down translation:Example: Sketch the graph
y 1 x 1
y=x2+4x+1 - ?
13.
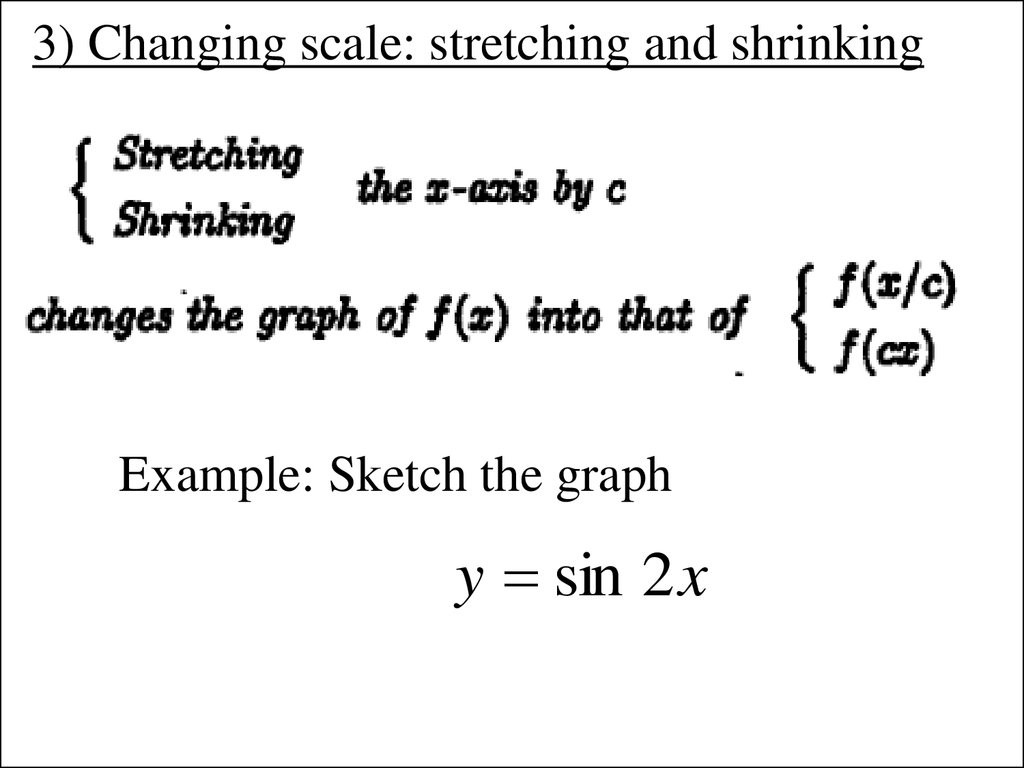
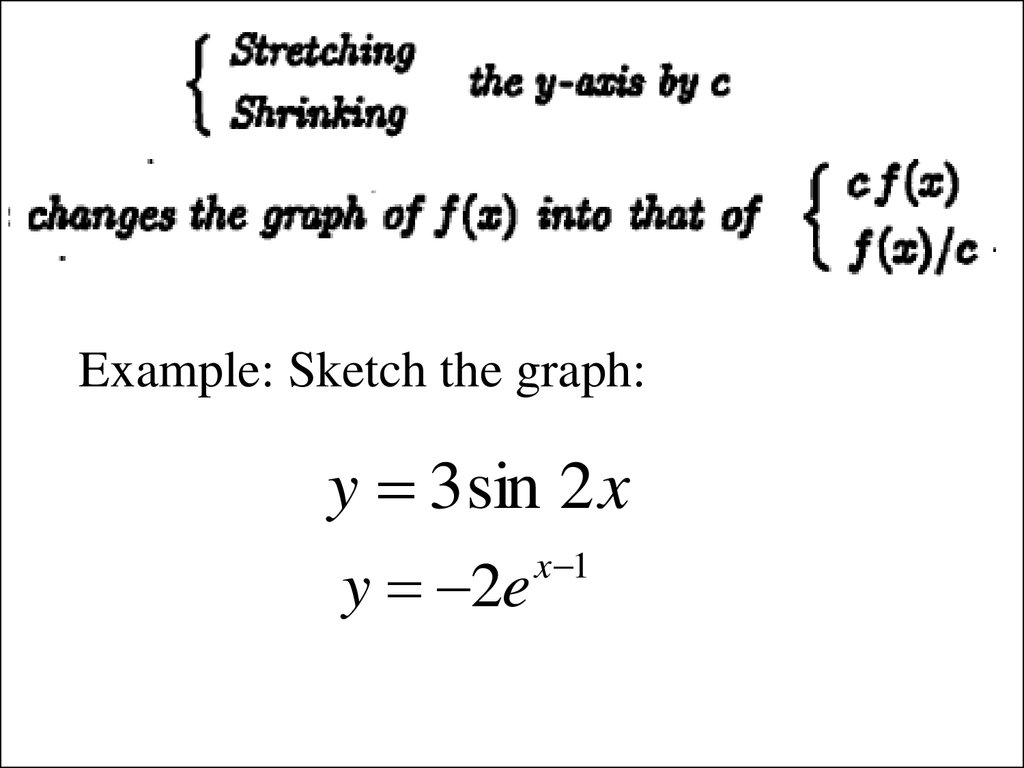
3) Changing scale: stretching and shrinkingExample: Sketch the graph
y sin 2 x
14.
Example: Sketch the graph:y 3 sin 2 x
y 2e
x 1
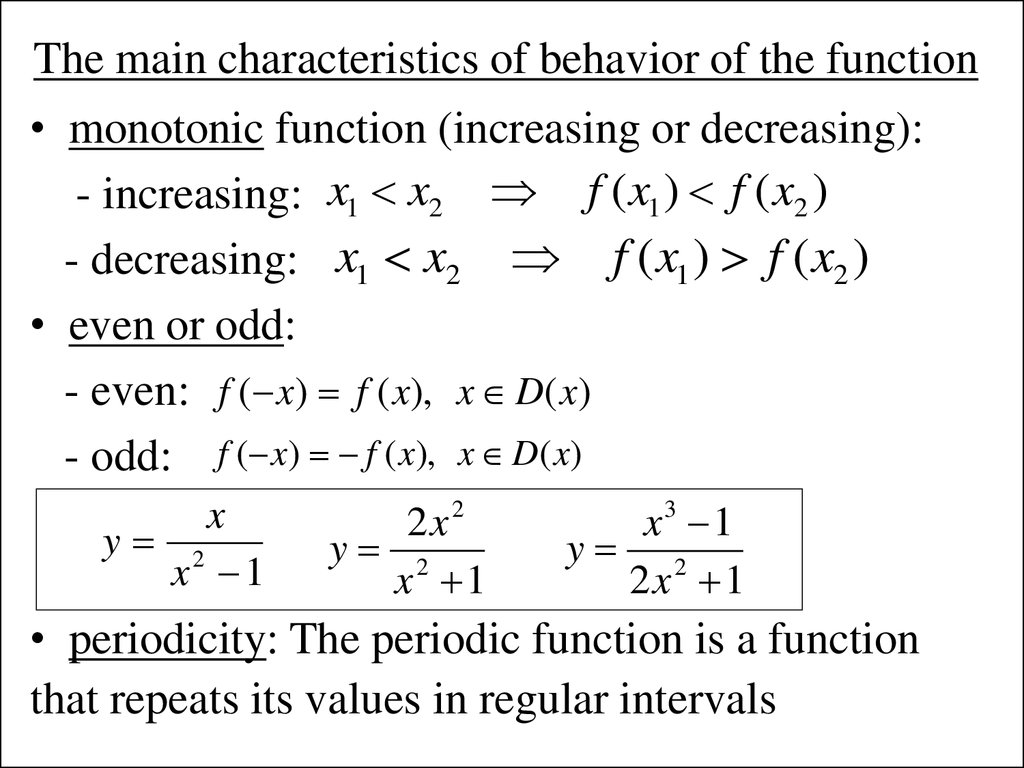
15. The main characteristics of behavior of the function
• monotonic function (increasing or decreasing):- increasing: x1 x2 f ( x1 ) f ( x2 )
- decreasing: x1 x2 f ( x1 ) f ( x2 )
• even or odd:
- even: f ( x) f ( x), x D( x)
- odd: f ( x) f ( x), x D( x)
x
y 2
x 1
2
2x
y 2
x 1
x 1
y 2
2x 1
3
• periodicity: The periodic function is a function
that repeats its values in regular intervals
16. The basic elementary functions:
The power function;
The exponential function;
The logarithmic function;
The trigonometric functions ( 4 );
The inverse trigonometric functions ( 4 ).
17.
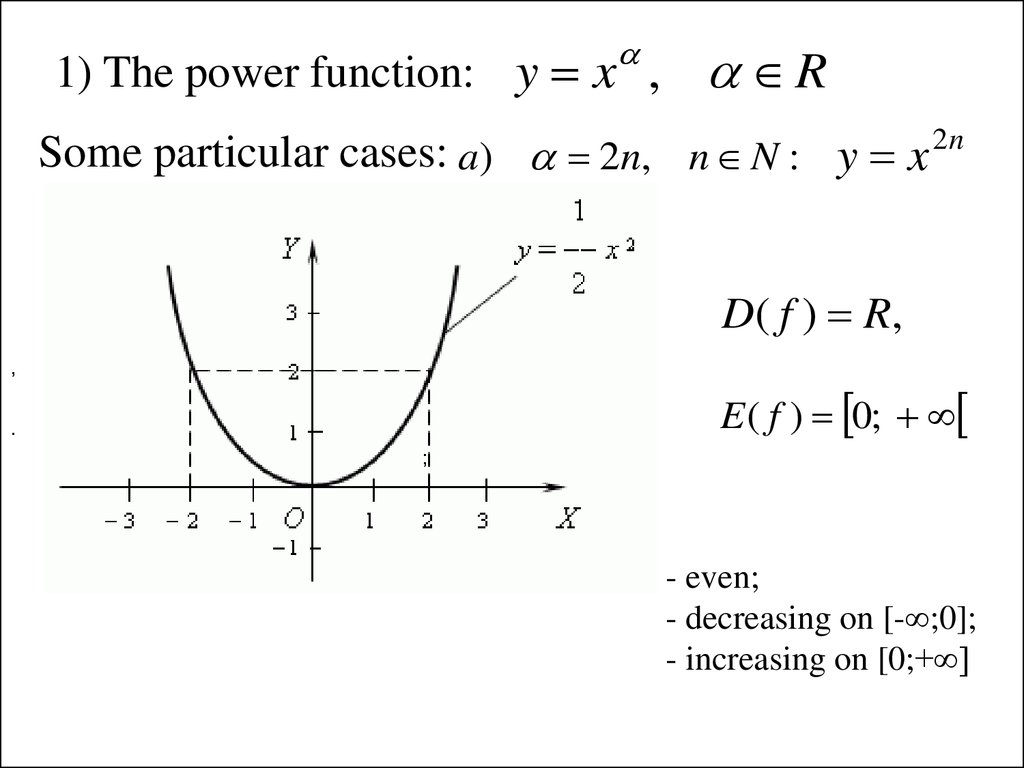
1) The power function: y x , RSome particular cases: a) 2n, n N : y x 2 n
D ( f ) R,
,
E ( f ) 0;
.
;
- even;
- decreasing on [-∞;0];
- increasing on [0;+∞]
18.
b) 2n 1, n N :y x
2 n 1
D ( f ) R,
,
:
;
.
E( f ) R
- odd;
- increasing on
D( f ) R
19.
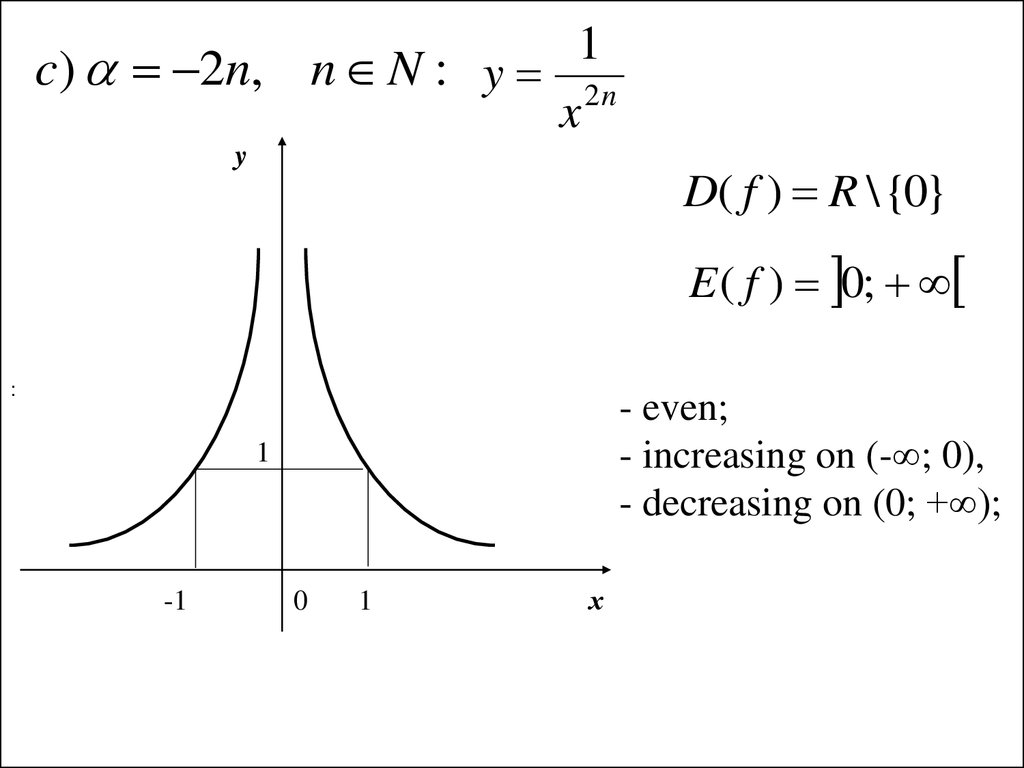
1c) 2n, n N : y 2 n
x
y
D( f ) R \ {0}
E ( f ) 0;
:
- even;
- increasing on (-∞; 0),
- decreasing on (0; +∞);
1
-1
0
1
х
20.
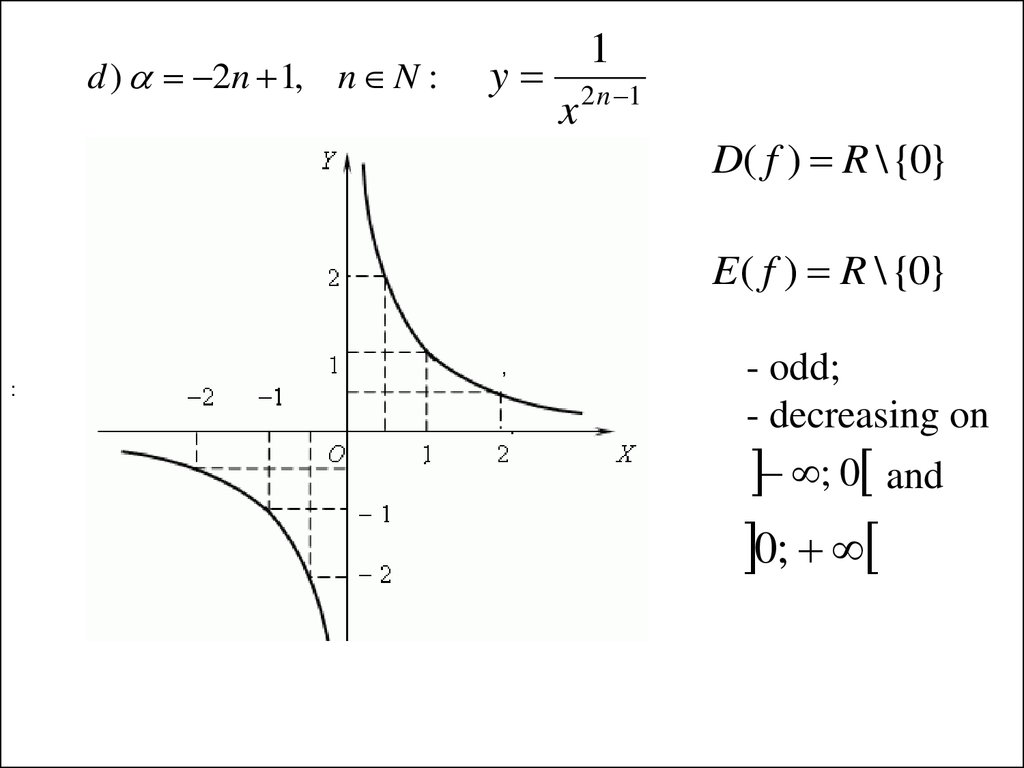
d ) 2n 1, n N :y
1
x 2 n 1
D( f ) R \ {0}
E ( f ) R \ {0}
,
:
.
.
- odd;
- decreasing on
; 0 and
0;
21.
2) The exponential function:y a x a 0; a 1
D( f ) R
E ( f ) 0;
(0<a<1)
(a>1)
,
,
.
If 0<a<1 then the function is decreasing,
if a>1 then the function is increasing.
22.
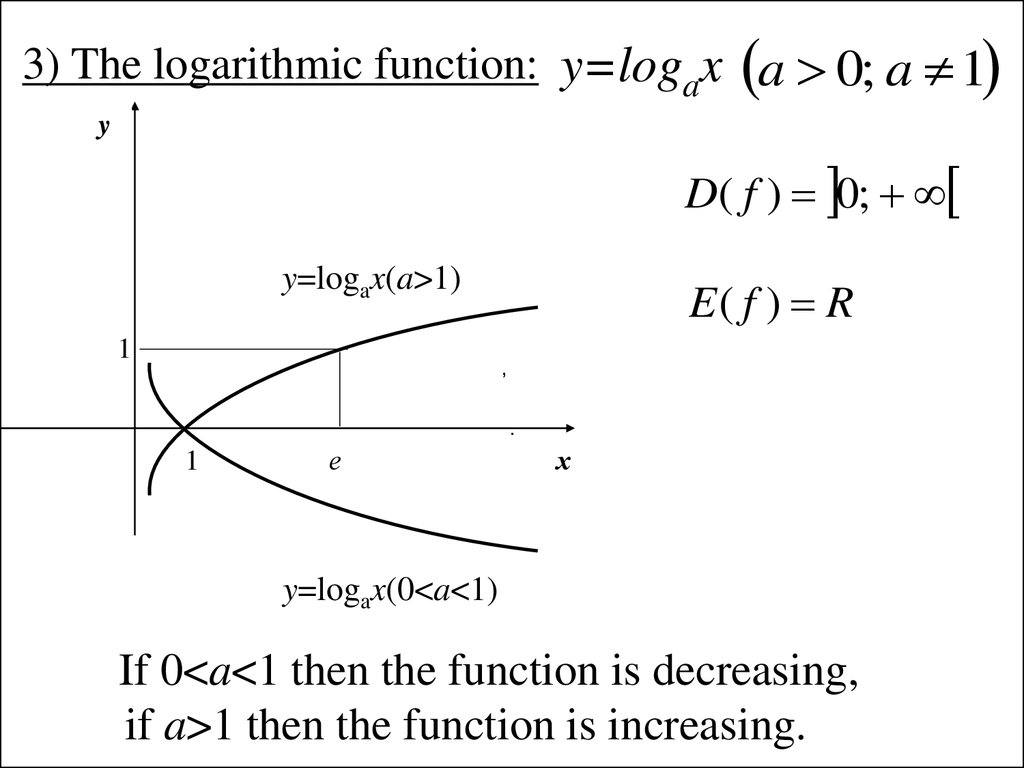
3) The logarithmic function: y=logax a 0; a 1y
D( f ) 0;
y=logax(a>1)
1
E( f ) R
,
.
1
е
х
y=logax(0<a<1)
If 0<a<1 then the function is decreasing,
if a>1 then the function is increasing.
23.
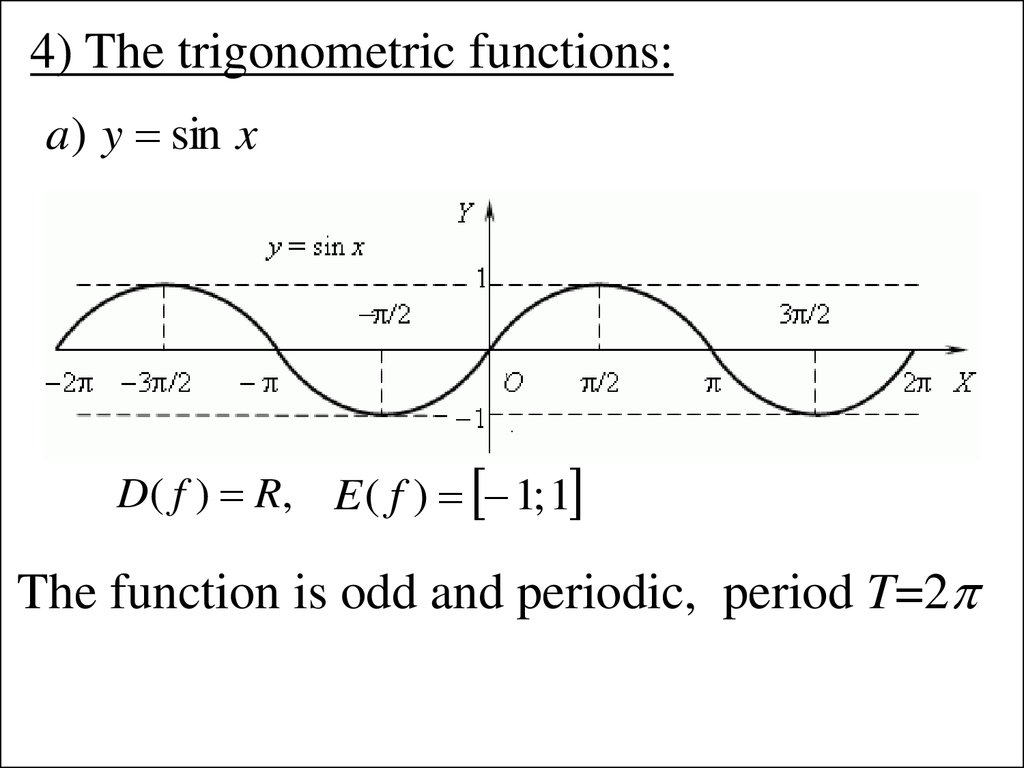
4) The trigonometric functions:a) y sin x
,
.
D( f ) R, E ( f ) 1; 1
The function is odd and periodic, period T=2
24.
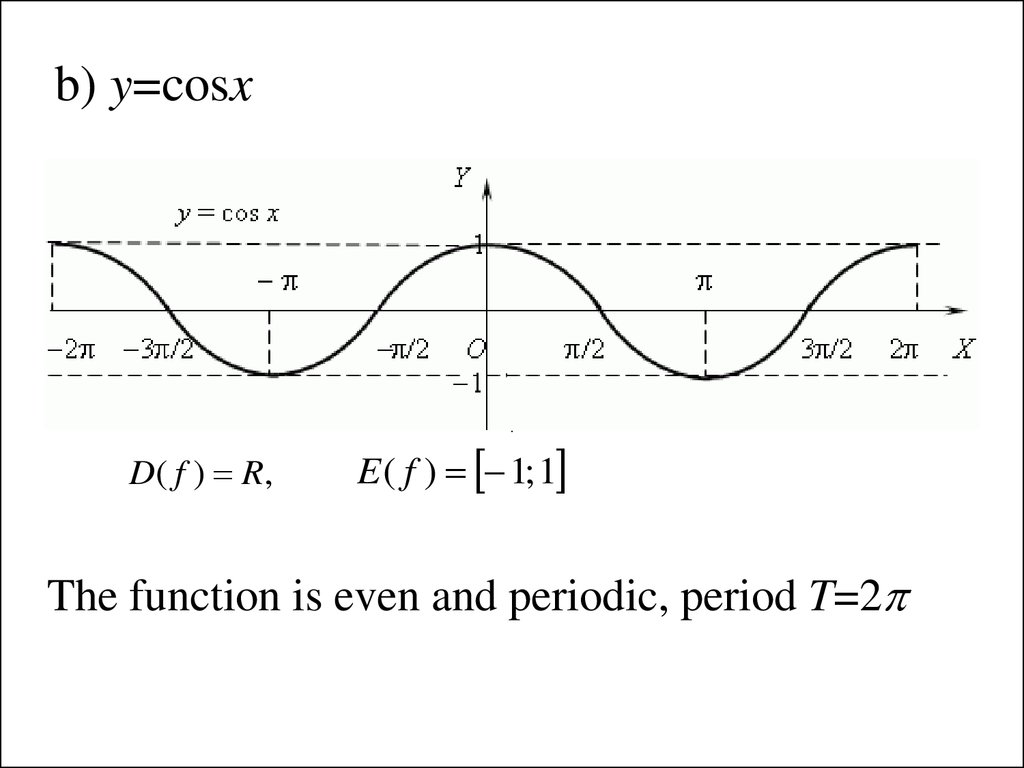
b) y=cosx,
.
D ( f ) R,
E ( f ) 1; 1
The function is even and periodic, period T=2
25.
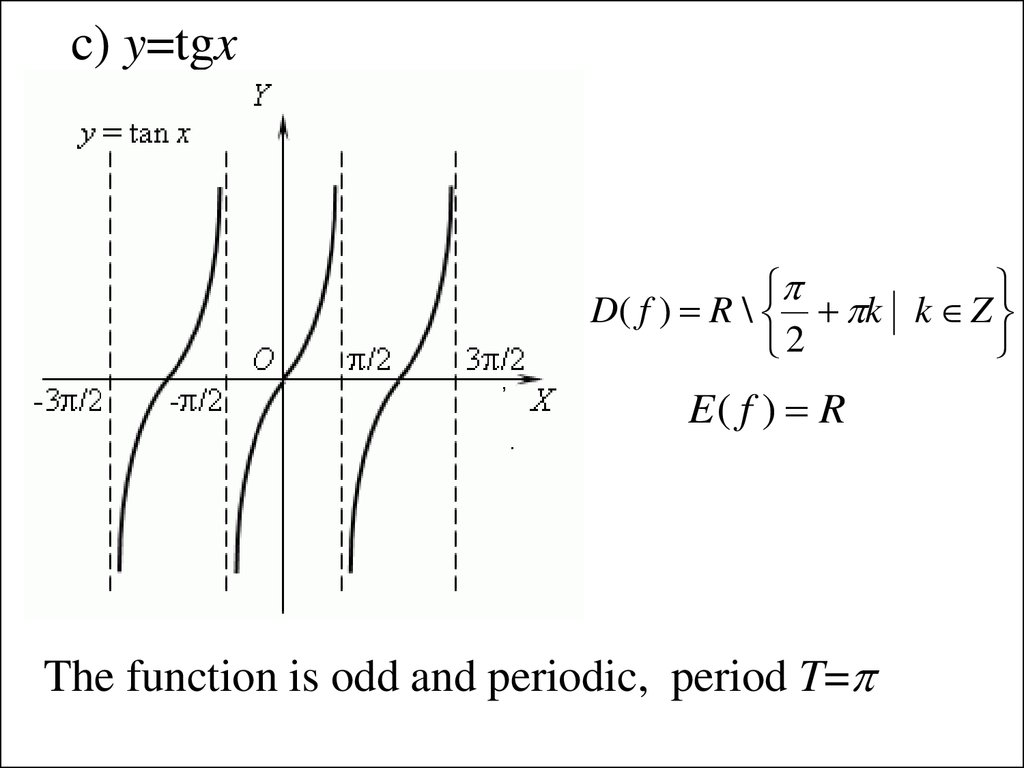
c) y=tgxD( f ) R \ k k Z
2
,
E( f ) R
.
The function is odd and periodic, period T=
26.
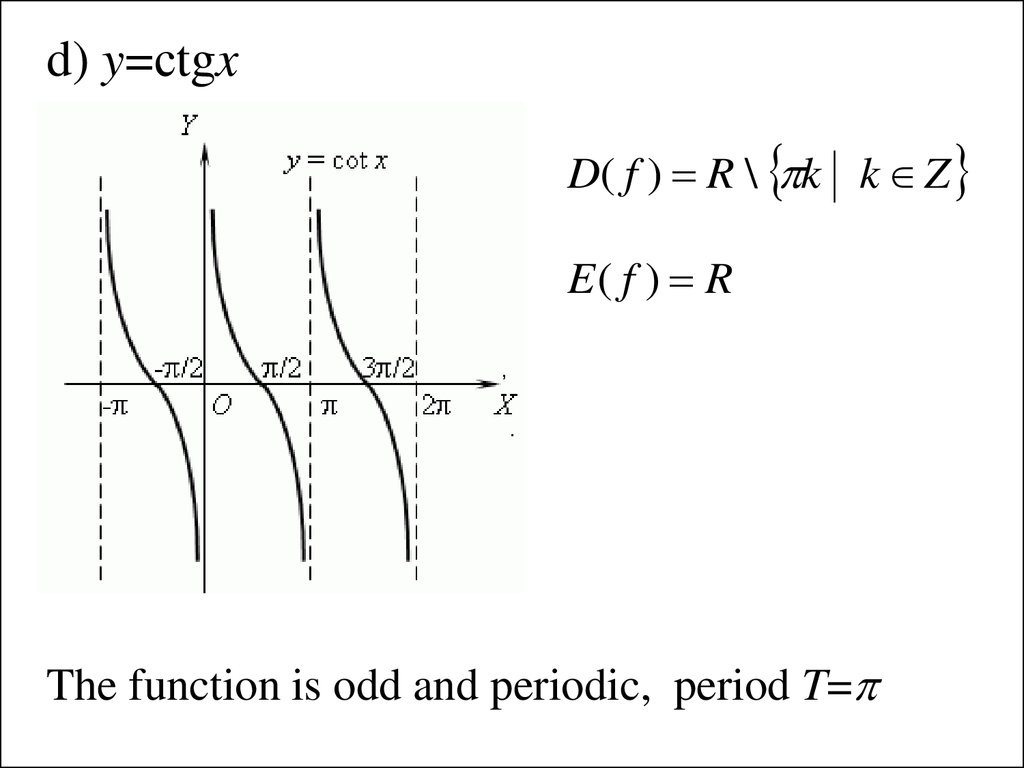
d) y=сtgxD( f ) R \ k k Z
E( f ) R
,
.
The function is odd and periodic, period T=
27.
5) The inverse trigonometric functions:а) y=arcsinx
D( f ) 1; 1
E ( f ) ;
2 2
,
.
The function is odd and increasing
28.
b) y=arccosxD( f ) 1; 1
E ( f ) 0;
,
.
The function is decreasing
29.
c) y=arctgx,E ( f ) R,
E( f ) ;
2 2
.
The function is odd and increasing
30.
d) y=arcсtgx,D( f ) ; , E ( f ) 0;
.
The function is decreasing
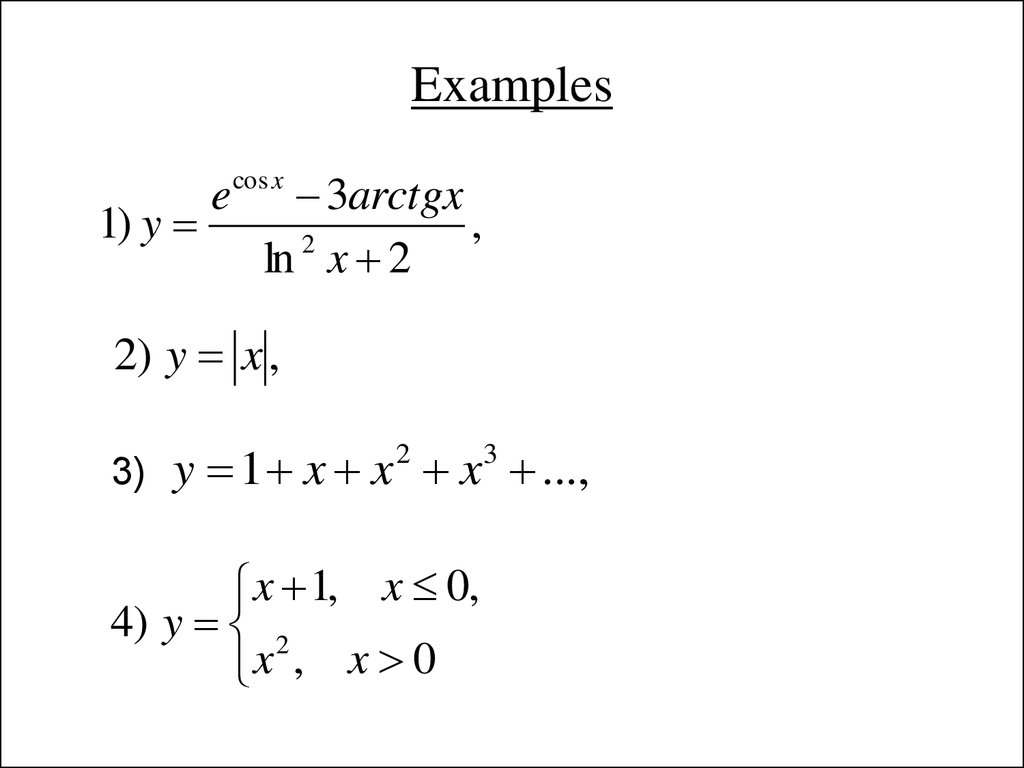
31. Examples
ecos x 3arctgx1) y
,
2
ln x 2
2) y x ,
3)
y 1 x x x ...,
2
x 1, x 0,
4) y 2
x , x 0
3
32.
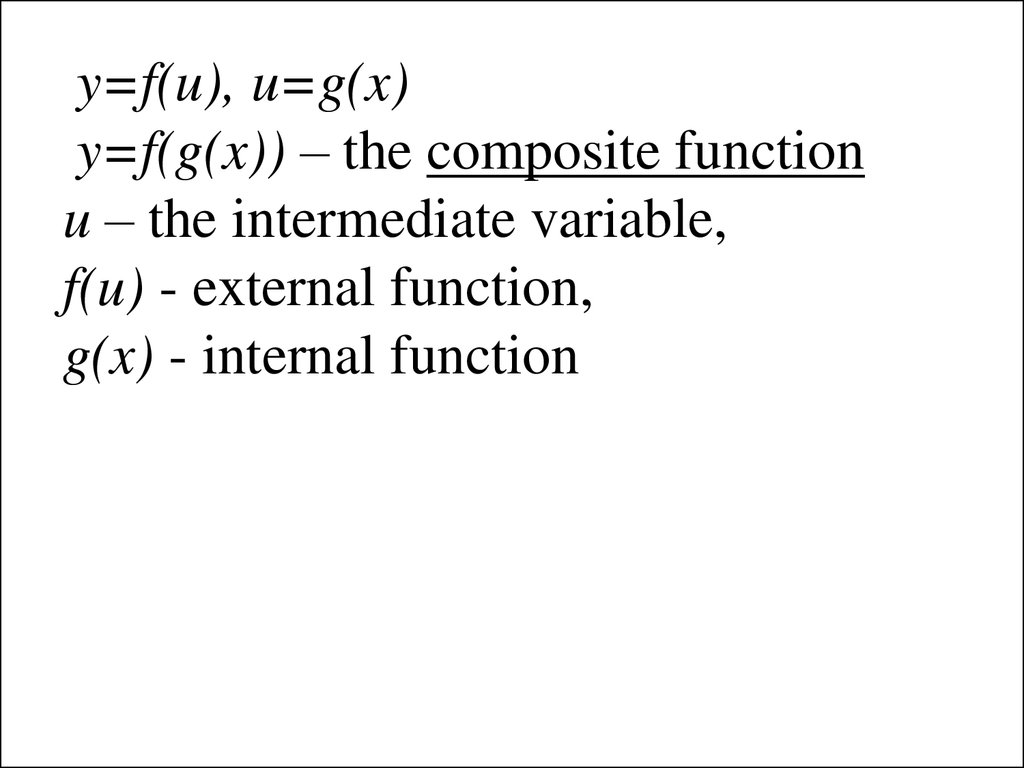
y=f(u), u=g(x)y=f(g(x)) – the composite function
u – the intermediate variable,
f(u) - external function,
g(x) - internal function
33.
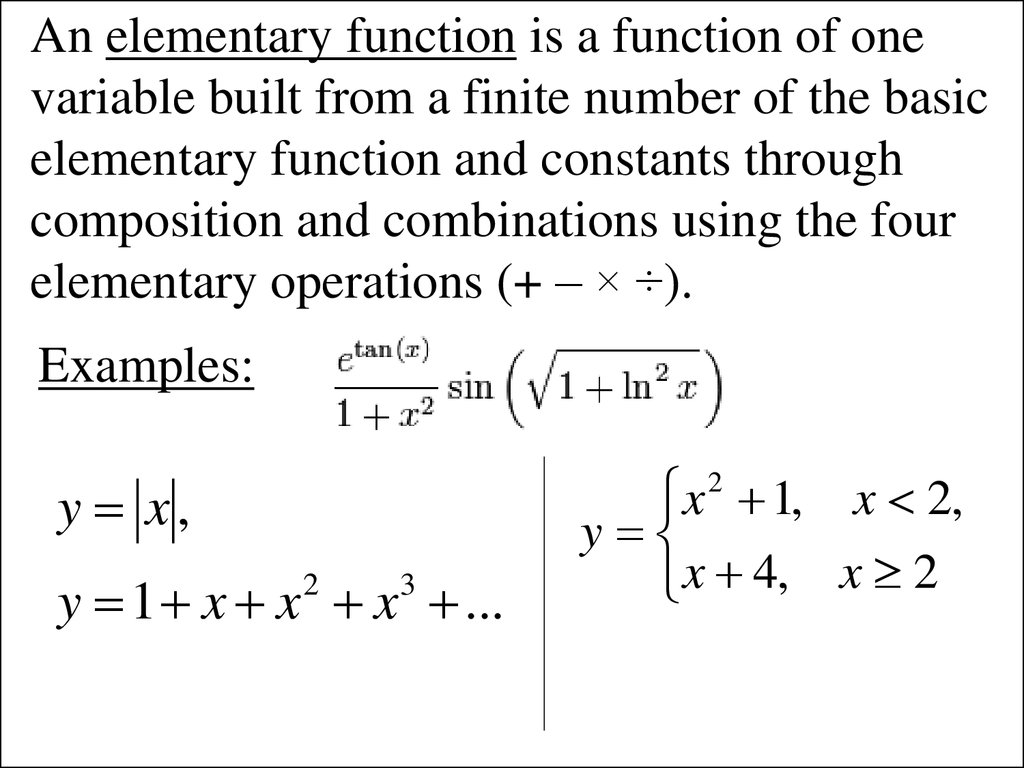
An elementary function is a function of onevariable built from a finite number of the basic
elementary function and constants through
composition and combinations using the four
elementary operations (+ – × ÷).
Examples:
y x,
y 1 x x x ...
2
3
x 2 1, x 2,
y
x 4, x 2
34.
The home work:Sketch the graph:
a) y=x2-4x+5;
b) y=3+2ln(x-1)
x
c) y 2 cos
2
















 mathematics
mathematics








