The triple integral. Properties of triple integrals. The calculation of the triple integral and volumes of solids

1. The triple integral. Properties of triple integrals. The calculation of the triple integral and volumes of solids.

THE TRIPLE INTEGRAL. PROPERTIES OF
TRIPLE
INTEGRALS. THE CALCULATION OF THE
TRIPLE
INTEGRAL AND VOLUMES OF SOLIDS.
Author of the presentation: Duysengalieva K., Marat Zhainagul.

2.

1. The physical meaning of the triple integral
If f (x; y; z)> 0 on U, the mass M of the body variable density γ = f (x; y; z) is calculated
using the formula:
2. The volume of the body

3. Evidence

EVIDENCE
Since f (x; y; z) = I> 0 to
Y, then
- Body weight at a density of γ = 1.
Therefore, M = γ · V = 1 · V = V. As a result, I =
V, as required.
3.
4.
5.
If U = U1 U2, where U1 and U2 do not intersect,

4.

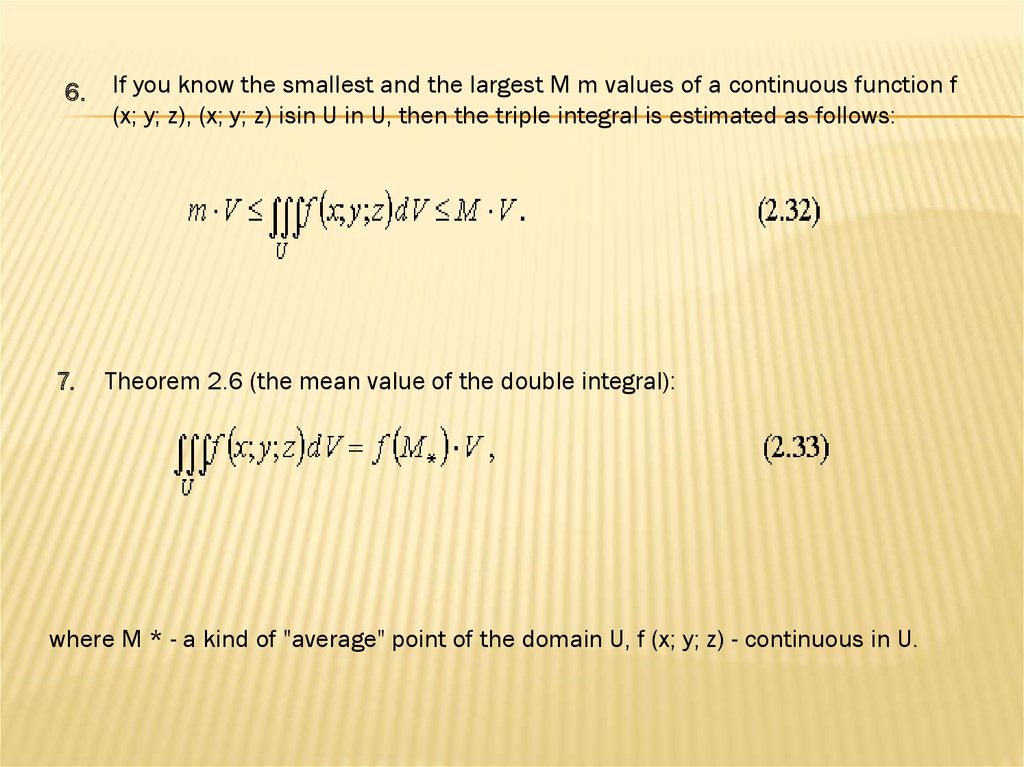
6.
7.
If you know the smallest and the largest M m values of a continuous function f
(x; y; z), (x; y; z) isin U in U, then the triple integral is estimated as follows:
Theorem 2.6 (the mean value of the double integral):
where M * - a kind of "average" point of the domain U, f (x; y; z) - continuous in U.

5. Evidence

EVIDENCE
Using the property
(6):
The number of I / U - intermediate value is a continuous function f (x; y; z), so there is a
point M * such that
eventually
Q.E.D.