Математика на английском языке

1. Analysis

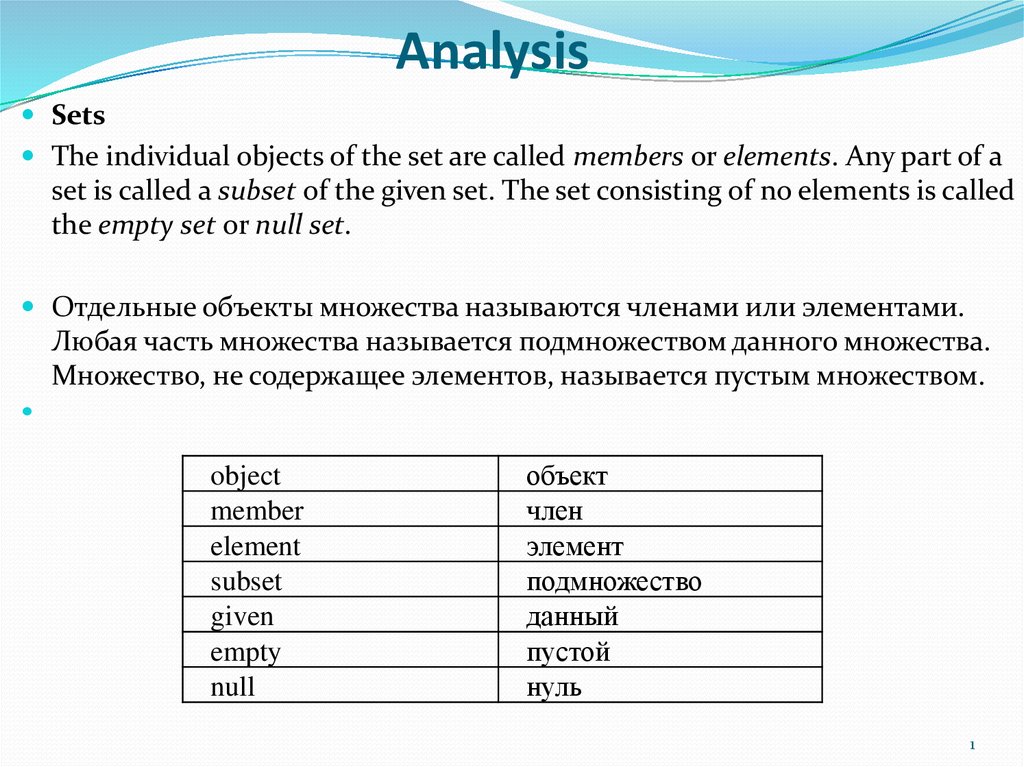
Sets
The individual objects of the set are called members or elements. Any part of a
set is called a subset of the given set. The set consisting of no elements is called
the empty set or null set.
Отдельные объекты множества называются членами или элементами.
Любая часть множества называется подмножеством данного множества.
Множество, не содержащее элементов, называется пустым множеством.
object
member
element
subset
given
empty
null
объект
член
элемент
подмножество
данный
пустой
нуль
1

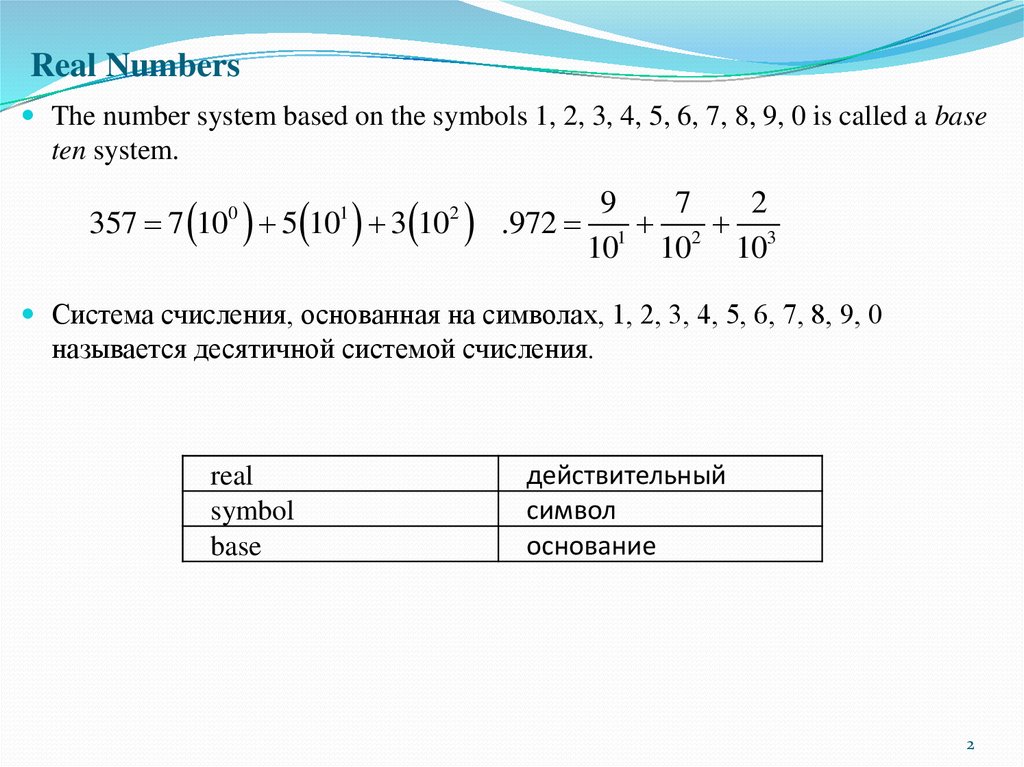
2. Real Numbers

The number system based on the symbols 1, 2, 3, 4, 5, 6, 7, 8, 9, 0 is called a base
ten system.
357 7 10 5 10 3 10
0
1
2
9
7
2
.972 1 2 3
10 10 10
Система счисления, основанная на символах, 1, 2, 3, 4, 5, 6, 7, 8, 9, 0
называется десятичной системой счисления.
real
symbol
base
действительный
символ
основание
2

3. Natural numbers

1. Natural numbers 1, 2, 3, 4, . . . , also called positive integers, are used in counting
members of a set. The sum a + b and product a・b or ab of any two natural numbers a and b
is also a natural number. The set of natural numbers is closed under the operations of addition
and multiplication, or satisfies the closure property with respect to these operations.
1. Натуральные числа 1, 2, 3, 4,..., называемые также положительными целыми,
используются при подсчете членов множества. Сумма а + b и произведение а · b или аb
любых двух натуральных чисел а и b также натуральное число. Множество
натуральных чисел замкнуто относительно операций сложения и умножения, или
удовлетворяет свойству замкнутости относительно этих операций.
natural
positive
integer
count
operation
closure
property
with respect to
натуральный
положительный
целый
считать
операция
замкнутый
свойство
по отношению к
3

4. Integer numbers

2. Negative integers and zero, denoted by –1, –2, –3, . . . , and 0, respectively, arose to
permit solutions of equations such as x + b = a, where a and b are any natural numbers. This
leads to the operation of subtraction, or inverse of addition, and we write x = a – b. The set of
positive and negative integers and zero is called the set of integers.
2. Отрицательные числа и ноль, обозначаемые -1, -2, -3,..., , и 0, соответственно,
возникли для обеспечения решения уравнений, таких как x + b = a, где а и b – любые
натуральные числа. Это приводит к операции вычитания или обращению сложения, и
мы пишем x = a – b. Множество положительных и отрицательных чисел и нуля,
называется множеством целых чисел.
.
negative
arise
permit
lead
subtraction
отрицательный
возникать
позволять
вести
вычитание
4

5. Rational numbers

3. Rational numbers or fractions such as 2/3 , 5/4, . . . arose to permit solutions of equations such as bx
= a for all integers a and b, where b _ 0. This leads to the operation of division, or inverse of
multiplication, and we write x = a/b or a ÷ b, where a is the numerator and b the denominator. The set of
integers is a subset of the rational numbers, since integers correspond to rational numbers where b = 1.
3. Рациональные числа или дроби, такие как 2/3 , 5/4, . . ., возникли, чтобы позволить решения
уравнений, таких как bx = a для всех целых чисел а и b, где b _ 0. Это приводит к операции
деления, или обращения умножения, и мы пишем x = a/b или a ÷ b, где a, где а – числитель и b –
знаменатель. Множество целых чисел есть подмножество рациональных чисел, так как целые
числа соответствуют рациональным числам, в которых b = 1.
.
rational
fractions
division
numerator
denominator
рациональный
дробь
деление
числитель
знаменатель
5

6. Irrational numbers

and π are numbers which are not rational; i.e.,
they cannot be expressed as a/b (called the quotient of a and b), where a and b are
integers and b 0. The set of rational and irrational numbers is called the set of real
numbers.
4. Irrational numbers such as
2
4. Иррациональные числа, такие как
и π – числа, которые не являются
рациональными; т.е. они не могут быть выражены в виде a/ b (называемому
частным а и b), где а и b целые и b 0. Множество рациональных и
иррациональных чисел называется множеством действительных чисел
.
irrational
express
quotient
2
иррациональный
выражать
частное
6

7.

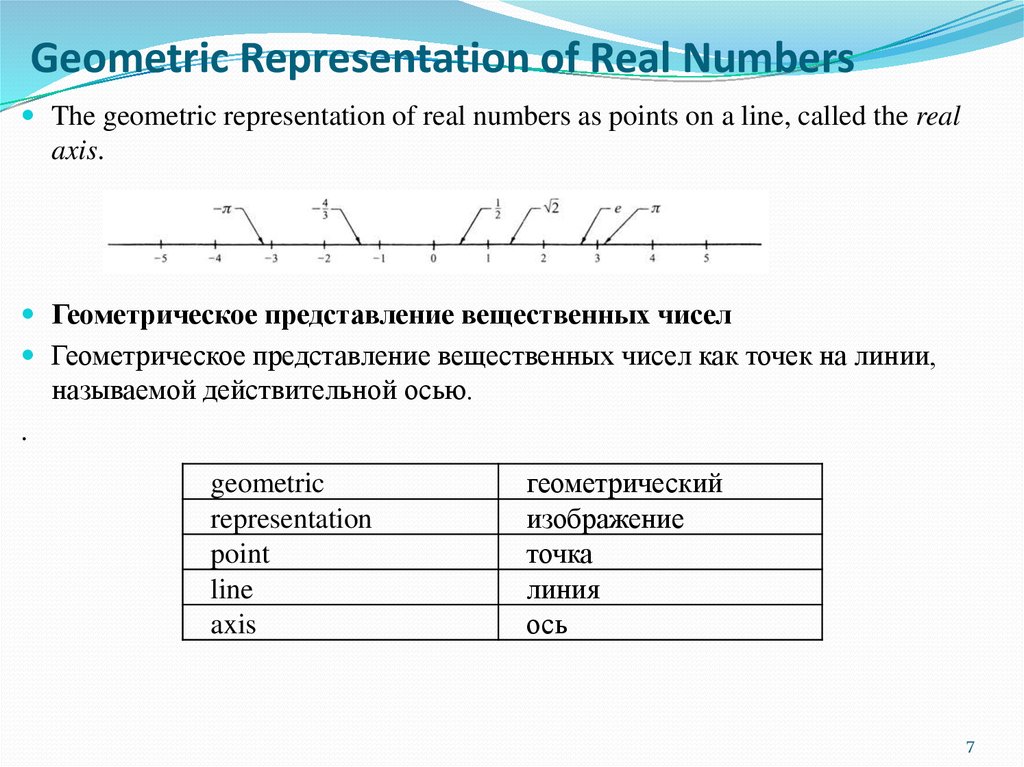
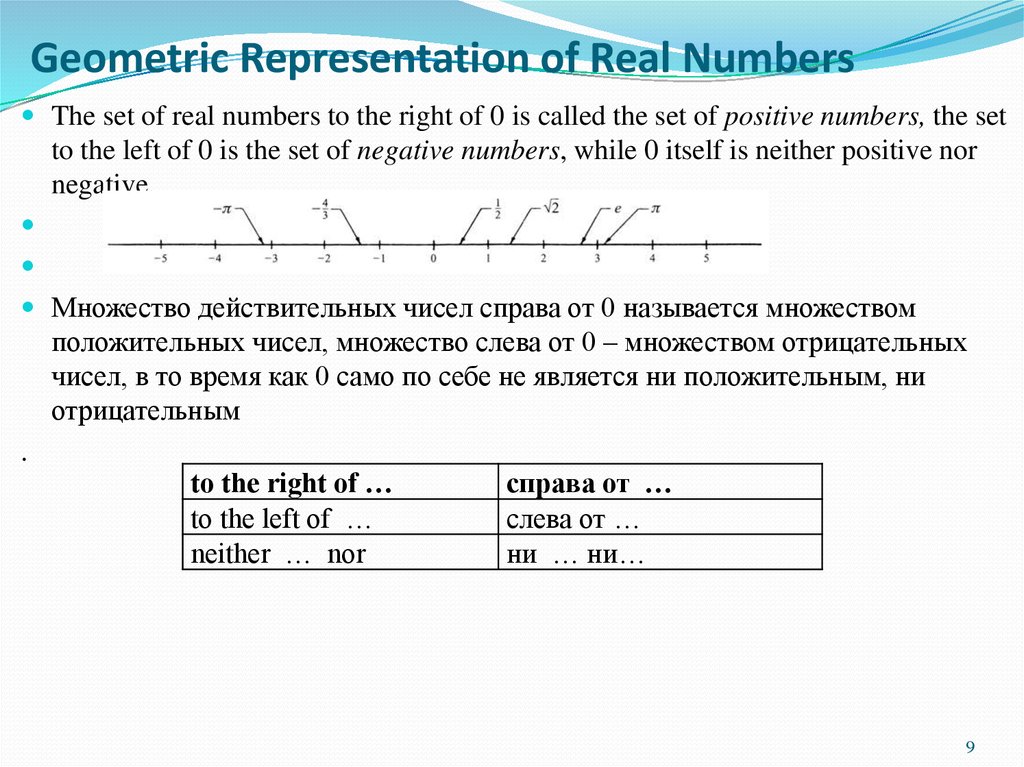
Geometric Representation of Real Numbers
The geometric representation of real numbers as points on a line, called the real
axis.
Геометрическое представление вещественных чисел
Геометрическое представление вещественных чисел как точек на линии,
называемой действительной осью.
.
geometric
representation
point
line
axis
геометрический
изображение
точка
линия
ось
7

8.

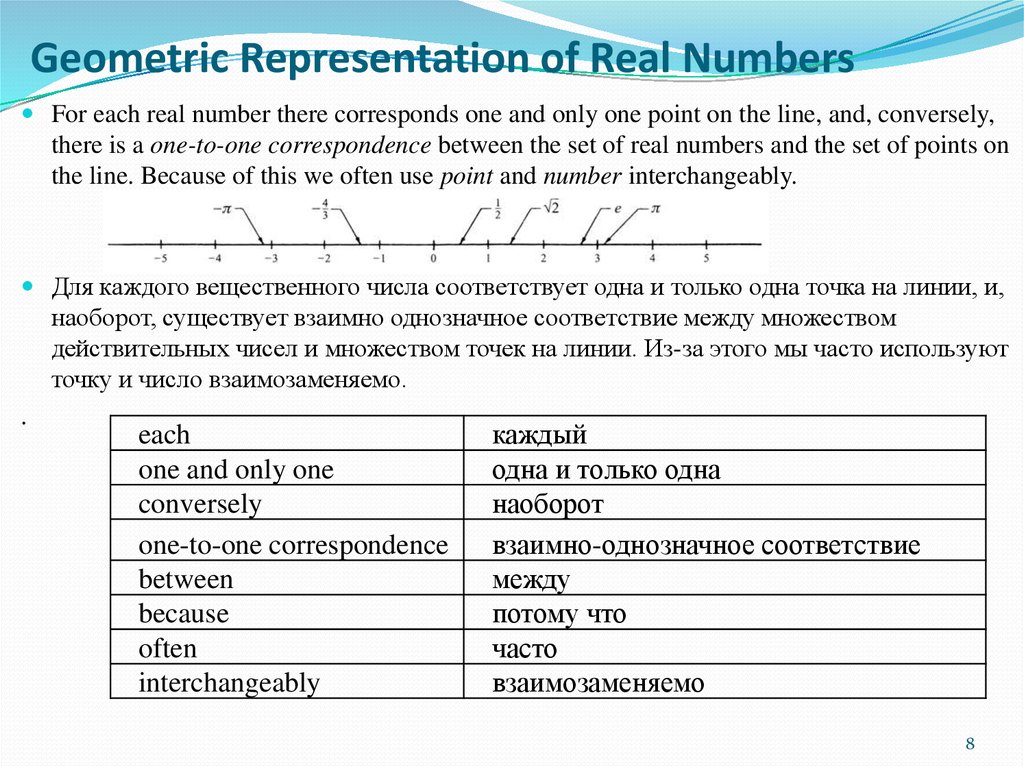
Geometric Representation of Real Numbers
For each real number there corresponds one and only one point on the line, and, conversely,
there is a one-to-one correspondence between the set of real numbers and the set of points on
the line. Because of this we often use point and number interchangeably.
Для каждого вещественного числа соответствует одна и только одна точка на линии, и,
наоборот, существует взаимно однозначное соответствие между множеством
действительных чисел и множеством точек на линии. Из-за этого мы часто используют
точку и число взаимозаменяемо.
.
each
one and only one
conversely
one-to-one correspondence
between
because
often
interchangeably
каждый
одна и только одна
наоборот
взаимно-однозначное соответствие
между
потому что
часто
взаимозаменяемо
8

9.

Geometric Representation of Real Numbers
The set of real numbers to the right of 0 is called the set of positive numbers, the set
to the left of 0 is the set of negative numbers, while 0 itself is neither positive nor
negative.
Множество действительных чисел справа от 0 называется множеством
положительных чисел, множество слева от 0 – множеством отрицательных
чисел, в то время как 0 само по себе не является ни положительным, ни
отрицательным
.
to the right of …
to the left of …
neither … nor
справа от …
слева от …
ни … ни…
9

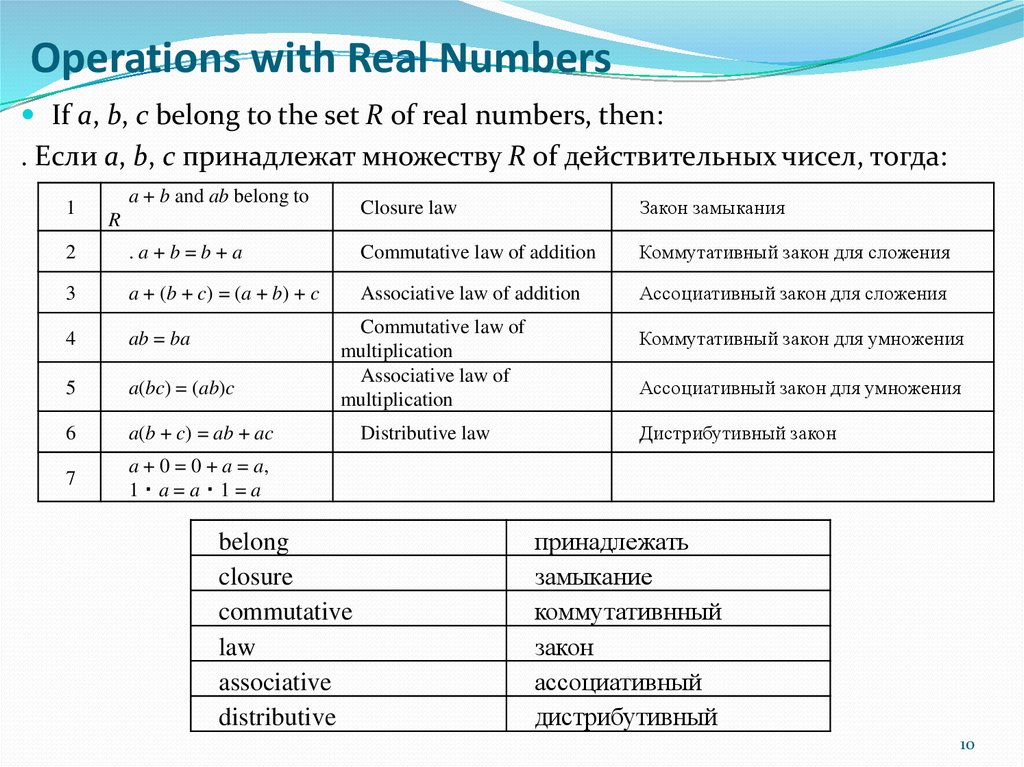
10. Operations with Real Numbers

If a, b, c belong to the set R of real numbers, then:
. Если a, b, c принадлежат множеству R of действительных чисел, тогда:
1
a + b and ab belong to
R
Closure law
Закон замыкания
2
.a+b=b+a
Commutative law of addition
Коммутативный закон для сложения
3
a + (b + c) = (a + b) + c
Associative law of addition
Ассоциативный закон для сложения
4
ab = ba
5
a(bc) = (ab)c
6
a(b + c) = ab + ac
7
a + 0 = 0 + a = a,
1・a = a・1 = a
Commutative law of
multiplication
Associative law of
multiplication
belong
closure
commutative
law
associative
distributive
Distributive law
Коммутативный закон для умножения
Ассоциативный закон для умножения
Дистрибутивный закон
принадлежать
замыкание
коммутативнный
закон
ассоциативный
дистрибутивный
10

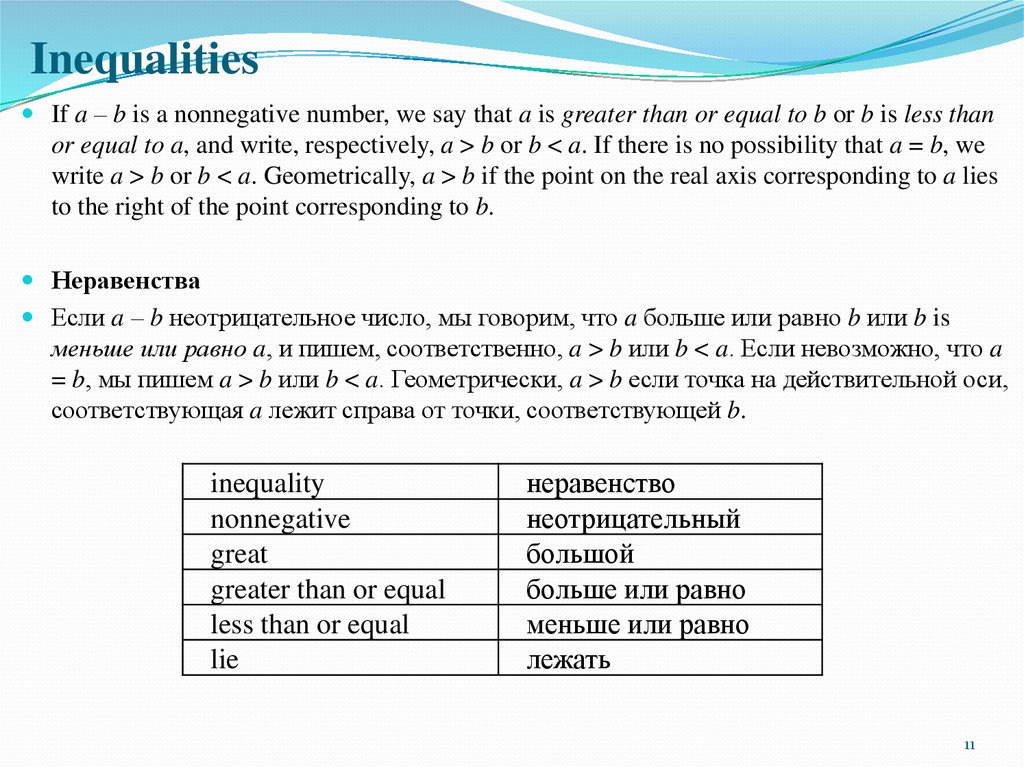
11. Inequalities

If a – b is a nonnegative number, we say that a is greater than or equal to b or b is less than
or equal to a, and write, respectively, a > b or b < a. If there is no possibility that a = b, we
write a > b or b < a. Geometrically, a > b if the point on the real axis corresponding to a lies
to the right of the point corresponding to b.
Неравенства
Если a – b неотрицательное число, мы говорим, что a больше или равно b или b is
меньше или равно a, и пишем, соответственно, a > b или b < a. Если невозможно, что a
= b, мы пишем a > b или b < a. Геометрически, a > b если точка на действительной оси,
соответствующая a лежит справа от точки, соответствующей b.
inequality
nonnegative
great
greater than or equal
less than or equal
lie
неравенство
неотрицательный
большой
больше или равно
меньше или равно
лежать
11

12. Absolute Value of Real Numbers

The absolute value of a real number a, denoted by |a|, is defined as a if a > 0, – a if a < 0, and
0 if a = 0.
The distance between any two points (real numbers) a and b on the real axis is |a–b| = |b–a|.
Абсолютное значение действительных чисел
Абсолютное значение действительного числа a, обозначаемое |a|, определяется как a
если a > 0, – a если a < 0, и 0 если a = 0.
Расстояние между двумя точками (действительными числами) a и b на действительной
оси есть |a–b| = |b–a|.
absolute
distance
абсолютный
расстояние
12

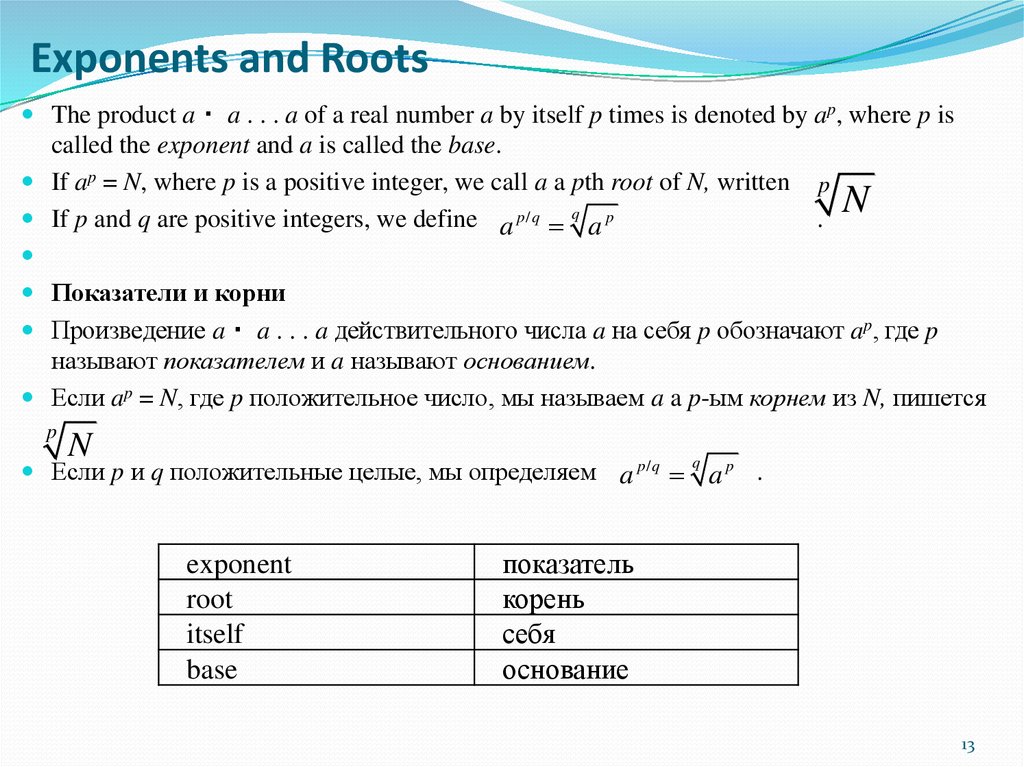
13. Exponents and Roots

The product a・ a . . . a of a real number a by itself p times is denoted by ap, where p is
called the exponent and a is called the base.
If ap = N, where p is a positive integer, we call a a pth root of N, written
p
If p and q are positive integers, we define a p / q
.
q
a
p
N
Показатели и корни
Произведение a・ a . . . a действительного числа a на себя p обозначают ap, где p
называют показателем и a называют основанием.
Если ap = N, где p положительное число, мы называем a a p-ым корнем из N, пишется
p
N
Если p и q положительные целые, мы определяем a p / q
exponent
root
itself
base
q
ap .
показатель
корень
себя
основание
13

14. Logarithms

If ap = N, p is called the logarithm of N to the base a, written p = loga N. If a and N
are positive and a 1, there is only one real value for p.
Логарифмы
Если ap = N, p называют логарифмом N по основанию a, пишется p = loga N.
Если a и N положительны и a 1, существует только одно действительное
значение для p.
.
logarithm
логарифм
14

15. Logarithms

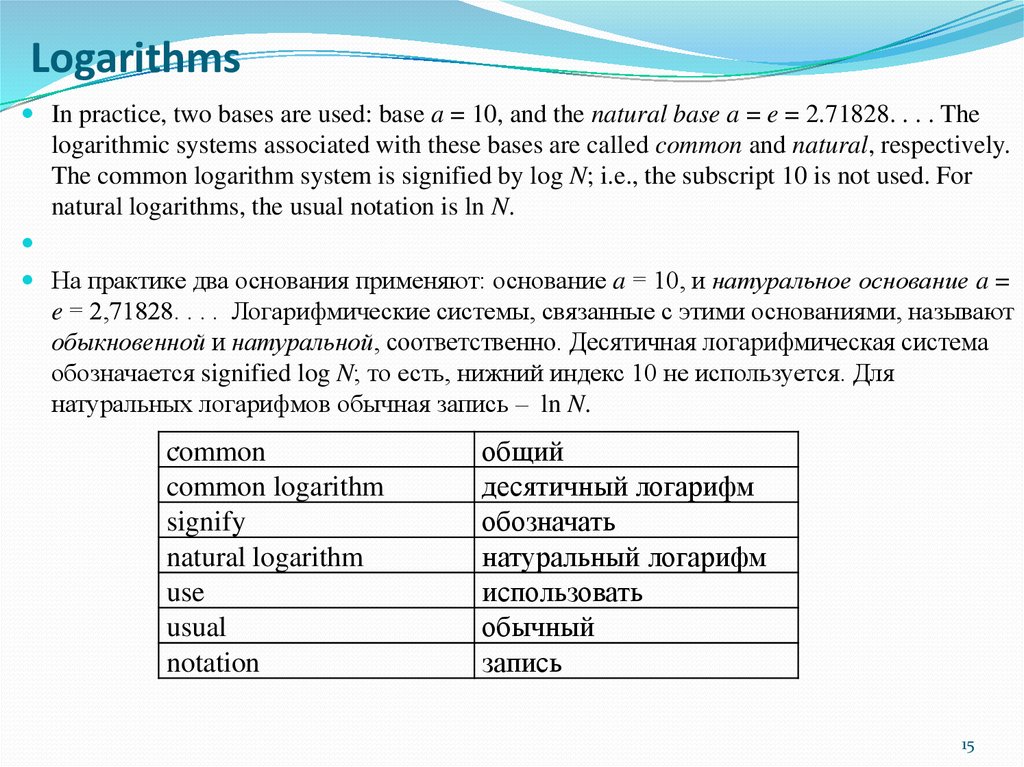
In practice, two bases are used: base a = 10, and the natural base a = e = 2.71828. . . . The
logarithmic systems associated with these bases are called common and natural, respectively.
The common logarithm system is signified by log N; i.e., the subscript 10 is not used. For
natural logarithms, the usual notation is ln N.
На практике два основания применяют: основание a = 10, и натуральное основание a =
e = 2,71828. . . . Логарифмические системы, связанные с этими основаниями, называют
обыкновенной и натуральной, соответственно. Десятичная логарифмическая система
обозначается signified log N; то есть, нижний индекс 10 не используется. Для
натуральных логарифмов обычная запись – ln N.
.
common
общий
common logarithm
signify
natural logarithm
use
usual
notation
десятичный логарифм
обозначать
натуральный логарифм
использовать
обычный
запись
15

16. Intervals

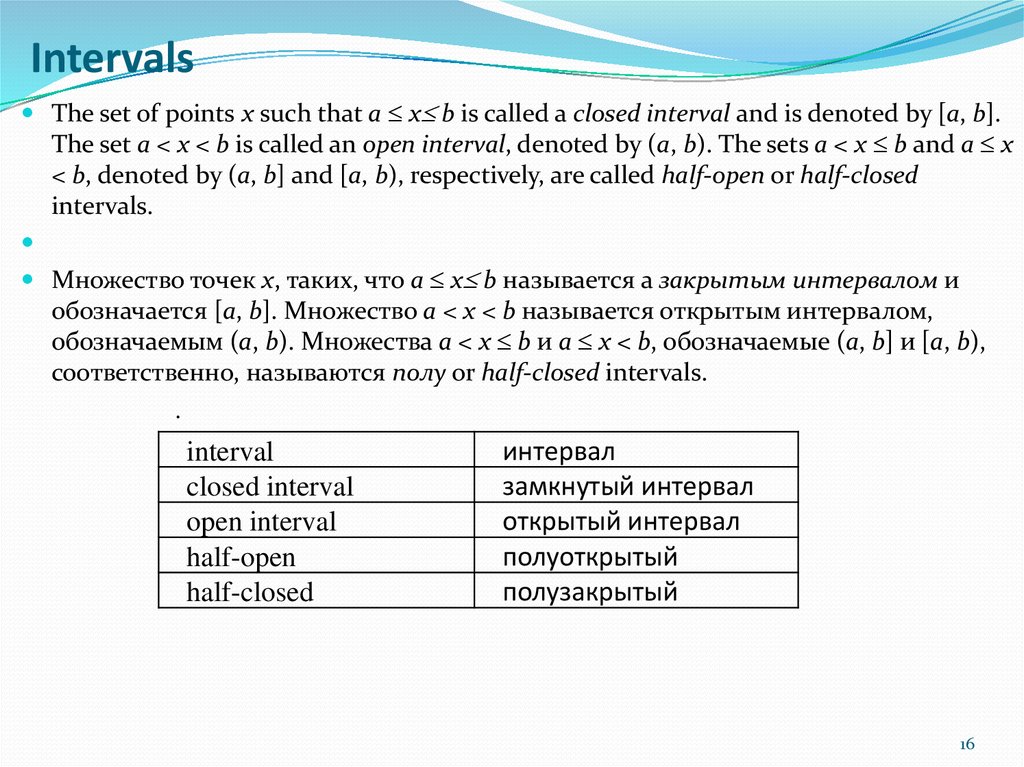
The set of points x such that a x b is called a closed interval and is denoted by [a, b].
The set a < x < b is called an open interval, denoted by (a, b). The sets a < x b and a x
< b, denoted by (a, b] and [a, b), respectively, are called half-open or half-closed
intervals.
Множество точек x, таких, что a x b называется a закрытым интервалом и
обозначается [a, b]. Множество a < x < b называется открытым интервалом,
обозначаемым (a, b). Множества a < x b и a x < b, обозначаемые (a, b] и [a, b),
соответственно, называются полу or half-closed intervals.
.
interval
closed interval
open interval
half-open
half-closed
интервал
замкнутый интервал
открытый интервал
полуоткрытый
полузакрытый
16

17. Intervals

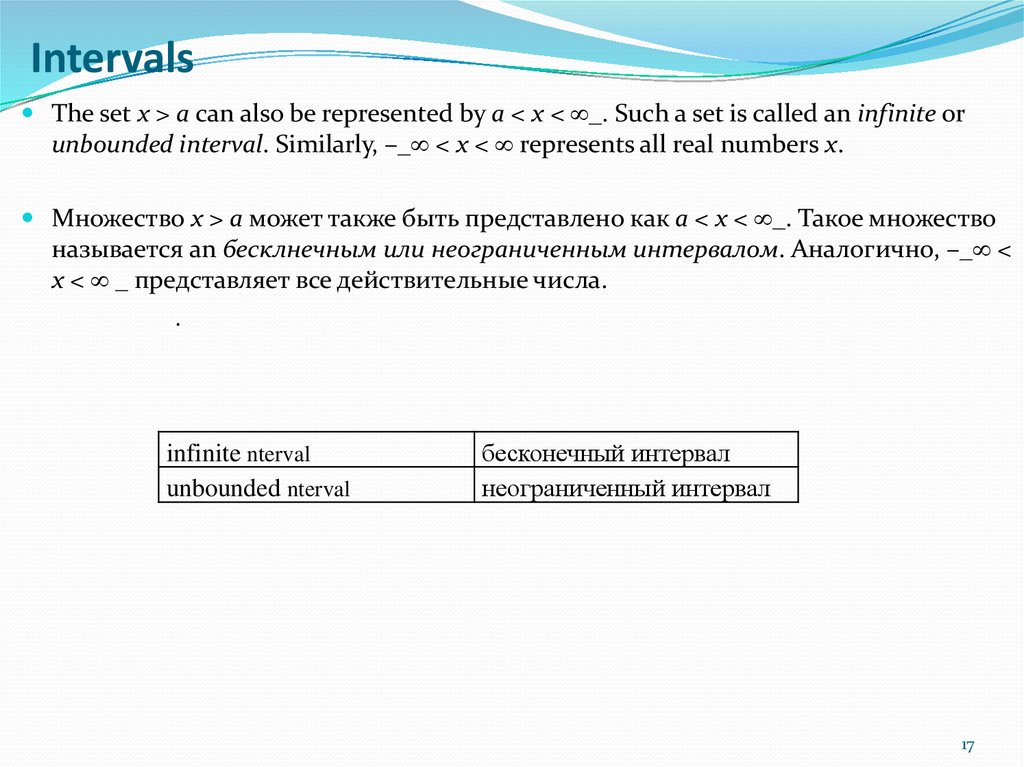
The set x > a can also be represented by a < x < _. Such a set is called an infinite or
unbounded interval. Similarly, –_ < x < represents all real numbers x.
Множество x > a может также быть представлено как a < x < _. Такое множество
называется an бесклнечным или неограниченным интервалом. Аналогично, –_ <
x < _ представляет все действительные числа.
.
infinite nterval
unbounded nterval
бесконечный интервал
неограниченный интервал
17

18. Neighborhoods

The set of all points x such that |x – a|< δ, where δ > 0, is called a δ neighborhood of the
point a. The set of all points x such that 0 < |x – a| < δ, in which x = a is excluded, is
called a deleted δ neighborhood of a or an open ball of radius δ about a.
Множество всех точек x таких, что |x – a|< δ, где δ > 0, называется δ окрестностью
точки a. Множество всех точек x, таких что 0 < |x – a| < δ, в котором x = a
исключено, называетсяa проколотой δ окрестностью a или открытым шаром
радиуса δ около a.
.
neighborhood
deleted δ neighborhood
ball
radius
about
окрестность
проколотая δ окрестность
шар
радиус
около
18

19. Bounds

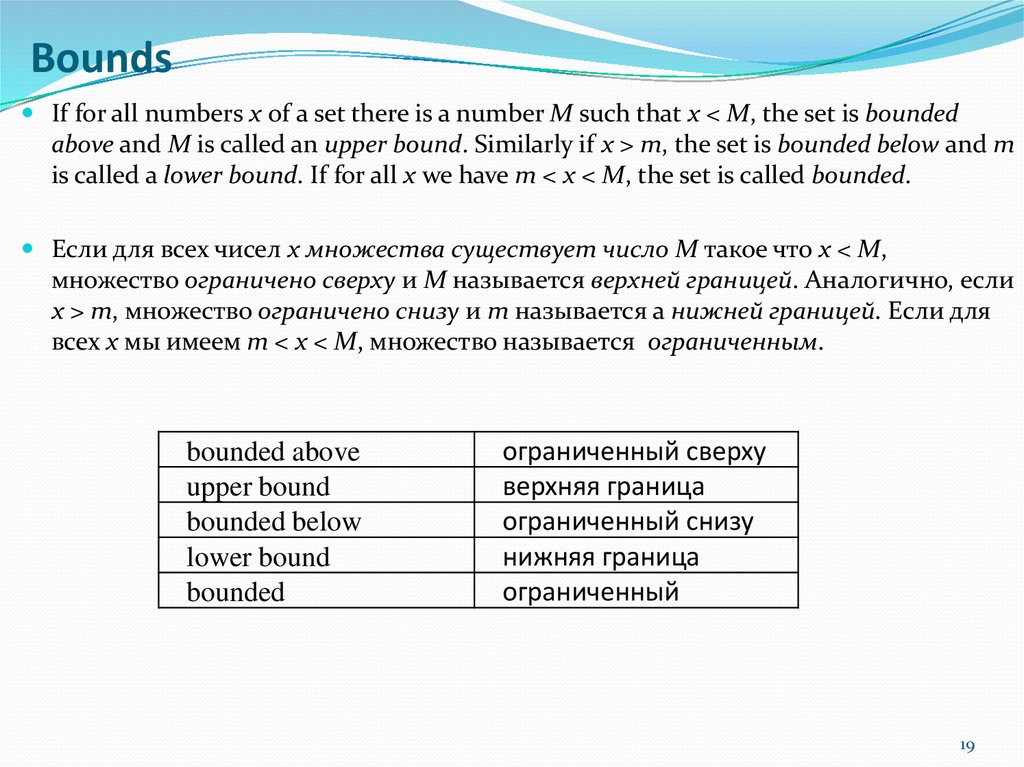
If for all numbers x of a set there is a number M such that x < M, the set is bounded
above and M is called an upper bound. Similarly if x > m, the set is bounded below and m
is called a lower bound. If for all x we have m < x < M, the set is called bounded.
Если для всех чисел x множества существует число M такое что x < M,
множество ограничено сверху и M называется верхней границей. Аналогично, если
x > m, множество ограничено снизу и m называется a нижней границей. Если для
всех x мы имеем m < x < M, множество называется ограниченным.
bounded above
upper bound
bounded below
lower bound
bounded
ограниченный сверху
верхняя граница
ограниченный снизу
нижняя граница
ограниченный
19

20. Algebraic and Transcendental Numbers

A number x which is a solution to the polynomial equation
a0xn + a1xn–1 + a2xn–2 + . . . + an–1x + an = 0
where a0 0, a1, a2, . . . , an are integers and n is a positive integer, called the degree of
polynomial, is called an algebraic number. A number which cannot be expressed as a
solution of any polynomial equation with integer coefficients is called a transcendental
number.
Число x которое является решением степенного уравнения (…)
где a0 0, a1, a2, . . . , an целые и n положительное целое, называемое степенью
многочлена, называется алгебраическим числом. Числа, которые не могут быть
выражены как решение какого-нибудь степенного уравнения с целыми
коэффициентами, называются трансцедентными числами.
.
polynom
degree
algebraic number
transcendental number
многочлен
степень
алгебраичечкое число
трансцендентное число
20

21. The Complex Number System

We can consider a complex number as an object having the form a + bi, where
a and b are real numbers called the real and imaginary parts, and i = is called
the imaginary unit.
Мы рассматриваем комплексное число как объект, имеющий форму a +
bi, где a и b действительные числа, называемые действительной и
мнимой частями, и i = называется мнимой единицей. .
complex number
real part
imaginary part
imaginary unit
комплексное число
действительная часть
мнимая часть
мнимая единица
21

22. The Complex Number System

The absolute value or modulus of a + bi is defined as |a + bi| = a 2 b 2 . The
complex conjugate of a + bi is defined as a – bi. The complex conjugate of the
complex number z is often indicated by or z*.
Абсолютное значение или модуль a + bi определяется как |a + bi| =
a 2 .b 2
Комплексно сопряженное к a + bi определяется как a – bi. Комплексно
сопряженное комплексного числа z часто обозначается как or z*.
absolute value
modulus
conjugate
complex conjugate
абсолютная величина
модуль
сопряженный
комплексно
сопряженный
22

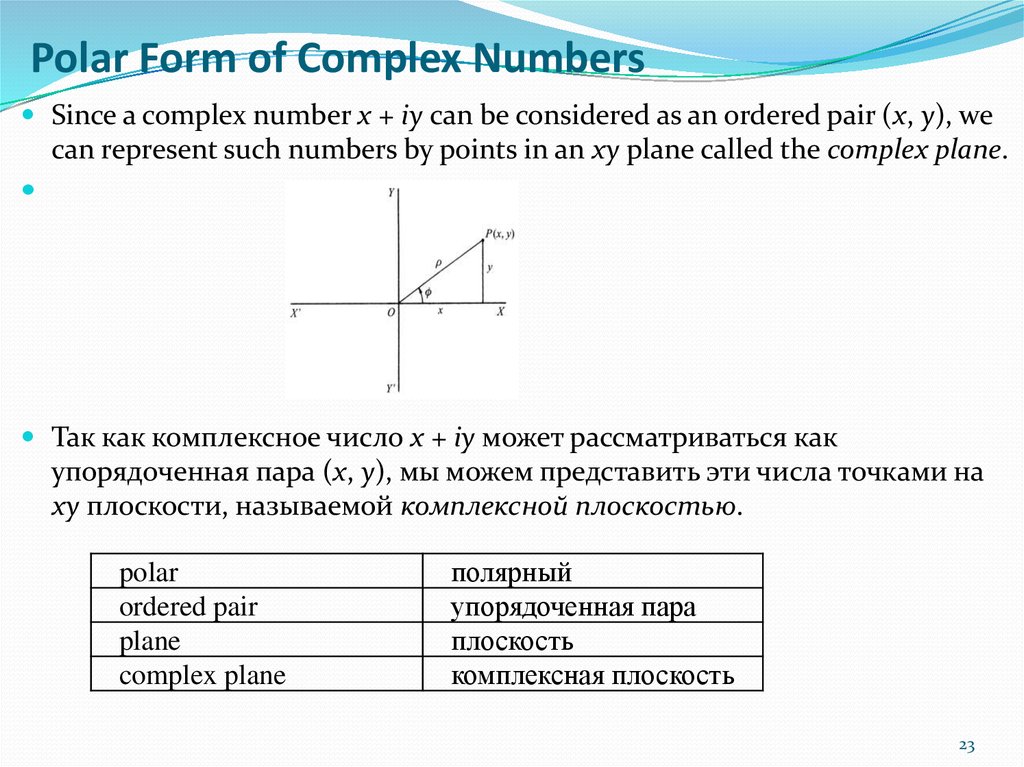
23. Polar Form of Complex Numbers

Since a complex number x + iy can be considered as an ordered pair (x, y), we
can represent such numbers by points in an xy plane called the complex plane.
Так как комплексное число x + iy может рассматриваться как
упорядоченная пара (x, y), мы можем представить эти числа точками на
xy плоскости, называемой комплексной плоскостью.
polar
ordered pair
plane
complex plane
полярный
упорядоченная пара
плоскость
комплексная плоскость
23

24. Polar Form of Complex Numbers

We see that x = ρ cos φ, y = ρ sin φ, where x 2 y 2 =| x + iy| and φ, called the
amplitude and argument, is the angle which line OP makes with the positive x
axis OX. It follows that z = x + iy = ρ(cos φ + i sin φ) called the polar form of
the complex number, where ρ and φ are called polar coordinates. It is
sometimes convenient to write cis φ instead of cos φ + i sin φ.
Мы видим, что x = ρ cos φ, y = ρ sin φ, где
= |x + iy| и φ,
называемые амплитудой и аргументом, – угол, который линия OP
делает с положительным x осиOX. Отсюда следует, что z = x + iy = ρ(cos
φ + i sin φ), называемое полярной формой комплексного числа, где ρ и φ
называются полярными координатами. Иногда удобно писать cis φ
вместо cos φ + i sin φ.
amplitude
argument
angle
polar form
polar coordinates
convenient
x2 y 2
амплитуда
аргумент
угол
полярная форма
полярные координаты
удобный
24

25. Polar Form of Complex Numbers

If z1 = x1 + iy1 = ρ1 (cos φ1 + i sin φ1) and z2 = x2 + iy2 = ρ2(cosφ2 + i sin φ2) and by using
the addition formulas for sine and cosine, we can show that
z1z2 = ρ1ρ2{cos(φ1 + φ2) + i sin(φ1 + φ2)}
z1 1
cos 1 2 i sin 1 2
z2 2
z n n cos n i sin n
2 k
z cos
n
1
n
1
n
2 k
i
sin
n
Если z1 = x1 + iy1 = ρ1 (cos φ1 + i sin φ1) и z2 = x2 + iy2 = ρ2(cosφ2 + i sin φ2), используя
формулу сложения для синуса и косинуса, мы можем показать, что (…)
sine
cosine
синус
косинус
25

26. Function

A function is composed of a domain set, a range set, and a
rule of correspondence that assigns exactly one element of
the range to each element of the domain.
Функция состоит из области определения, области
значений и правила соответствия, которое назначает
точно один элемент из области значений каждому
элементу из области определения.
domain set
range set
rule
область определения
область значений
правило
26

27. Monotonic Functions

A function is called monotonic increasing in an interval if for
any two points x1 and x2 in the interval, (x1 < x2),f x1 f x2 . If
f x1 f x2 whenever x < x , then f(x) the function is called
1
2
strictly increasing.
Функция называется монотонно неубывающей на
интервале, если для любых двух точек x1 и x2 на
интервале,( x1 < x2), f x1 f x2 . Еслиf x1 f x2 функция
называется монотонно возрастающей.
monotonic increasing
strictly increasing
монотонно неубывающая
монотонно возрастающая
27

28. Monotonic Functions

A function is called monotonic decreasing in an interval if
for any two points x1 and x2 in the interval, (x1 < x2), f x1 f x2
If f x1 f x2 whenever x1 < x2, then f(x) the function is
called
strictly вуcreasing.
Функция называется монотонно невозрастающей на
интервале, если для любых двух точек x1 и x2 на
интервале,( x1 < x2), f x1 f x2 . Если f x1 f x2 функция
называется монотонно возрастающей.
monotonic decreasing
strictly decreasing
монотонно невозрастающая
монотонно убывающая
28

29. Inverse function

Let the correspondence y=f(x) between the domain and range
values be one-to-one. Then a new function f –1, called the
inverse function of f, can be created by interchanging the
domain and range of f. This information is contained in the
form x = f –1(y).
Пусть соответствие y=f(x) между областью определения и
областью значений взаимно-однозначно. Тогда новая функция f –1,
называемая обратной функцией f, может быть образована
взаимозаменой областью определения и области значений f. Эта
информация содержится в форме x = f –1(y).
inverse function
обратная функция
29

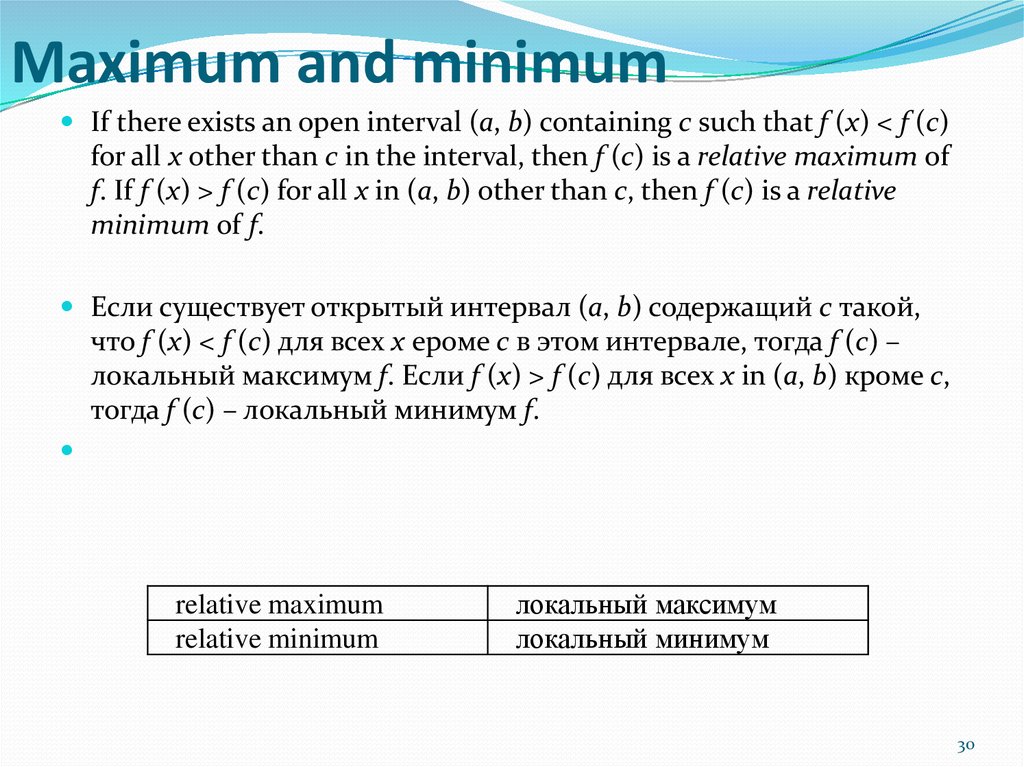
30. Maximum and minimum

If there exists an open interval (a, b) containing c such that f (x) < f (c)
for all x other than c in the interval, then f (c) is a relative maximum of
f. If f (x) > f (c) for all x in (a, b) other than c, then f (c) is a relative
minimum of f.
Если существует открытый интервал (a, b) содержащий c такой,
что f (x) < f (c) для всех x ероме c в этом интервале, тогда f (c) –
локальный максимум f. Если f (x) > f (c) для всех x in (a, b) кроме c,
тогда f (c) – локальный минимум f.
relative maximum
relative minimum
локальный максимум
локальный минимум
30

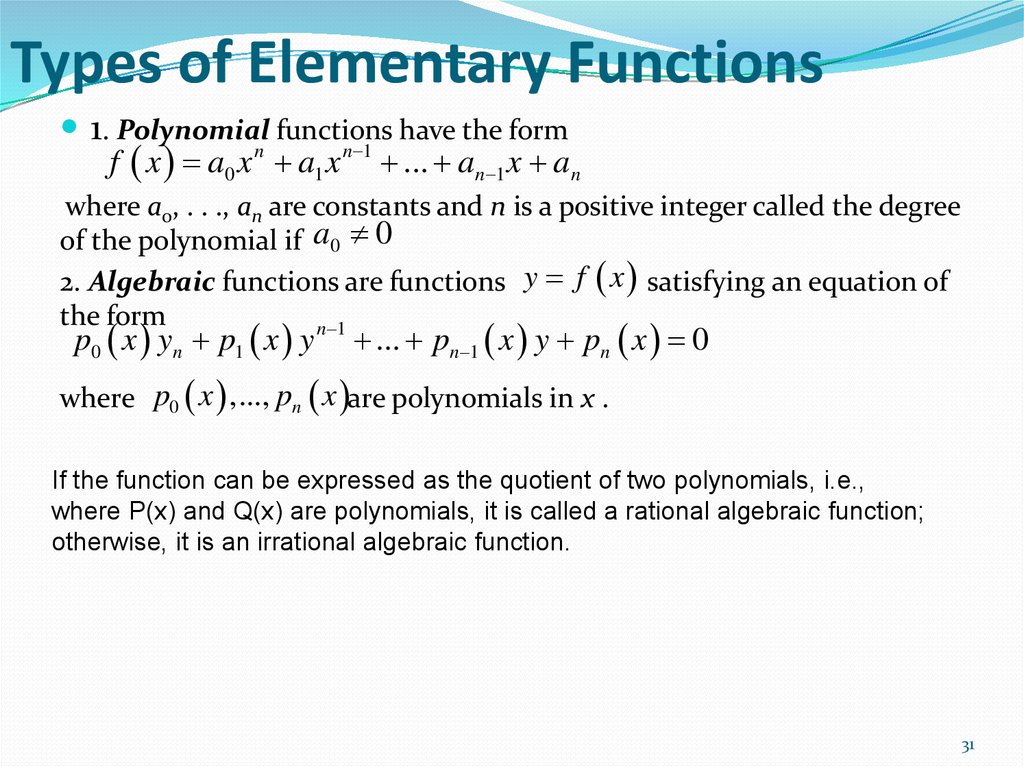
31. Types of Elementary Functions

1. Polynomial functions have the form
f x a0 x n a1 x n 1 ... an 1 x an
where a0, . . ., an are constants and n is a positive integer called the degree
of the polynomial if a0 0
2. Algebraic functions are functions y f x satisfying an equation of
the form
n 1
p0 x yn p1 x y
... pn 1 x y pn x 0
where p0 x ,..., pn x are polynomials in x .
If the function can be expressed as the quotient of two polynomials, i.e.,
where P(x) and Q(x) are polynomials, it is called a rational algebraic function;
otherwise, it is an irrational algebraic function.
31

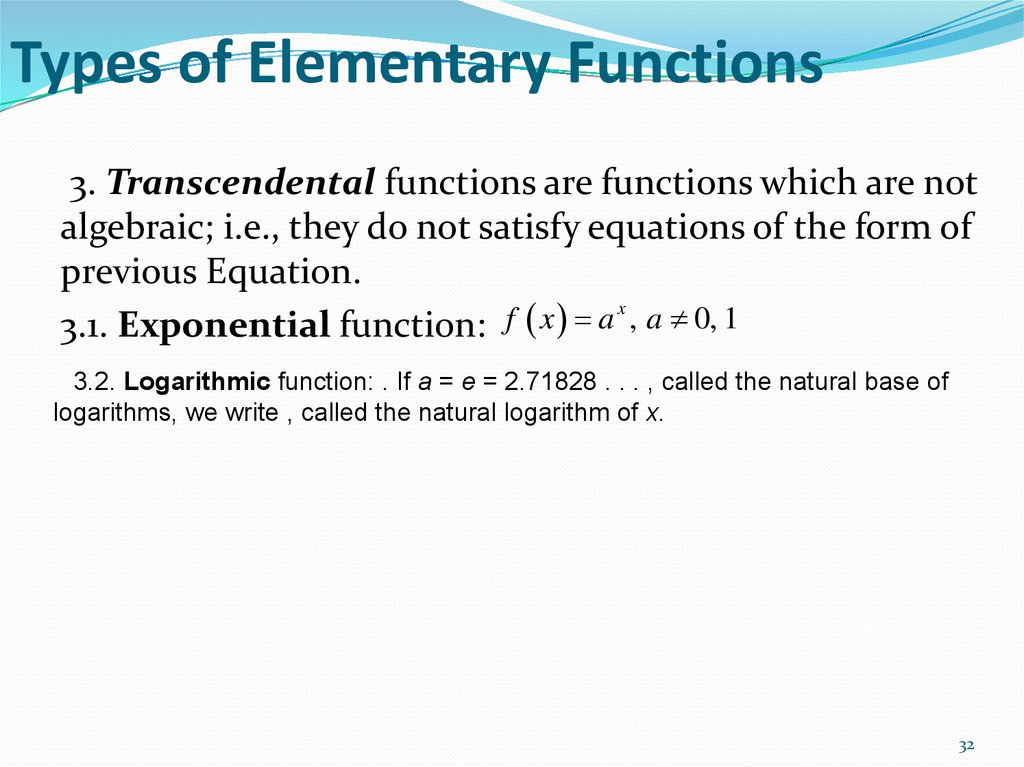
32. Types of Elementary Functions

3. Transcendental functions are functions which are not
algebraic; i.e., they do not satisfy equations of the form of
previous Equation.
x
f
x
a
, a 0, 1
3.1. Exponential function:
3.2. Logarithmic function: . If a = e = 2.71828 . . . , called the natural base of
logarithms, we write , called the natural logarithm of x.
32

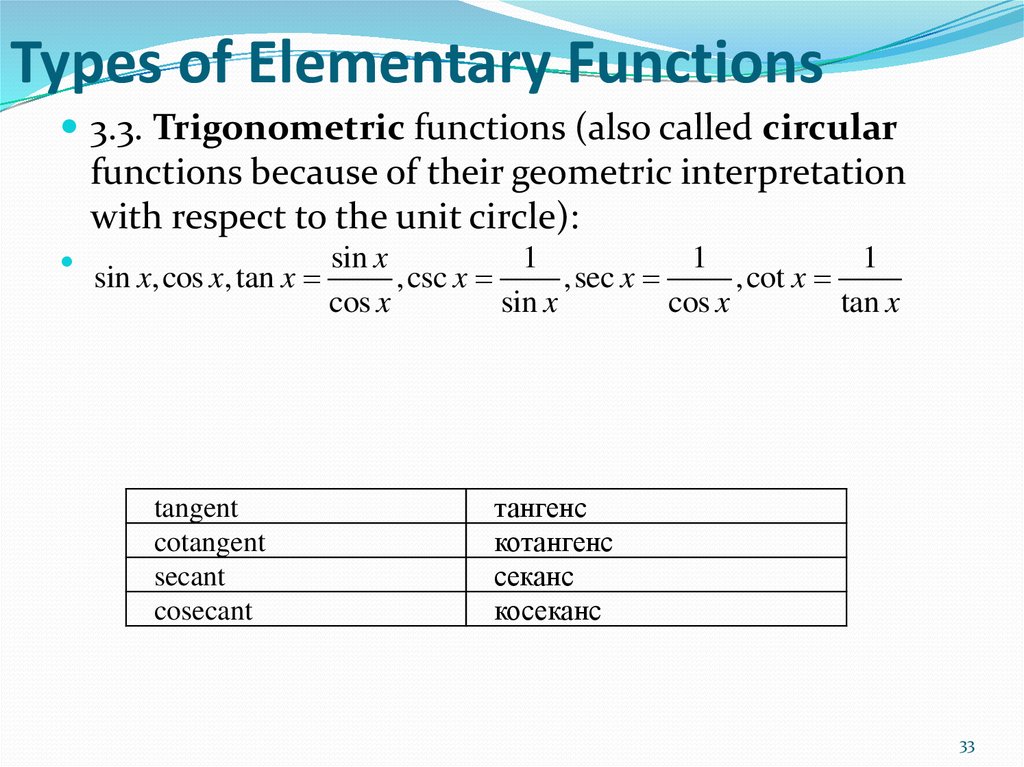
33. Types of Elementary Functions

3.3. Trigonometric functions (also called circular
functions because of their geometric interpretation
with respect to the unit circle):
sin x,cos x, tan x
tangent
cotangent
secant
cosecant
sin x
1
1
1
,csc x
,sec x
,cot x
cos x
sin x
cos x
tan x
тангенс
котангенс
секанс
косеканс
33

34. Types of Elementary Functions

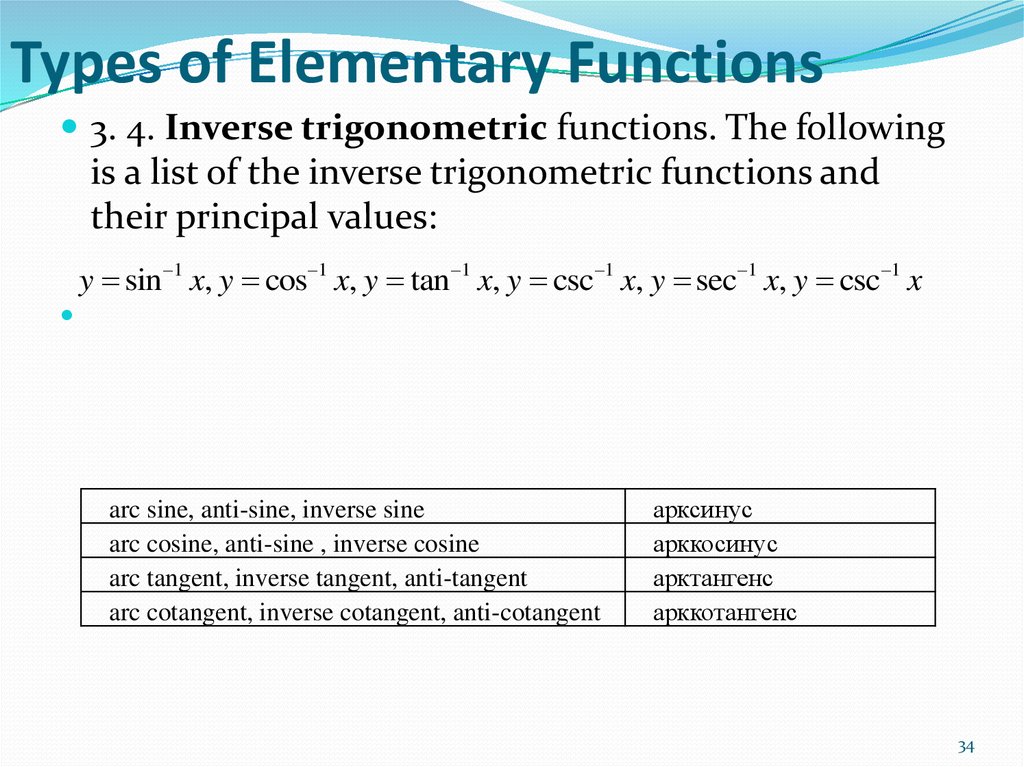
3. 4. Inverse trigonometric functions. The following
is a list of the inverse trigonometric functions and
their principal values:
y sin 1 x, y cos 1 x, y tan 1 x, y csc 1 x, y sec 1 x, y csc 1 x
arc sine, anti-sine, inverse sine
arc cosine, anti-sine , inverse cosine
arc tangent, inverse tangent, anti-tangent
arc cotangent, inverse cotangent, anti-cotangent
арксинус
арккосинус
арктангенс
арккотангенс
34

35. Types of Elementary Functions

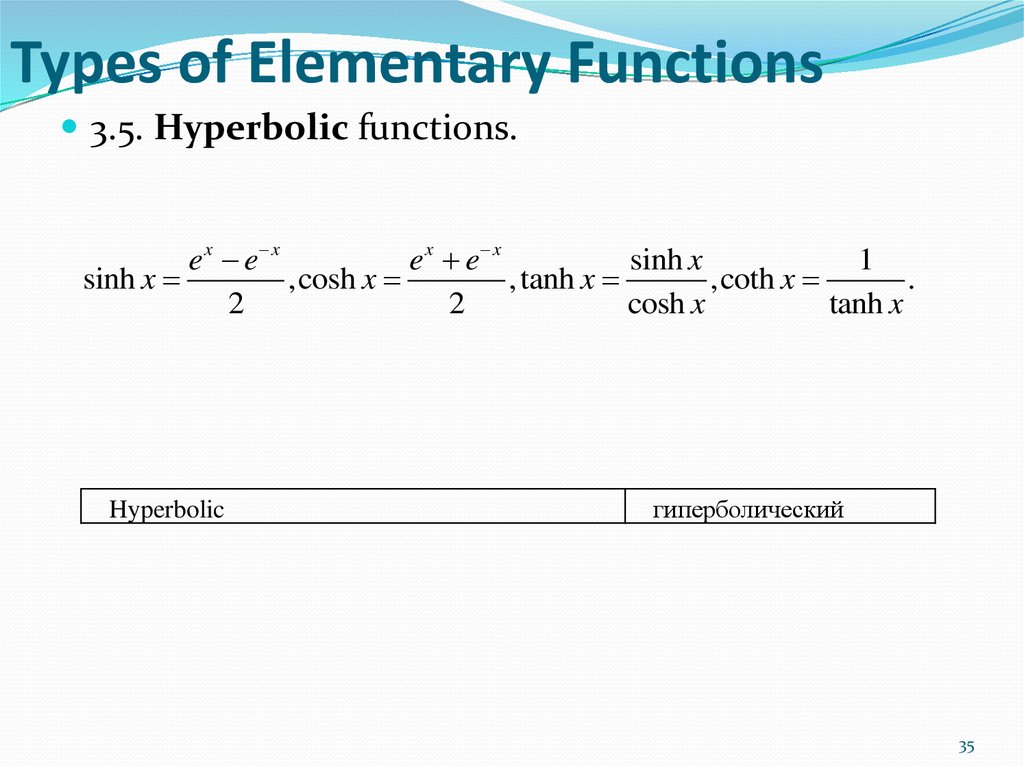
3.5. Hyperbolic functions.
e x e x
e x e x
sinh x
1
sinh x
,cosh x
, tanh x
,coth x
.
2
2
cosh x
tanh x
Hyperbolic
гиперболический
35

36. Limit of Functions

Let f (x) be defined and single-valued for all values of x near x = x0 with
the possible exception of x = x0 itself (i.e., in a deleted δ neighborhood of x0).
We say that the number l is the limit of f(x) as x approaches x0 and write
lim f x l if for any positive number (however small) we can find some
x x0
positive number δ (usually depending on ) such that f x l whenever
x x0 . In such a case we also say that f(x) approaches l as x approaches x0 and
write f (x) → l as x → x0.
Пусть f (x) определена и однозначна для всех значений x около x = x0 с возможным
исключением x = x0 самой (т.е., в проколотой δ окрестности x0). Мы говорим, что
число l –это предел f(x), когда x стремится к x0 и пишем lim f x l если для
x x0
любого положительного числа (как угодно малого) мы можем найти такое
положительное число δ (обычно зависящего от ) такое, что f x l когда
x x0 . . В этом случае, мы также говорим, что f(x) стремится к l при x
стремящимся к x0 и пишем f (x) → l as x → x0.
36

37. Derivatives

The derivative of the function f at its domain value x0 is a limit
f x0 h f x0
f ' x lim
h 0
h
If this limit can be formed at each point of a subdomain of the domain of
f, then f is said to be differentiable on that subdomain and a new
function f′ has been constructed.
Производная функции f в точке ее области определения x0 это
предел …..
Если этот предел может быть образован в каждой точке
подмножества области определения f, тогда f , говорят,
дифференцируема на этом подмножестве и новая функция f′
построена.
derivative
производная
subdomain
подобласть
differentiable
дифференцируемый
construct
строить
37

38. Derivatives

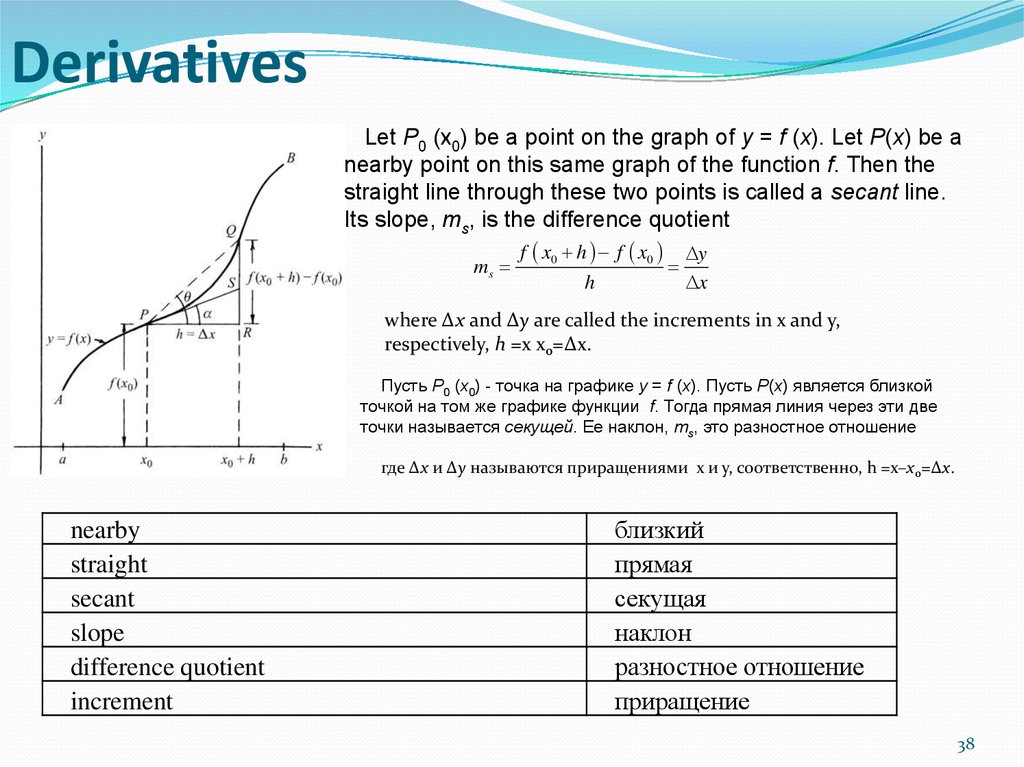
Let P0 (x0) be a point on the graph of y = f (x). Let P(x) be a
nearby point on this same graph of the function f. Then the
straight line through these two points is called a secant line.
Its slope, ms, is the difference quotient
ms
f x0 h f x0
h
y
x
where Δx and Δy are called the increments in x and y,
respectively, h =x x0=Δx.
Пусть P0 (x0) - точка на графике y = f (x). Пусть P(x) является близкой
точкой на том же графике функции f. Тогда прямая линия через эти две
точки называется секущей. Ее наклон, ms, это разностное отношение
где Δx и Δy называются приращениями x и y, соответственно, h =x–x0=Δx.
nearby
straight
secant
slope
difference quotient
increment
близкий
прямая
секущая
наклон
разностное отношение
приращение
38

39. Differentials

Let Δx = dx be an increment given to x. Then Δy = f (x + Δx) – f (x) is called
the increment in y = f (x). If f (x) is continuous and has a continuous first
derivative in an interval, then Δy = f ′(x) Δx + Δx where → 0 as Δx → 0.
The expression dy = f ′(x)dx is called the differential of y or f (x) or the
principal part of Δy.
Пусть Δx = dx приращение, данное x. Тогда Δy = f (x + Δx) – f (x)
называется приращением y = f (x). если f (x) непрерывна и имеет
непрерывную первую производную на интервале, тогда Δy = f ′(x) Δx +
Δx где _ → 0 при Δx → 0. Выражение dy = f ′(x)dx называется
дифференциалом y или f (x) или главной частью Δy.
differential
continuous
expression
principal part
дифференциал
непрерывный
выражение
главная часть
39

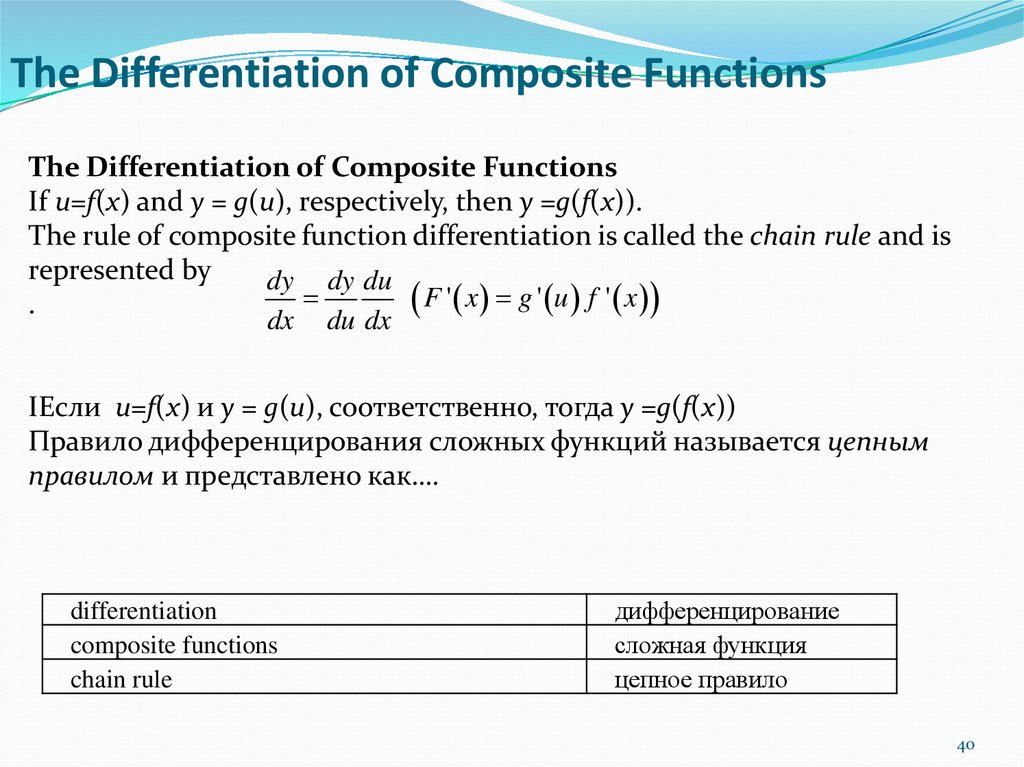
40. The Differentiation of Composite Functions

The Differentiation of Composite Functions
If u=f(x) and y = g(u), respectively, then y =g(f(x)).
The rule of composite function differentiation is called the chain rule and is
represented by
dy dy du
F ' x g ' u f ' x
.
dx du dx
IЕсли u=f(x) и y = g(u), соответственно, тогда y =g(f(x))
Правило дифференцирования сложных функций называется цепным
правилом и представлено как….
differentiation
composite functions
chain rule
дифференцирование
сложная функция
цепное правило
40

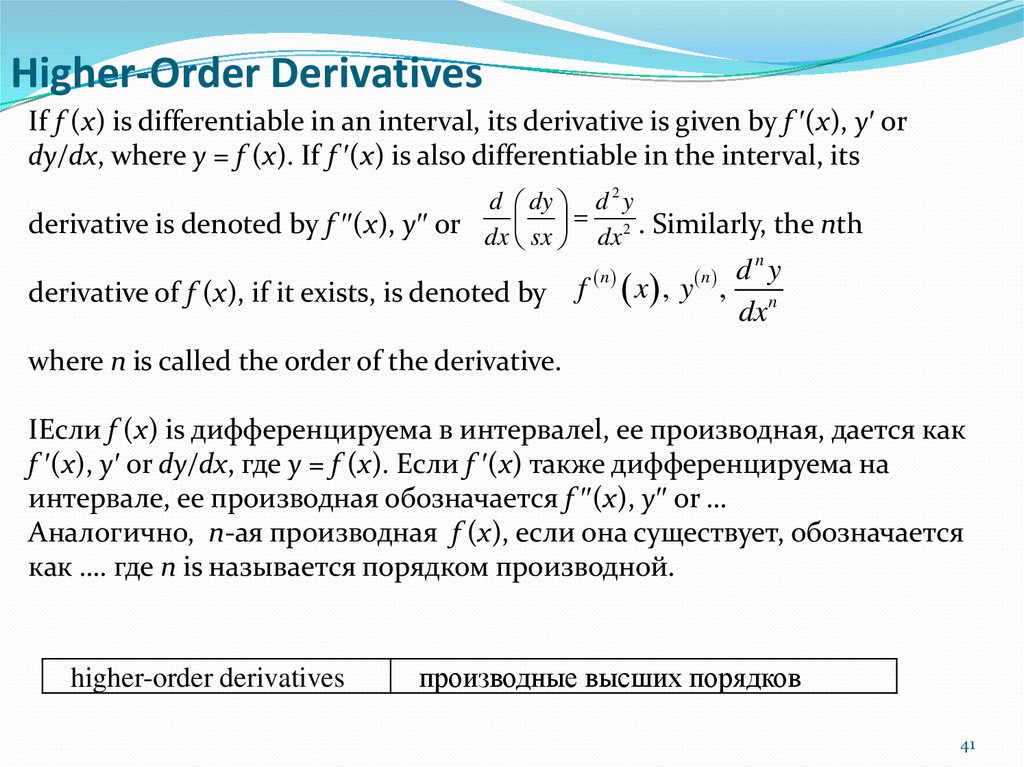
41. Higher-Order Derivatives

If f (x) is differentiable in an interval, its derivative is given by f ′(x), y′ or
dy/dx, where y = f (x). If f ′(x) is also differentiable in the interval, its
d dy d 2 y
derivative is denoted by f ″(x), y″ or dx sx dx 2 . Similarly, the nth
derivative of f (x), if it exists, is denoted by
f
n
dny
x , y , n
dx
n
where n is called the order of the derivative.
IЕсли f (x) is дифференцируема в интервалеl, ее производная, дается как
f ′(x), y′ or dy/dx, где y = f (x). Если f ′(x) также дифференцируема на
интервале, ее производная обозначается f ″(x), y″ or …
Аналогично, n-ая производная f (x), если она существует, обозначается
как …. где n is называется порядком производной.
higher-order derivatives
производные высших порядков
41