Similar presentations:
Mathematics for еconomists. (Week 1-12)
1.
2. Mathematics for Economists II Week #12
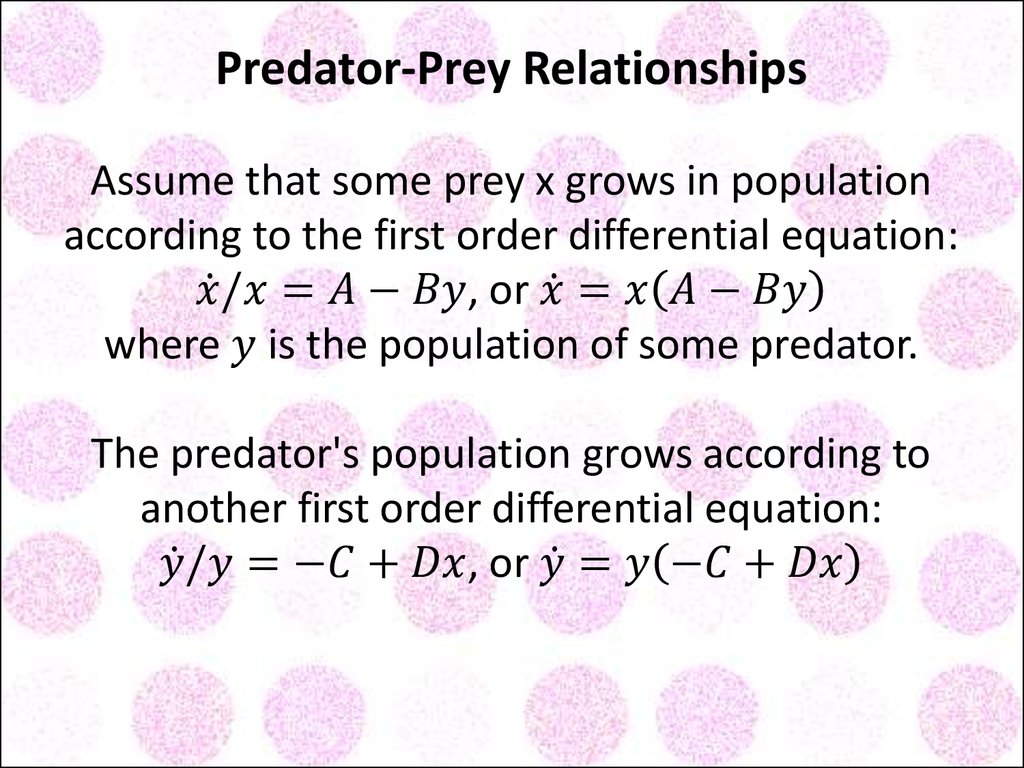
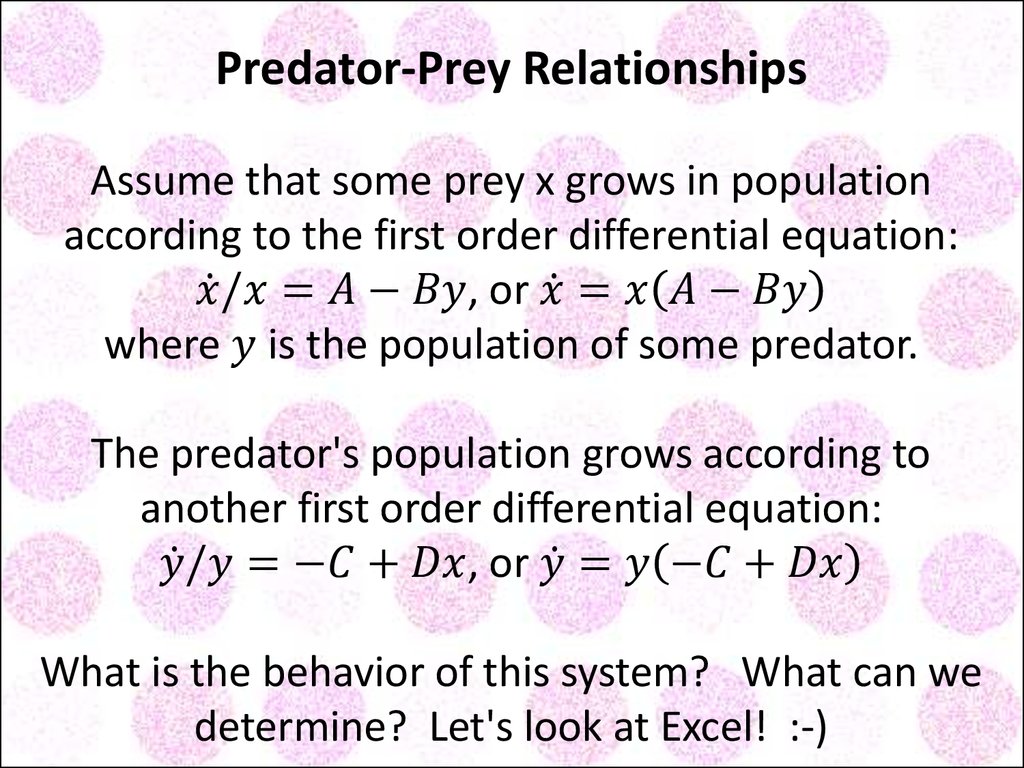
ReviewPredator-prey relationships
Solving simultaneous first order differential equations
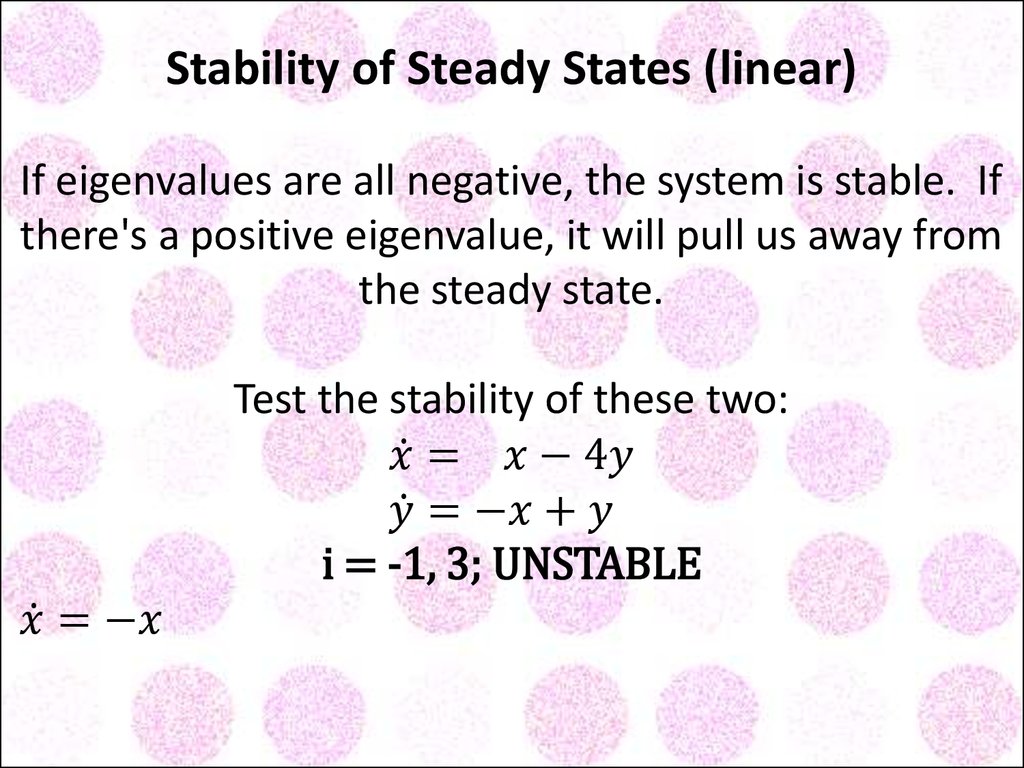
Steady states and their stability
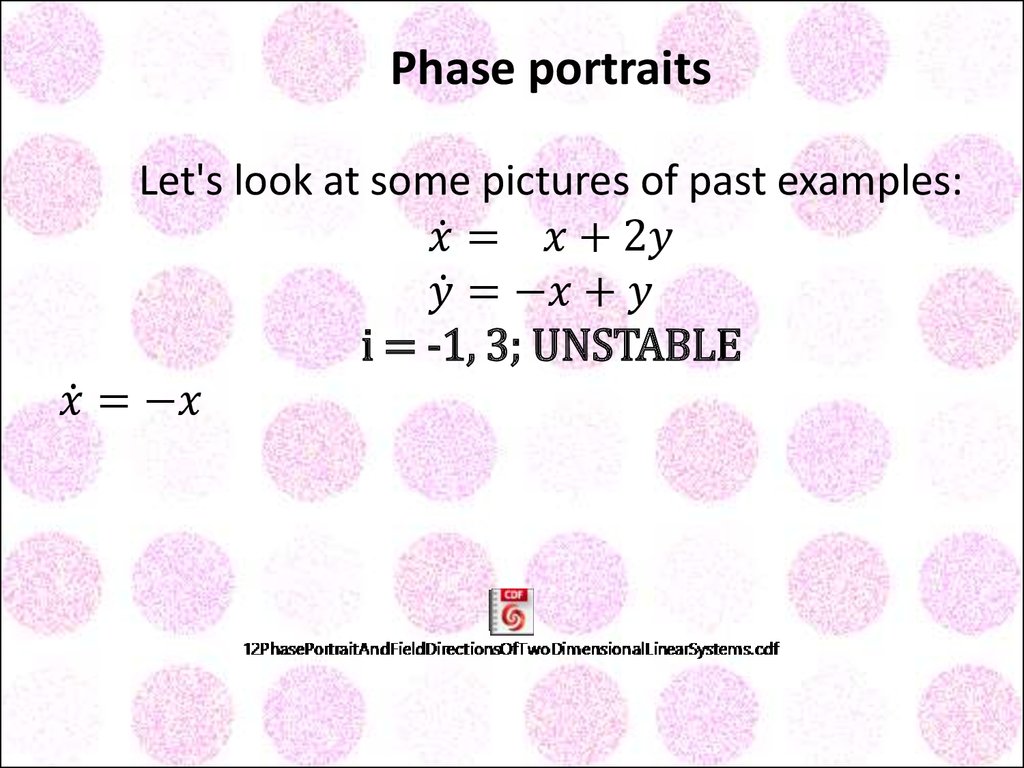
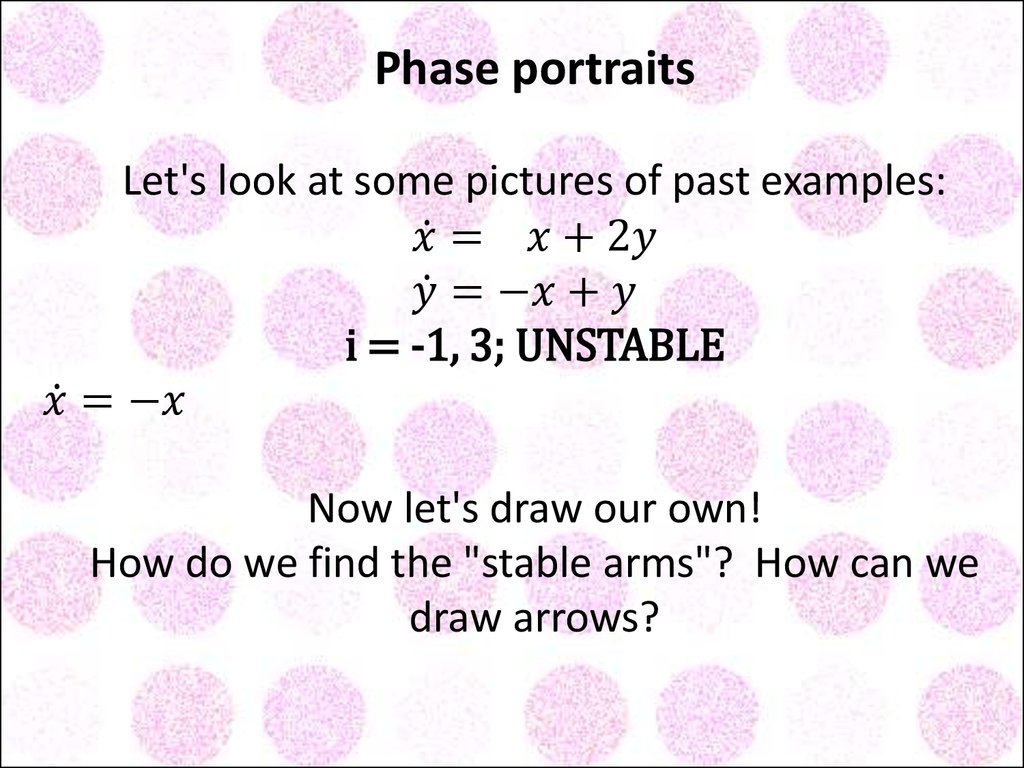
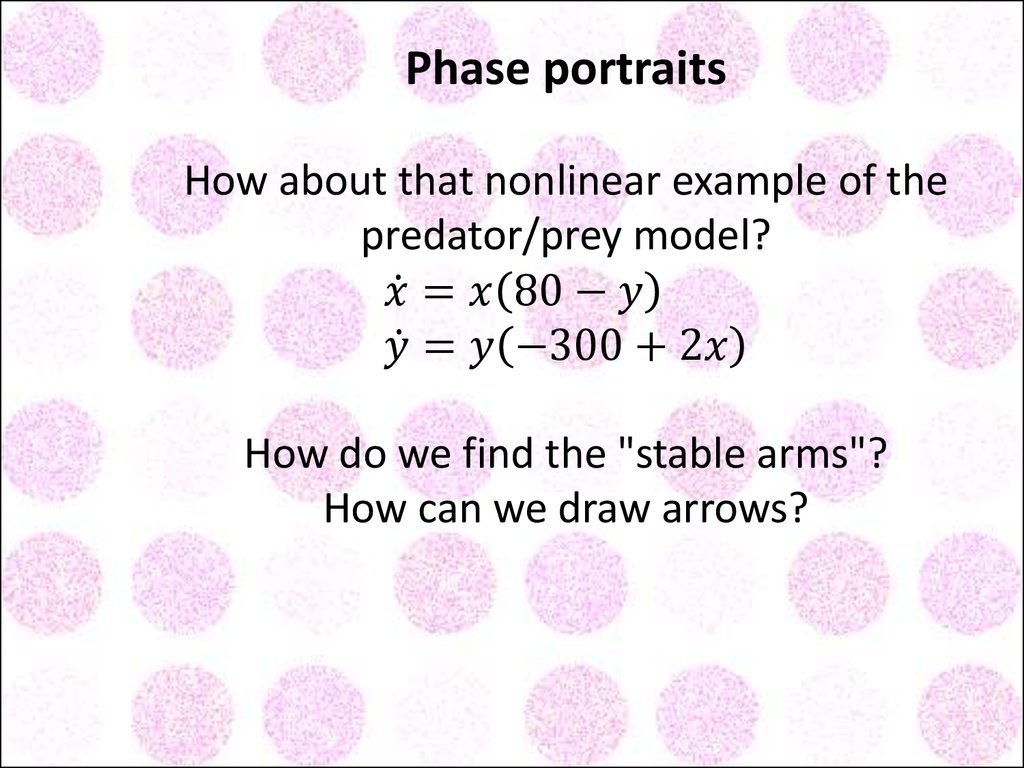
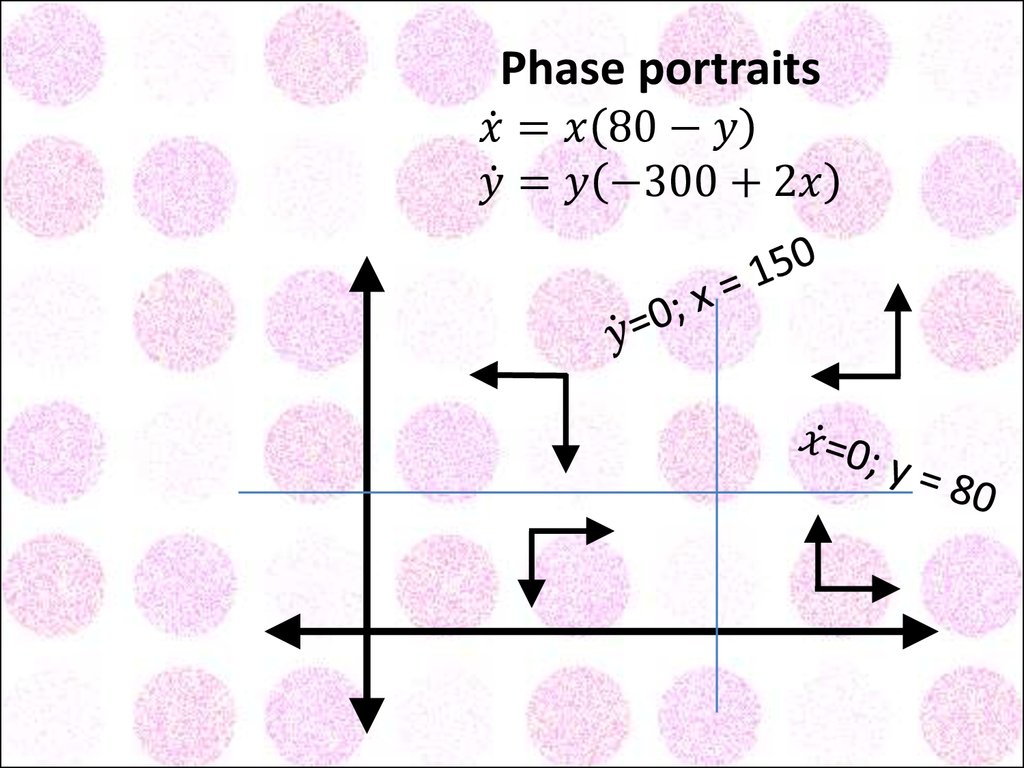
Phase portraits of systems
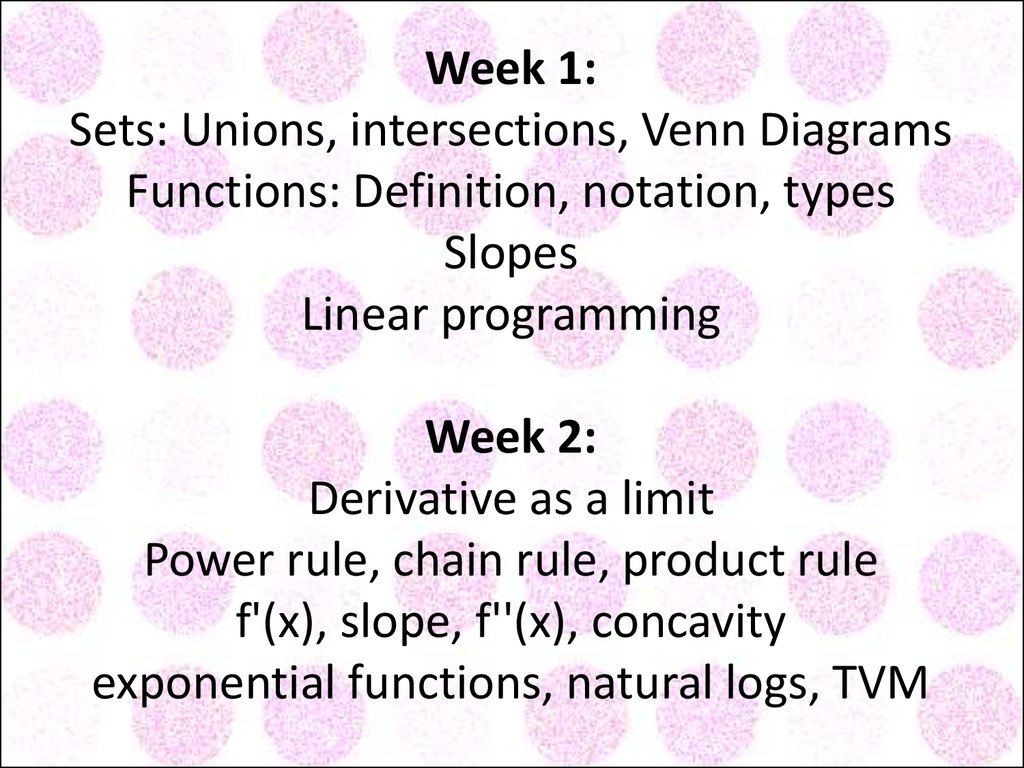
3. Week 1: Sets: Unions, intersections, Venn Diagrams Functions: Definition, notation, types Slopes Linear programming Week 2: Derivative as a limit Power rule, chain rule, product rule f'(x), slope, f''(x), concavity exponential functions, natural logs, TVM
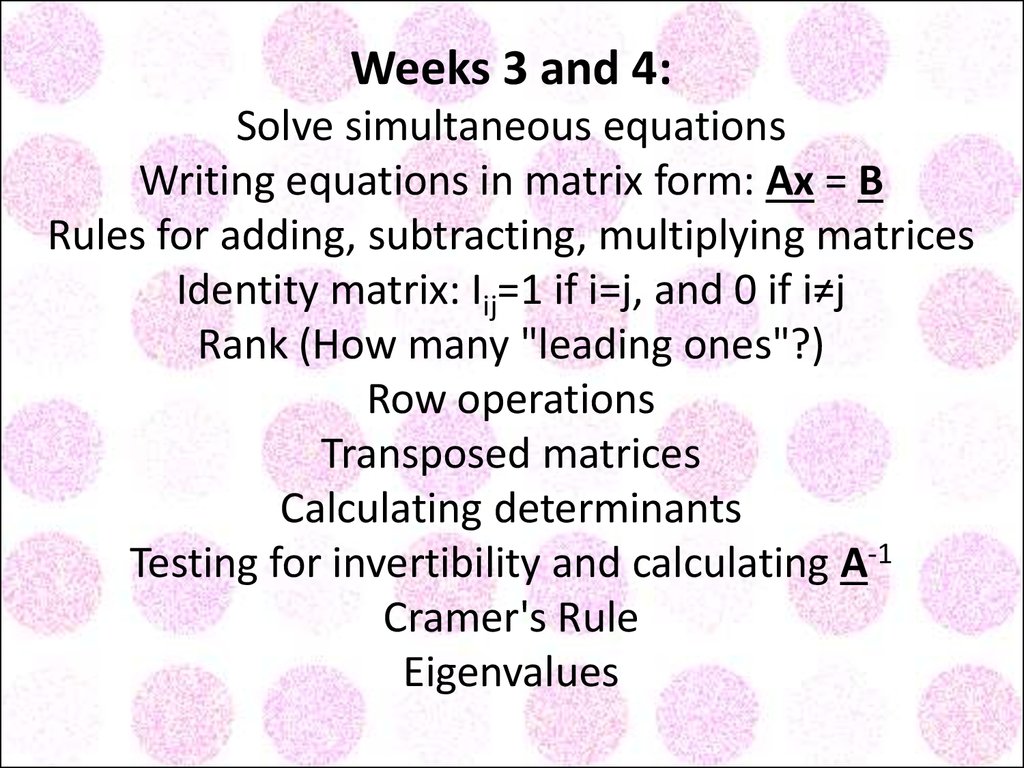
4. Weeks 3 and 4: Solve simultaneous equations Writing equations in matrix form: Ax = B Rules for adding, subtracting, multiplying matrices Identity matrix: Iij=1 if i=j, and 0 if i≠j Rank (How many "leading ones"?) Row operations Transposed matrices Calcu
Weeks 3 and 4:Solve simultaneous equations
Writing equations in matrix form: Ax = B
Rules for adding, subtracting, multiplying matrices
Identity matrix: Iij=1 if i=j, and 0 if i≠j
Rank (How many "leading ones"?)
Row operations
Transposed matrices
Calculating determinants
Testing for invertibility and calculating A-1
Cramer's Rule
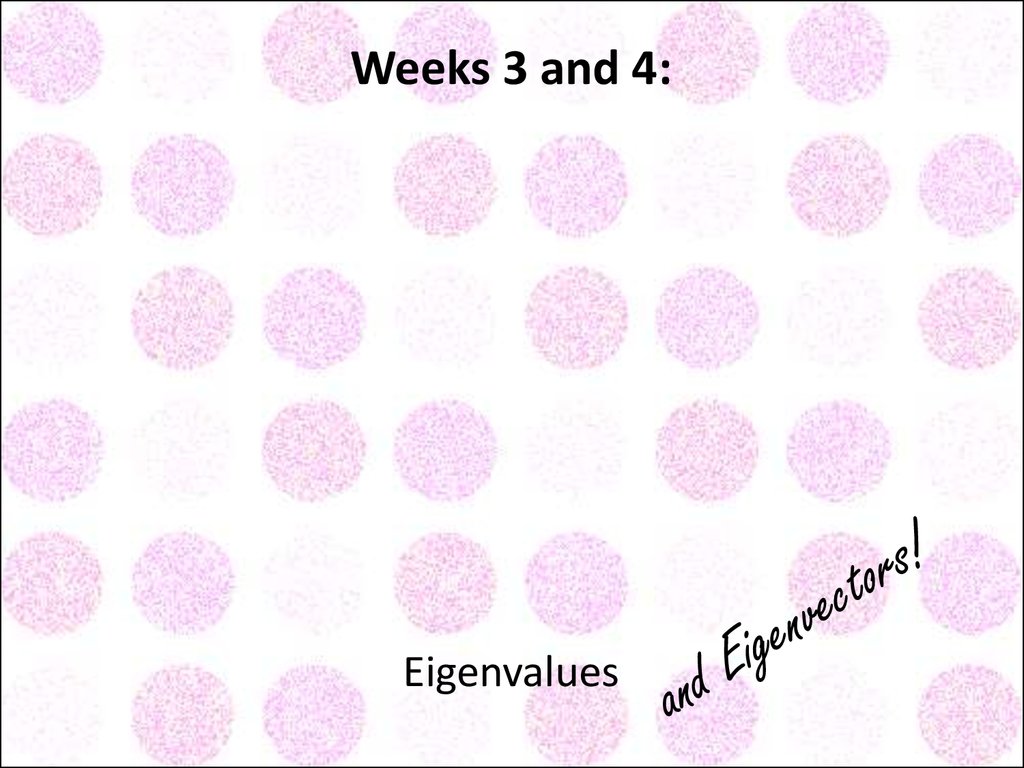
Eigenvalues
5. Weeks 3 and 4: Eigenvalues
6. Weeks 3 and 4: Example: [■8(2&1@1&2)] Eigenvalues
Weeks 3 and 4:Example:
2 1
1 2
Eigenvalues
7. Weeks 3 and 4: Example: [■8(2&1@1&2)] (2 - i)(2 - i) - 1 = 0 i2 - 4i + 4 - 1 = 0 i2 - 4i + 3 = 0 (i - 3)(i - 1) = 0, so i = 1, 3 Eigenvalues
Weeks 3 and 4:Example:
2 1
1 2
(2 - i)(2 - i) - 1 = 0
i2 - 4i + 4 - 1 = 0
i2 - 4i + 3 = 0
(i - 3)(i - 1) = 0, so i = 1, 3
Eigenvalues
8. Now... the vectors. Example: i = 1, 3 [■8(2&1@1&2)][■8(x@y)]=1[■8(x@y)], leading to x = -y [■8(2&1@1&2)][■8(x@y)]=3[■8(x@y)], leading to x = y Eigenvectors are v1 = [■8(1@-1)], v2 = [■8(1@1)]
Now... the vectors.Example:
i = 1, 3

![Weeks 3 and 4: Example: [■8(2&1@1&2)] Eigenvalues Weeks 3 and 4: Example: [■8(2&1@1&2)] Eigenvalues](https://cf.ppt-online.org/files/slide/x/Xl9AuhxaYPQEVyMtgrnKpWoLjJDkvfIUOzB50F/slide-5.jpg)
![Weeks 3 and 4: Example: [■8(2&1@1&2)] (2 - i)(2 - i) - 1 = 0 i2 - 4i + 4 - 1 = 0 i2 - 4i + 3 = 0 (i - 3)(i - 1) = 0, so i = 1, 3 Eigenvalues Weeks 3 and 4: Example: [■8(2&1@1&2)] (2 - i)(2 - i) - 1 = 0 i2 - 4i + 4 - 1 = 0 i2 - 4i + 3 = 0 (i - 3)(i - 1) = 0, so i = 1, 3 Eigenvalues](https://cf.ppt-online.org/files/slide/x/Xl9AuhxaYPQEVyMtgrnKpWoLjJDkvfIUOzB50F/slide-6.jpg)
![Now... the vectors. Example: i = 1, 3 [■8(2&1@1&2)][■8(x@y)]=1[■8(x@y)], leading to x = -y [■8(2&1@1&2)][■8(x@y)]=3[■8(x@y)], leading to x = y Eigenvectors are v1 = [■8(1@-1)], v2 = [■8(1@1)] Now... the vectors. Example: i = 1, 3 [■8(2&1@1&2)][■8(x@y)]=1[■8(x@y)], leading to x = -y [■8(2&1@1&2)][■8(x@y)]=3[■8(x@y)], leading to x = y Eigenvectors are v1 = [■8(1@-1)], v2 = [■8(1@1)]](https://cf.ppt-online.org/files/slide/x/Xl9AuhxaYPQEVyMtgrnKpWoLjJDkvfIUOzB50F/slide-7.jpg)


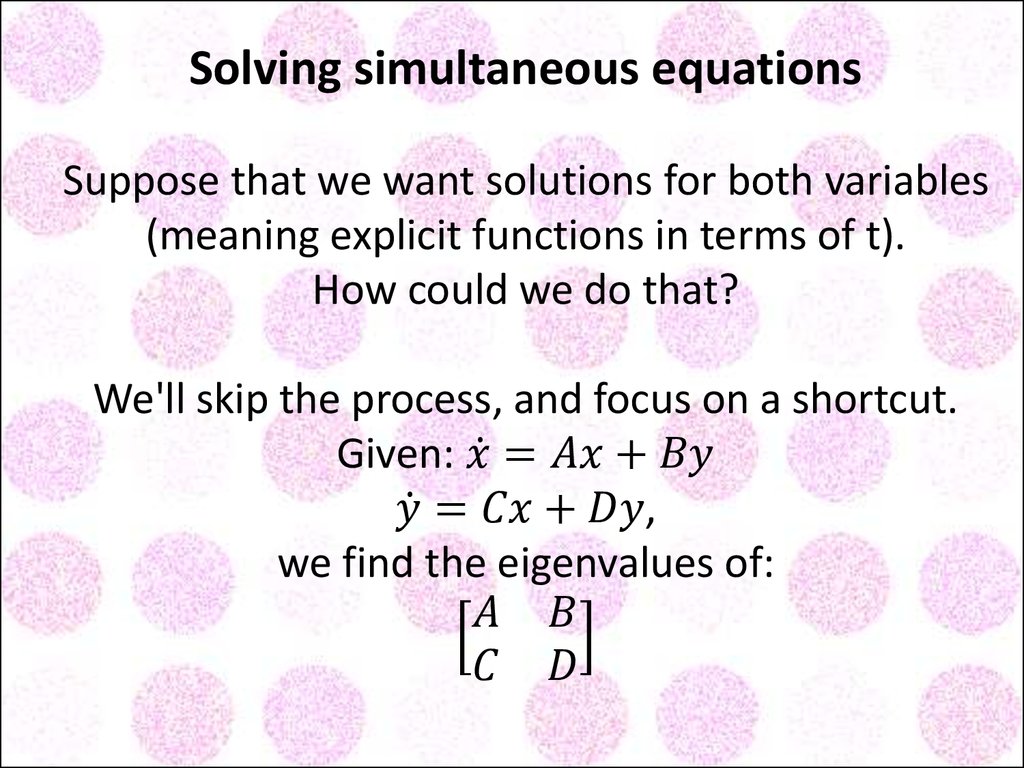
![Solving simultaneous equations [■8(A&B@C&D)] Next, we take the eigenvalues i and calculate the eigenvectors with |A|v = iv. Finally, we use these for the general solution: x(t)=c_1 v_1,1 e^(i_1 t)+c_2 v_1,2 e^(i_2 t) y(t)=c_1 v_2,1 e^(i_1 t)+c_2 v_2,2 e Solving simultaneous equations [■8(A&B@C&D)] Next, we take the eigenvalues i and calculate the eigenvectors with |A|v = iv. Finally, we use these for the general solution: x(t)=c_1 v_1,1 e^(i_1 t)+c_2 v_1,2 e^(i_2 t) y(t)=c_1 v_2,1 e^(i_1 t)+c_2 v_2,2 e](https://cf.ppt-online.org/files/slide/x/Xl9AuhxaYPQEVyMtgrnKpWoLjJDkvfIUOzB50F/slide-16.jpg)
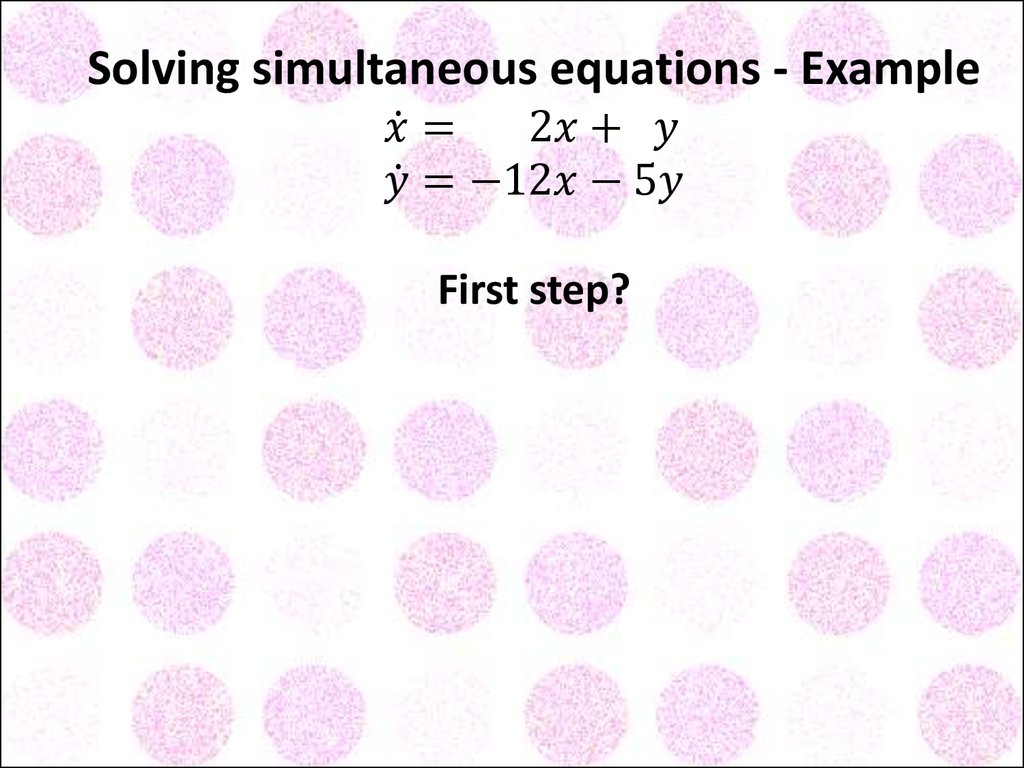
![Solving simultaneous equations - Example x ̇= 2x+ y y ̇=-12x-5y 1. Make matrix A. [■8(2&1@-12&-5)] Next step? Solving simultaneous equations - Example x ̇= 2x+ y y ̇=-12x-5y 1. Make matrix A. [■8(2&1@-12&-5)] Next step?](https://cf.ppt-online.org/files/slide/x/Xl9AuhxaYPQEVyMtgrnKpWoLjJDkvfIUOzB50F/slide-18.jpg)
![Solving simultaneous equations - Example x ̇= 2x+ y y ̇=-12x-5y 2. Find the eigenvalues. [■8(2&1@-12&-5)] Solving simultaneous equations - Example x ̇= 2x+ y y ̇=-12x-5y 2. Find the eigenvalues. [■8(2&1@-12&-5)]](https://cf.ppt-online.org/files/slide/x/Xl9AuhxaYPQEVyMtgrnKpWoLjJDkvfIUOzB50F/slide-19.jpg)
![Solving simultaneous equations - Example x ̇= 2x+ y y ̇=-12x-5y 2. Find the eigenvalues. [■8(2&1@-12&-5)] (2 - i)(-5 - i) + 12 = 0 10 + 5i - 2i + i2 + 12 = 0 i2 + 3i + 2 = 2 (i + 1)(i + 2) = 0, so i = -1, -2. Next step? Solving simultaneous equations - Example x ̇= 2x+ y y ̇=-12x-5y 2. Find the eigenvalues. [■8(2&1@-12&-5)] (2 - i)(-5 - i) + 12 = 0 10 + 5i - 2i + i2 + 12 = 0 i2 + 3i + 2 = 2 (i + 1)(i + 2) = 0, so i = -1, -2. Next step?](https://cf.ppt-online.org/files/slide/x/Xl9AuhxaYPQEVyMtgrnKpWoLjJDkvfIUOzB50F/slide-20.jpg)
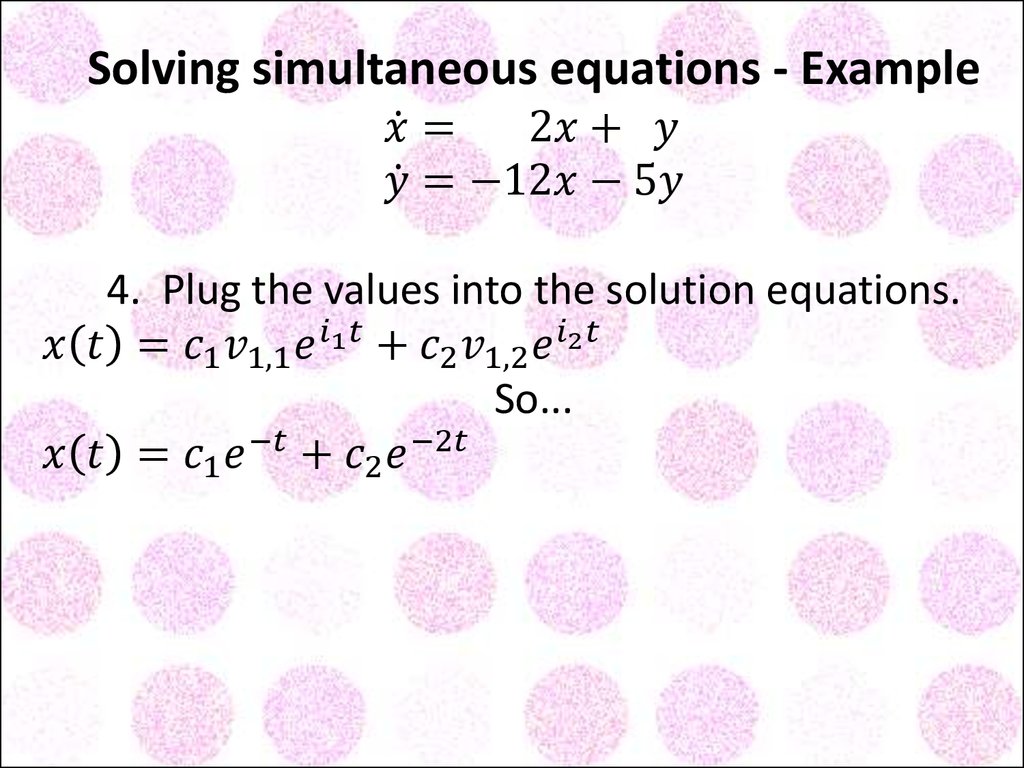
![Solving simultaneous equations - Example x ̇= 2x+ y y ̇=-12x-5y 3. Find the eigenvectors that go with each eigenvalue. i = -1, -2 [■8(2&1@-12&-5)][■8(x@y)]=-1[■8(x@y)], leading to ____________ [■8(2&1@-12&-5)][■8(x@y)]=-2[■8(x@y)], leading Solving simultaneous equations - Example x ̇= 2x+ y y ̇=-12x-5y 3. Find the eigenvectors that go with each eigenvalue. i = -1, -2 [■8(2&1@-12&-5)][■8(x@y)]=-1[■8(x@y)], leading to ____________ [■8(2&1@-12&-5)][■8(x@y)]=-2[■8(x@y)], leading](https://cf.ppt-online.org/files/slide/x/Xl9AuhxaYPQEVyMtgrnKpWoLjJDkvfIUOzB50F/slide-21.jpg)
![Solving simultaneous equations - Example x ̇= 2x+ y y ̇=-12x-5y 3. Find the eigenvectors that go with each eigenvalue. i = -1, -2 [■8(2&1@-12&-5)][■8(x@y)]=-1[■8(x@y)], leading to 3x = -y, so [■8(1@-3)] [■8(2&1@-12&-5)][■8(x@y)]=-2[■8(x@ Solving simultaneous equations - Example x ̇= 2x+ y y ̇=-12x-5y 3. Find the eigenvectors that go with each eigenvalue. i = -1, -2 [■8(2&1@-12&-5)][■8(x@y)]=-1[■8(x@y)], leading to 3x = -y, so [■8(1@-3)] [■8(2&1@-12&-5)][■8(x@y)]=-2[■8(x@](https://cf.ppt-online.org/files/slide/x/Xl9AuhxaYPQEVyMtgrnKpWoLjJDkvfIUOzB50F/slide-22.jpg)


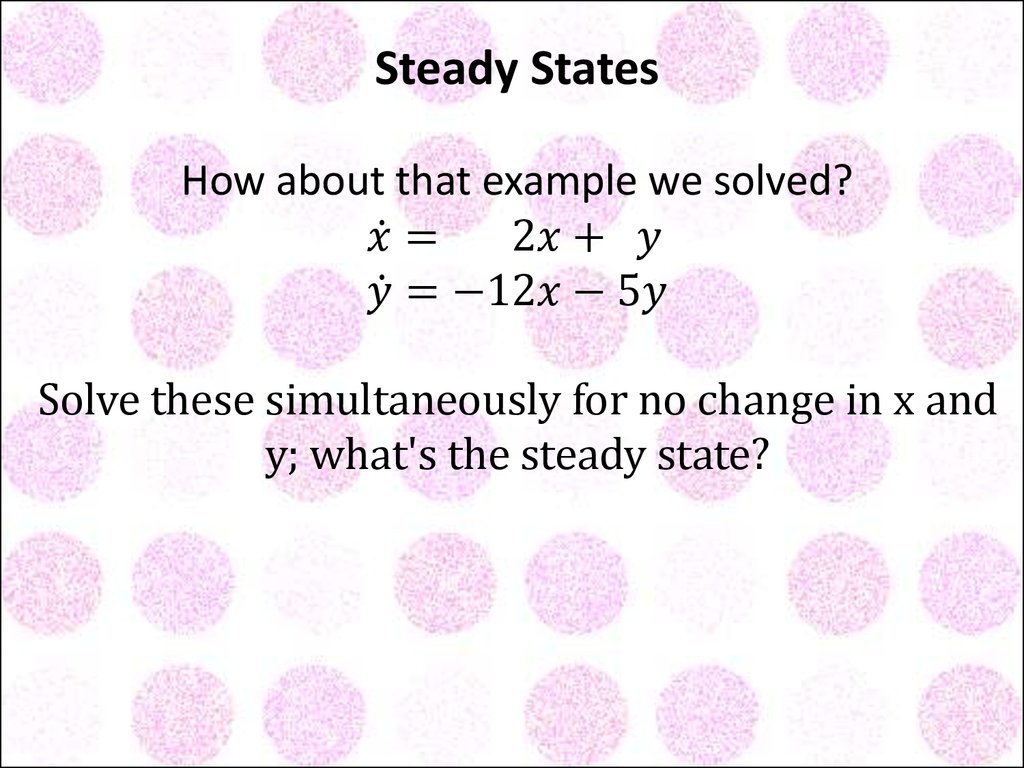

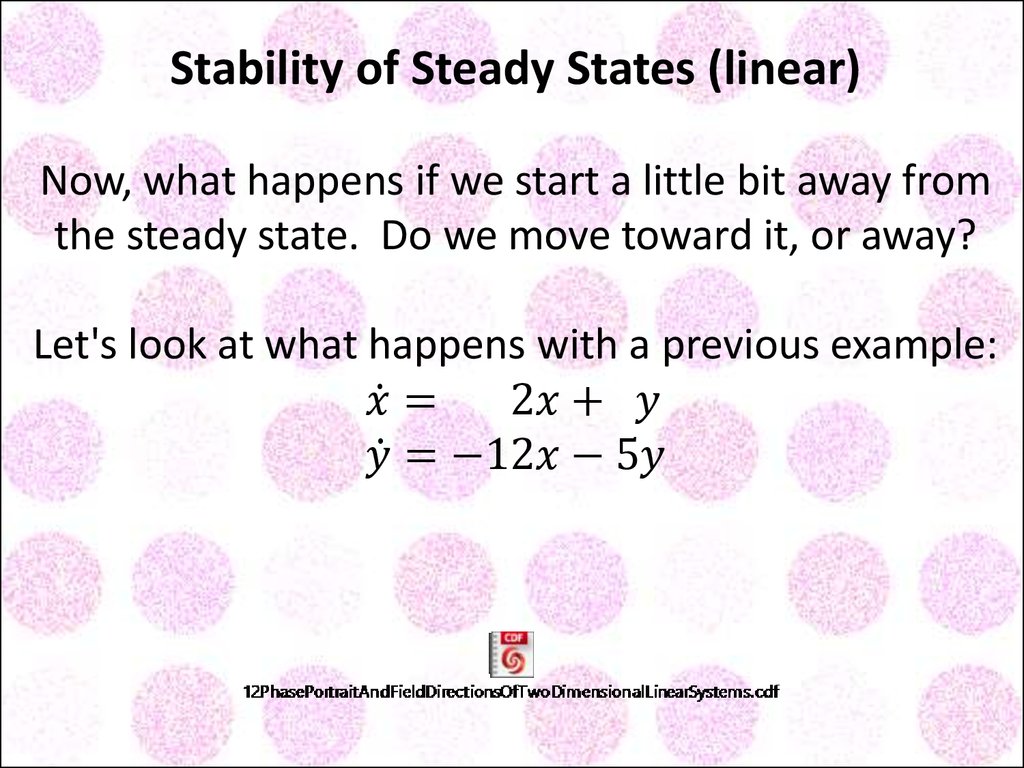

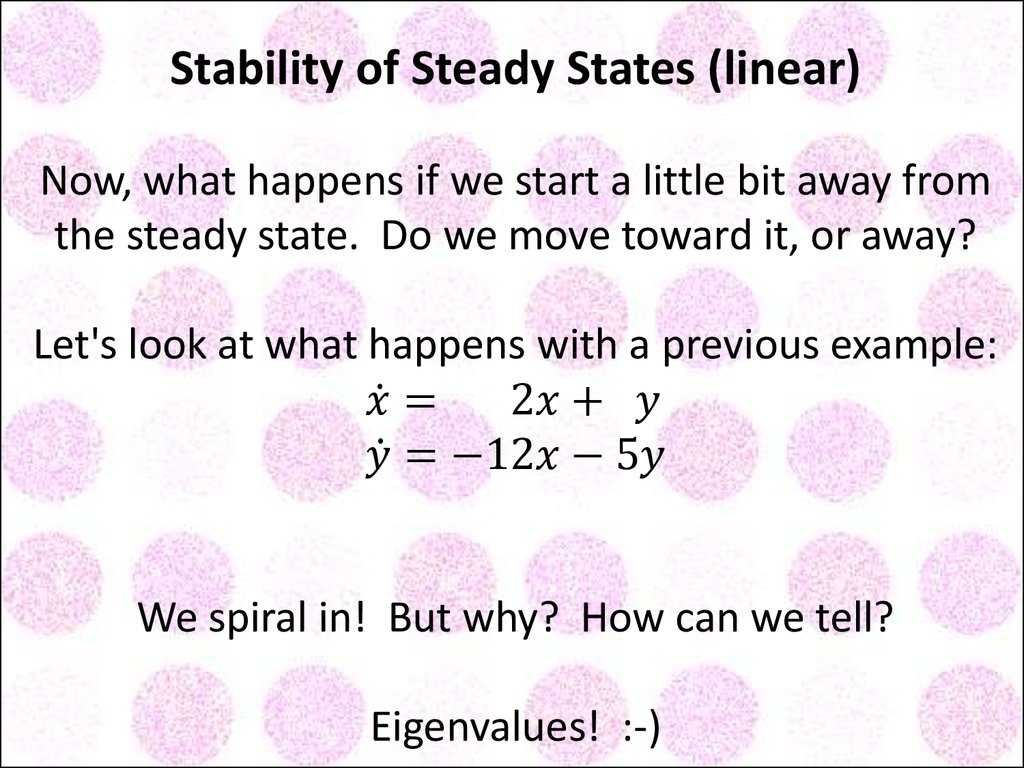
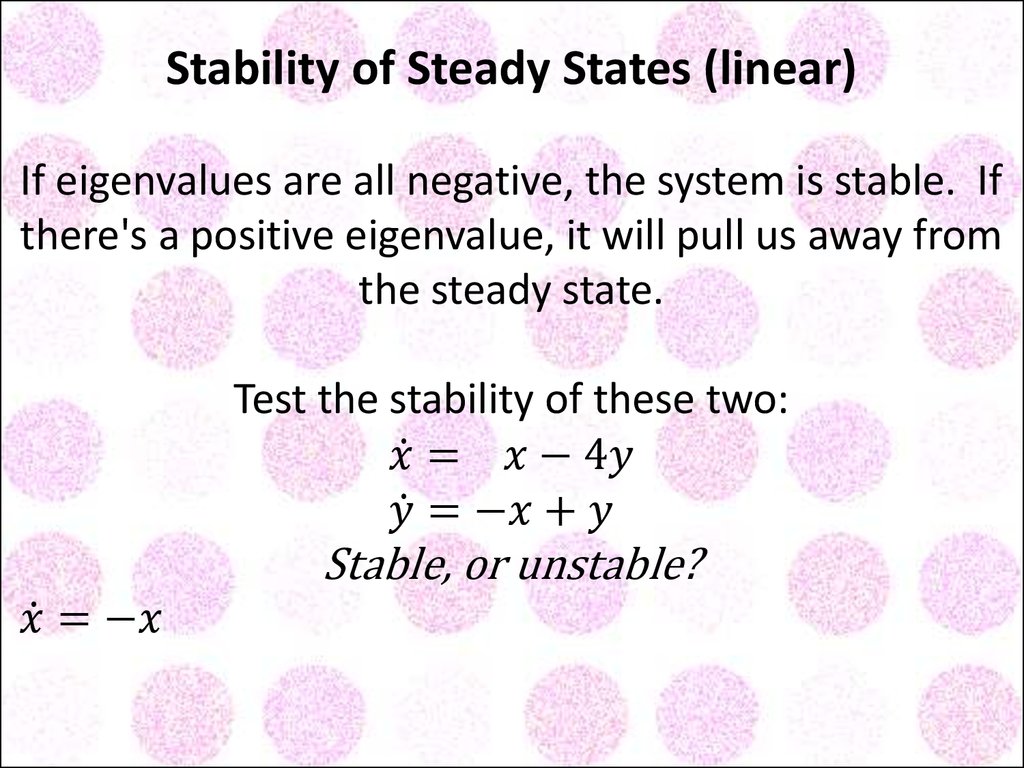


 mathematics
mathematics








