PID controller Design

1.

Review past lectures & Continuation of 
PID Controller Design
Md Hazrat Ali
Department of Mechanical Engineering
School of Engineering,
Nazarbayev University

2.

Today’s Quote:
“Good, better, best. Never let it rest. Til your good is better
and your better is best.”
― St. Jerome

3.

Classical ControllerPID Controller

4.

Introduction
Design PID control
Know mathematical model various design techniques
Plant is complicated, can’t obtain mathematical model
experimental approaches to the tuning of PID controllers

5. PID Control

PID Control
A closed loop (feedback) control system, generally with 
Single Input­Single Output (SISO)
A portion of the signal being fed back is:
Proportional to the signal  (P)
Proportional to integral of the signal (I)
Proportional to the derivative of the signal (D)

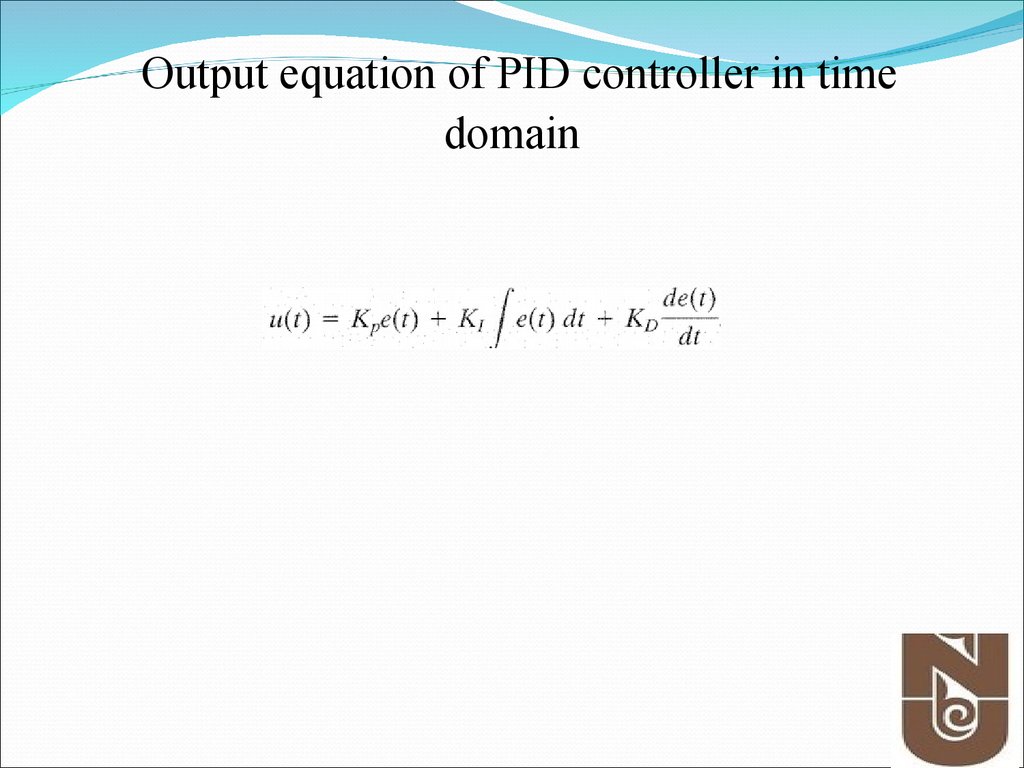
6. Output equation of PID controller in time domain

7.

The Characteristics of P, I, and D controllers
CL RESPONSE
RISE TIME
OVERSHOOT
SETTLING TIME
S-S ERROR
Kp
Decrease
Increase
Small Change
Decrease
Ki
Decrease
Increase
Increase
Eliminate
Kd
Small Change
Decrease
Decrease
Small Change

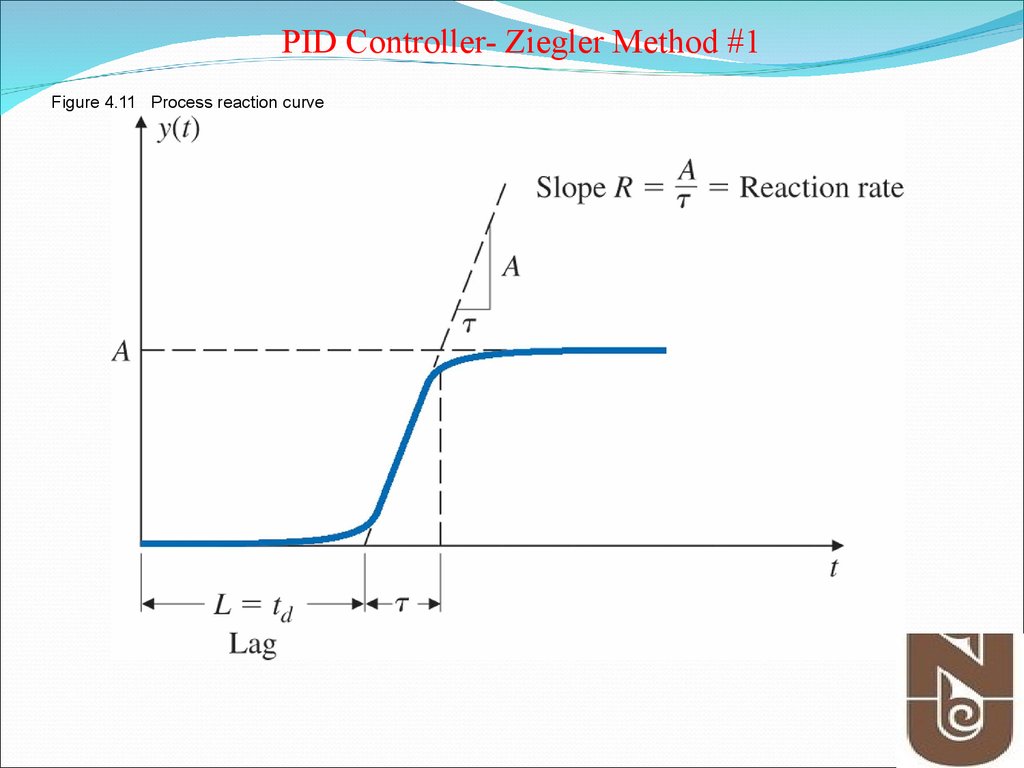
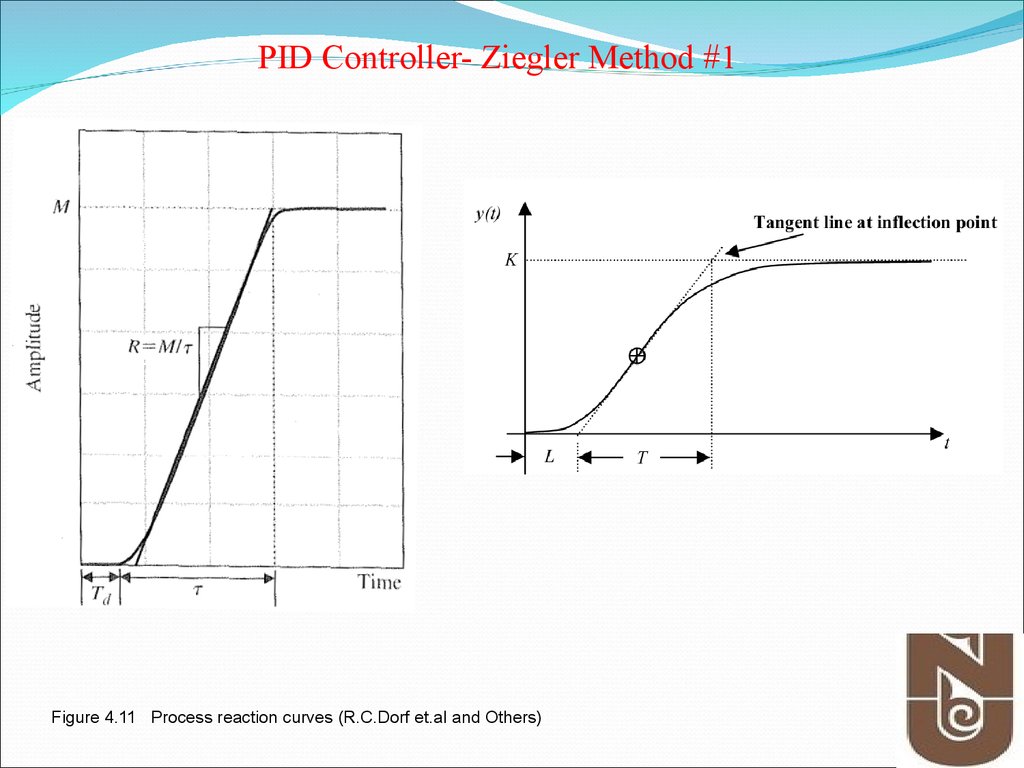
8. Figure 4.11 Process reaction curve

PID Controller- Ziegler Method #1
Figure 4.11 Process reaction curve

9.

PID Controller- Ziegler Method #1
Figure 4.11 Process reaction curves (R.C.Dorf et.al and Others)

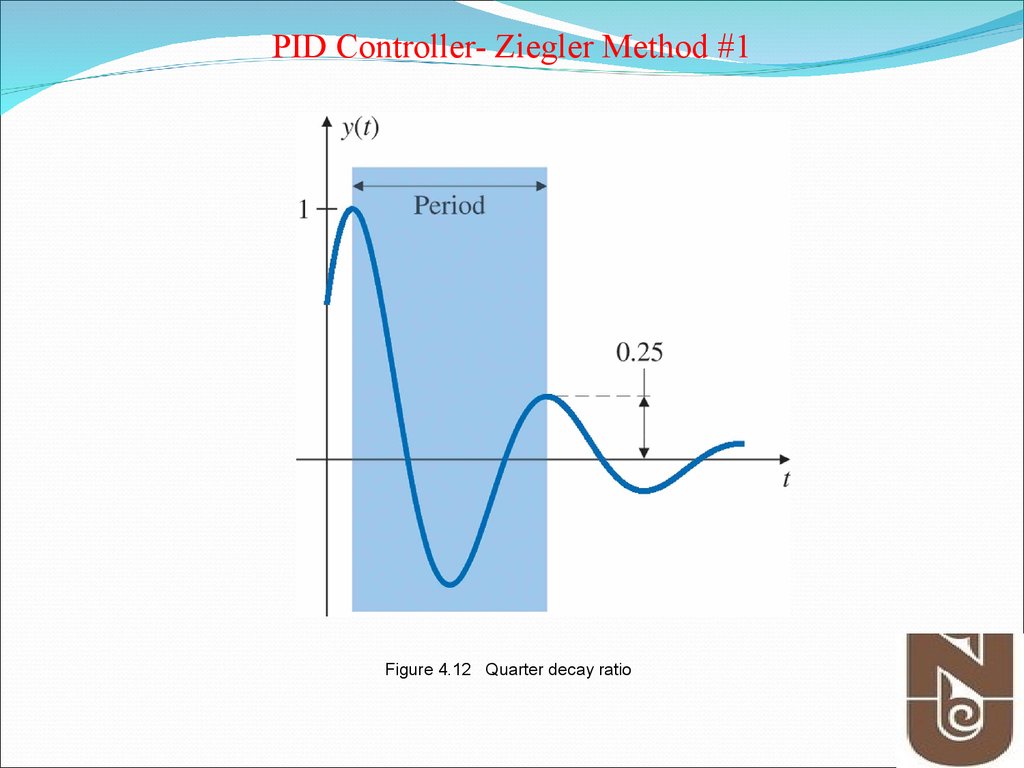
10. Figure 4.12 Quarter decay ratio

PID Controller- Ziegler Method #1
Figure 4.12 Quarter decay ratio

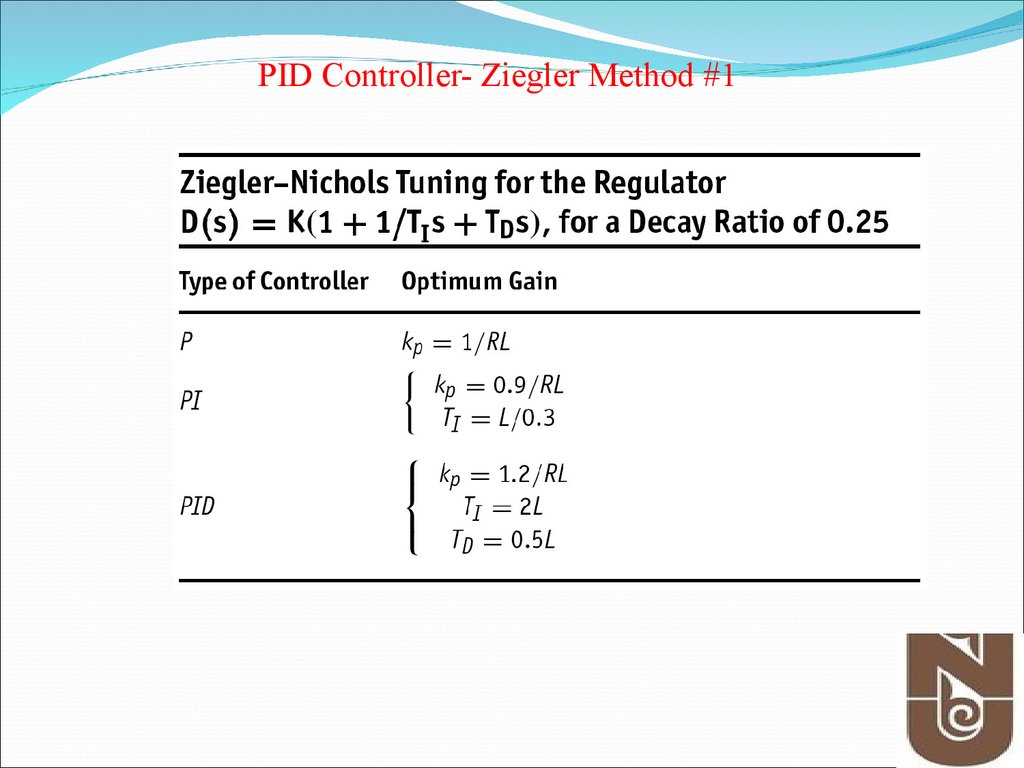
11.

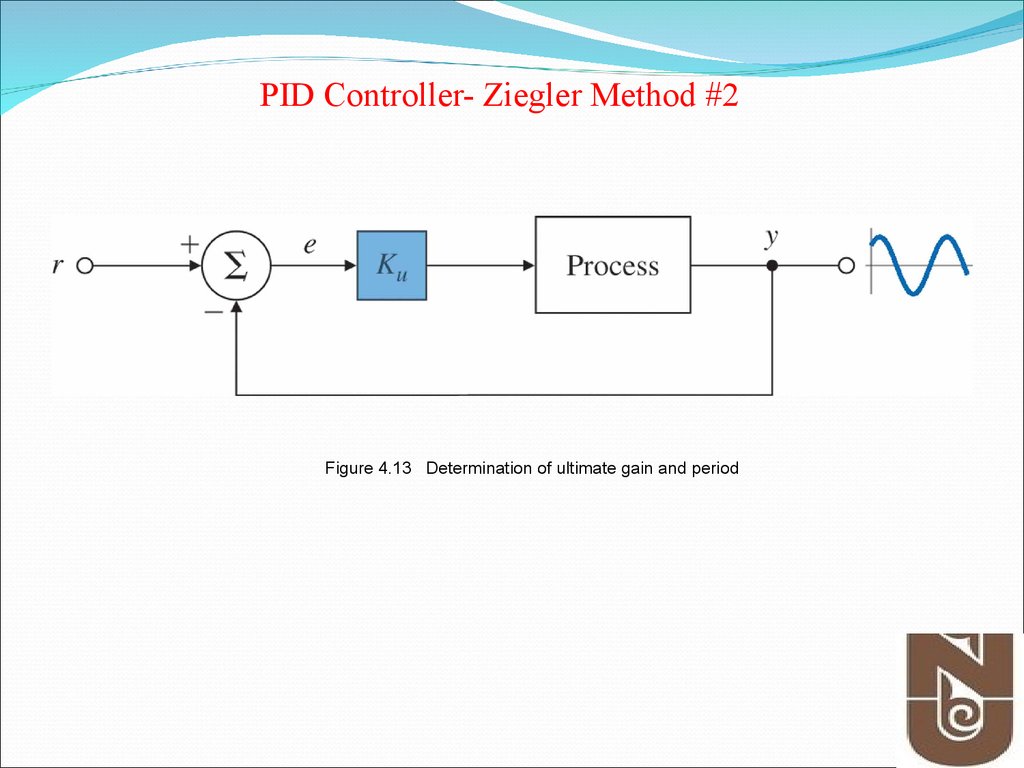
PID Controller- Ziegler Method #1

12. Figure 4.13 Determination of ultimate gain and period

PID Controller- Ziegler Method #2
Figure 4.13 Determination of ultimate gain and period

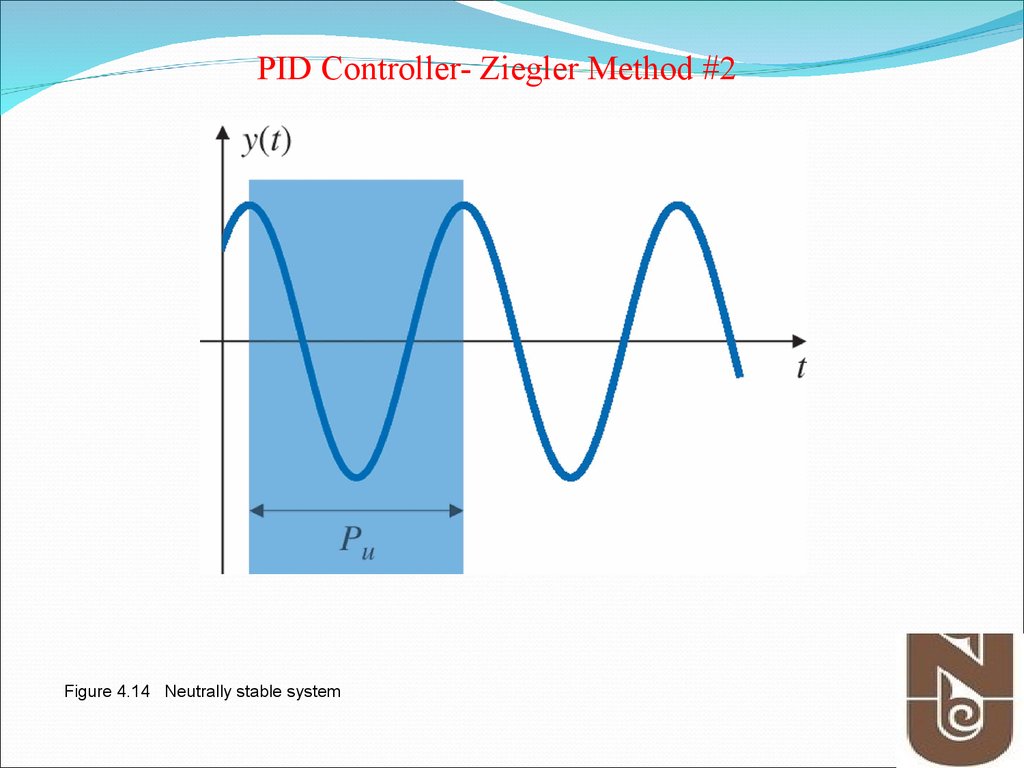
13. Figure 4.14 Neutrally stable system

PID Controller- Ziegler Method #2
Figure 4.14 Neutrally stable system

14.

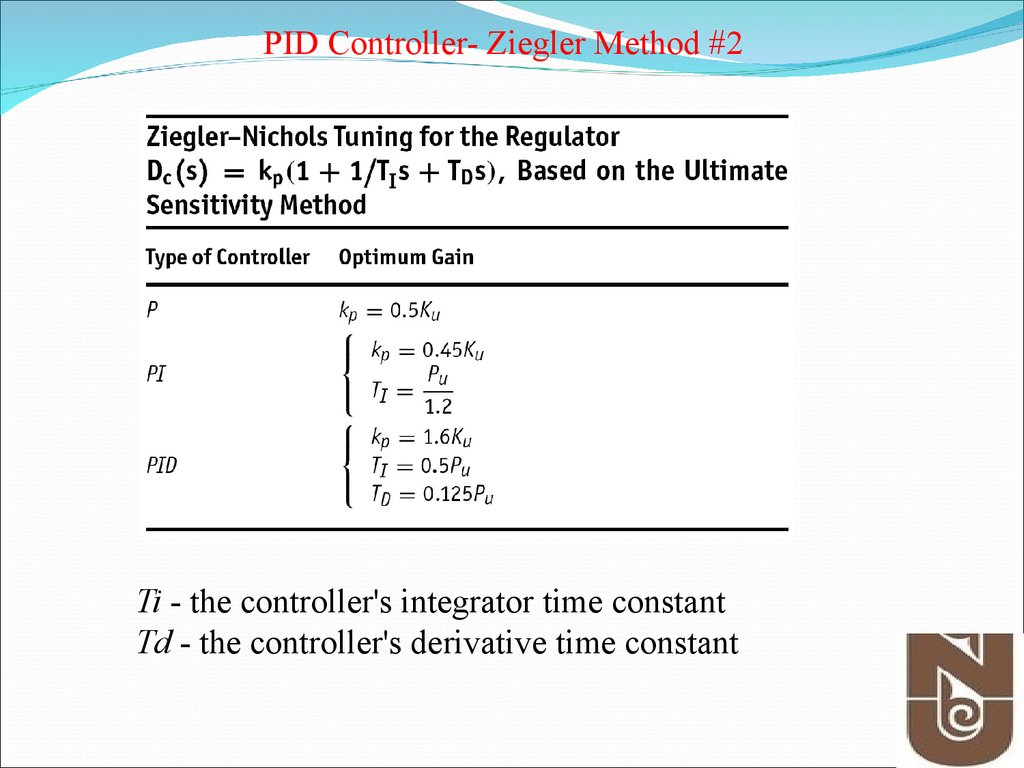
PID Controller- Ziegler Method #2
Ti - the controller's integrator time constant
Td - the controller's derivative time constant

15.

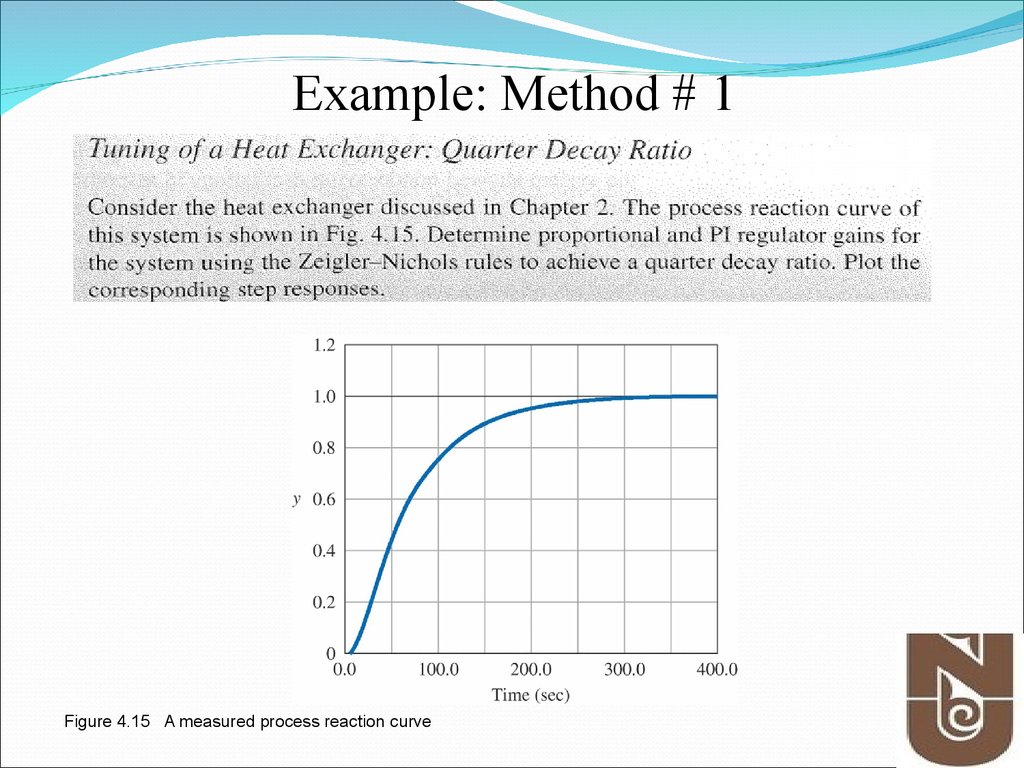
Example: Method # 1
Figure 4.15 A measured process reaction curve

16.

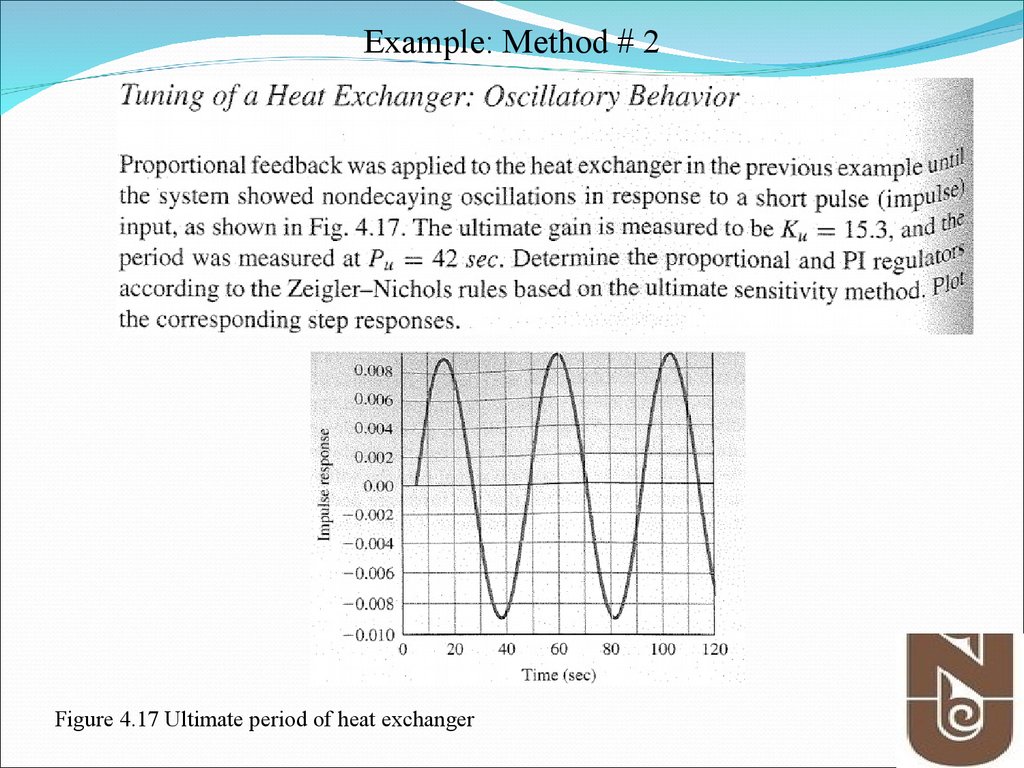
Example: Method # 2
Figure 4.17 Ultimate period of heat exchanger

17.

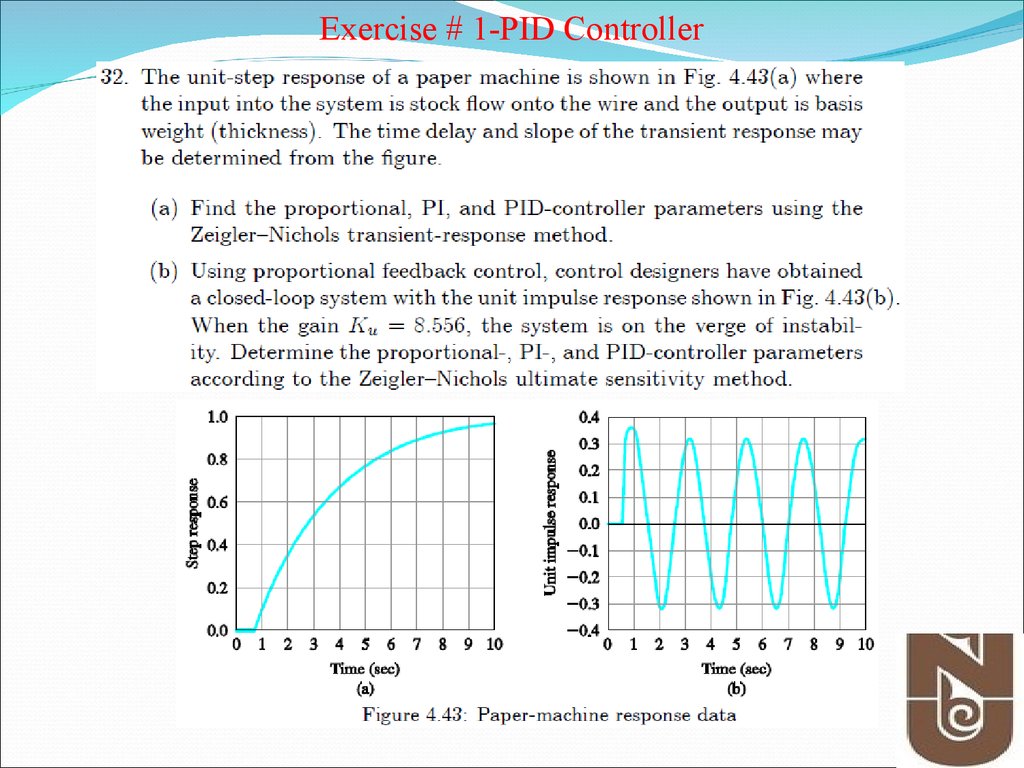
Exercise # 1-PID Controller

18.

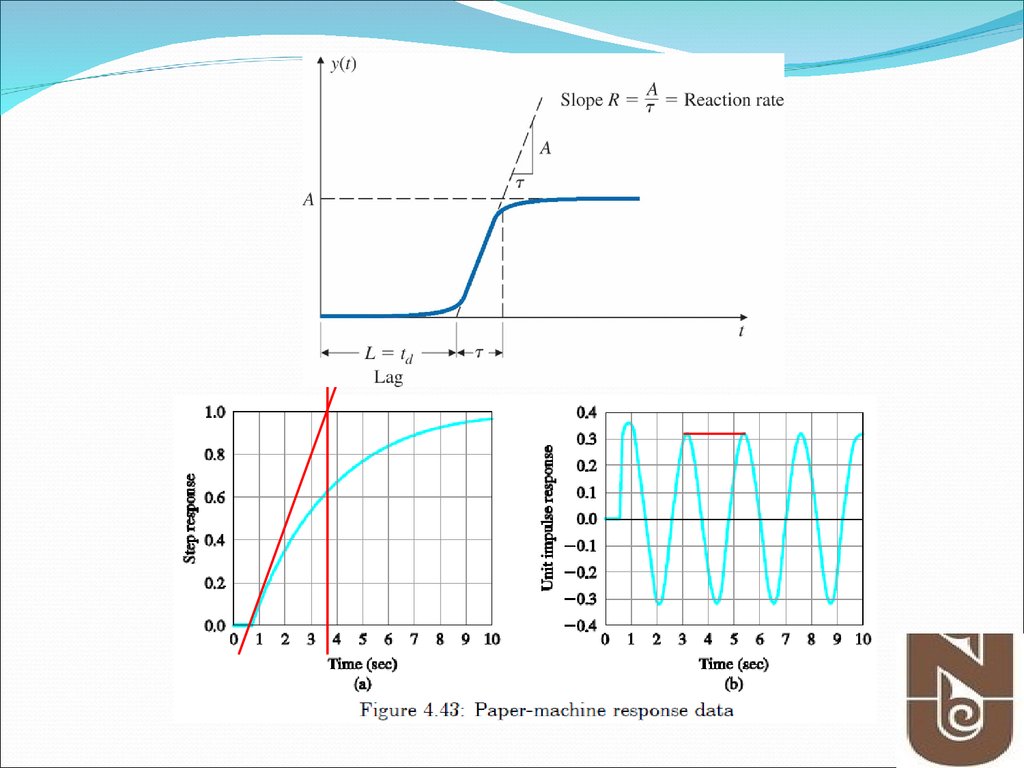
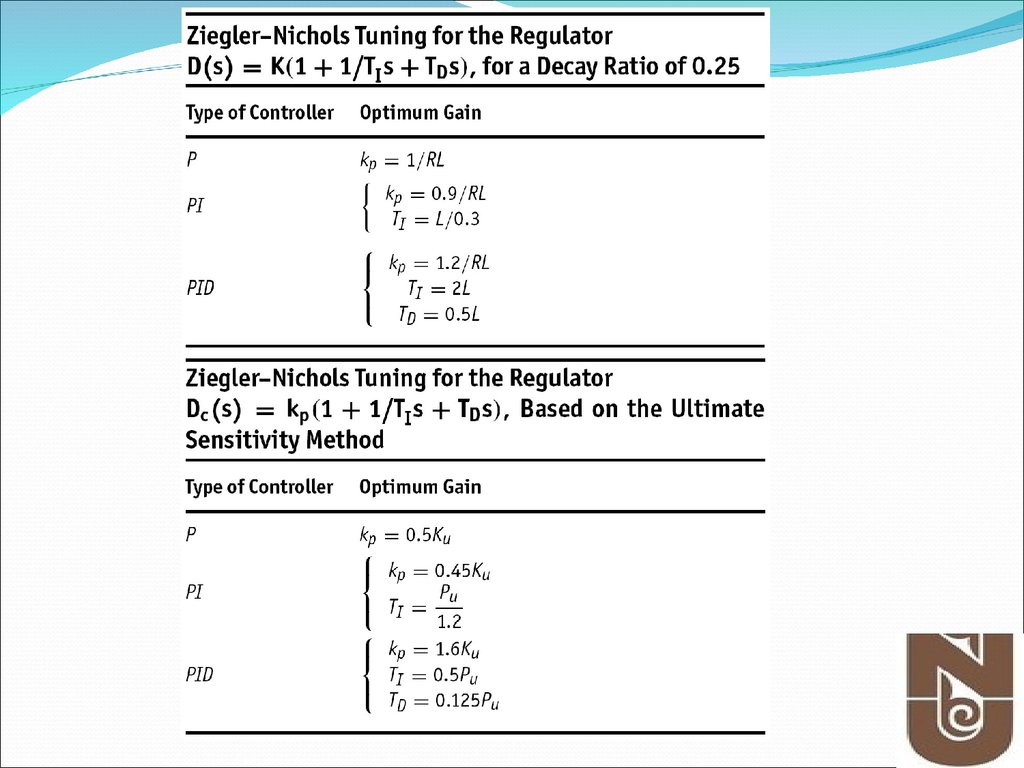
19.

20.

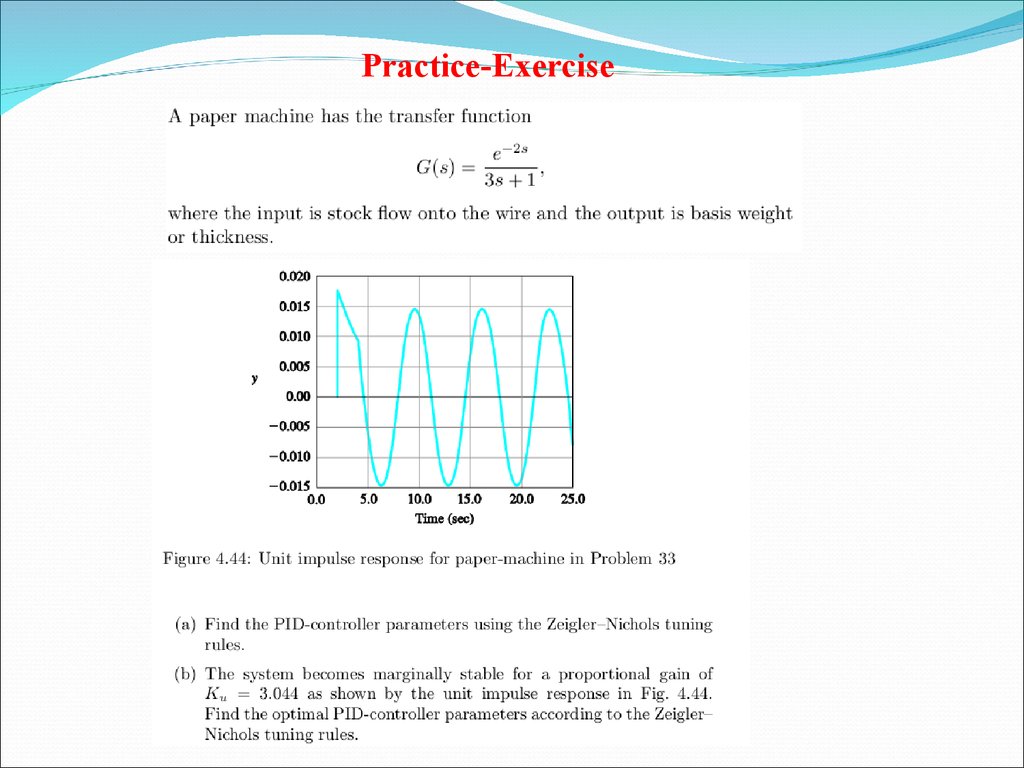
Practice-Exercise

21. Further Reading

Franklin, et. al., Chapter 4
Section 4.3
Richard C. Dorf et.al, Chapter 6,
Chapter 6.2