Nanophotonics class 4. Density of states

1.

Nanophotonics
Class 4
Density of states

2. Outline

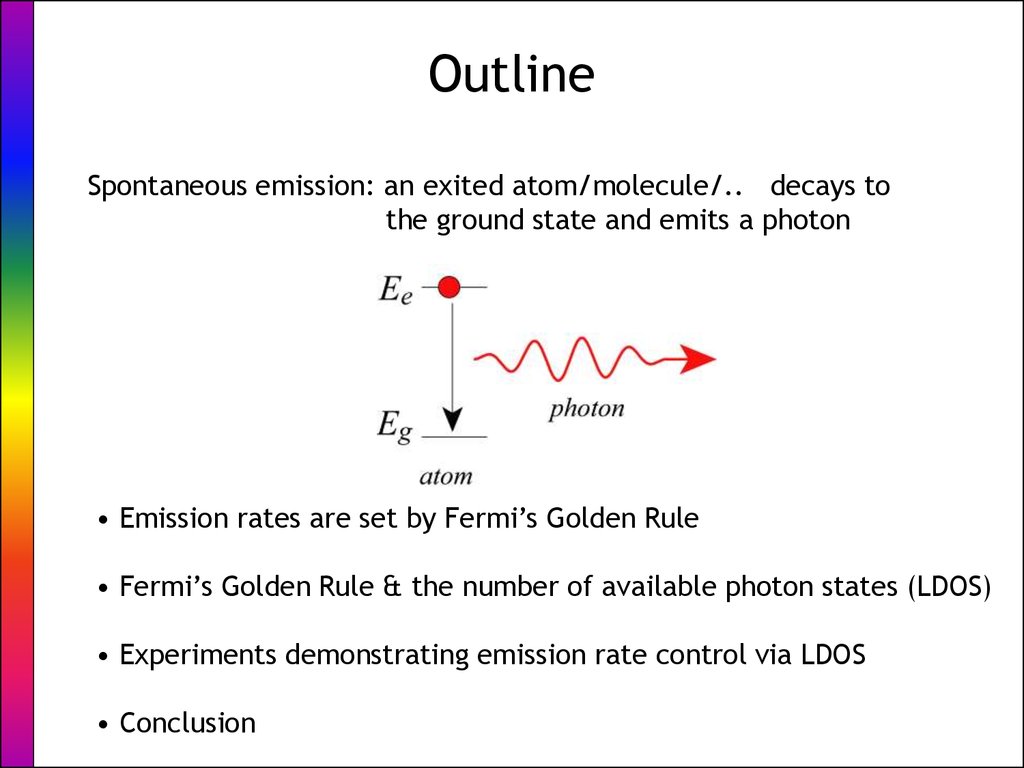
Spontaneous emission: an exited atom/molecule/.. decays to
the ground state and emits a photon
• Emission rates are set by Fermi’s Golden Rule
• Fermi’s Golden Rule & the number of available photon states (LDOS)
• Experiments demonstrating emission rate control via LDOS
• Conclusion

3. Fermi’s Golden Rule

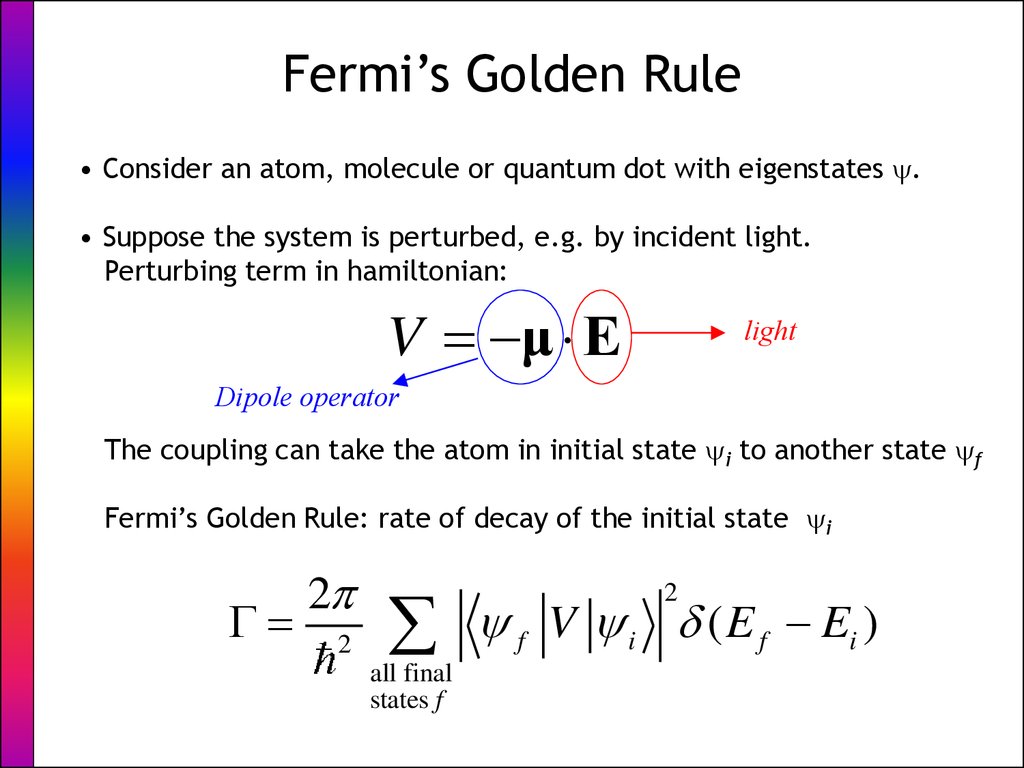
• Consider an atom, molecule or quantum dot with eigenstates y.
• Suppose the system is perturbed, e.g. by incident light.
Perturbing term in hamiltonian:
V μ E
light
Dipole operator
The coupling can take the atom in initial state yi to another state yf
Fermi’s Golden Rule: rate of decay of the initial state yi
2
2
all final
states f
2
y f V y i ( E f Ei )

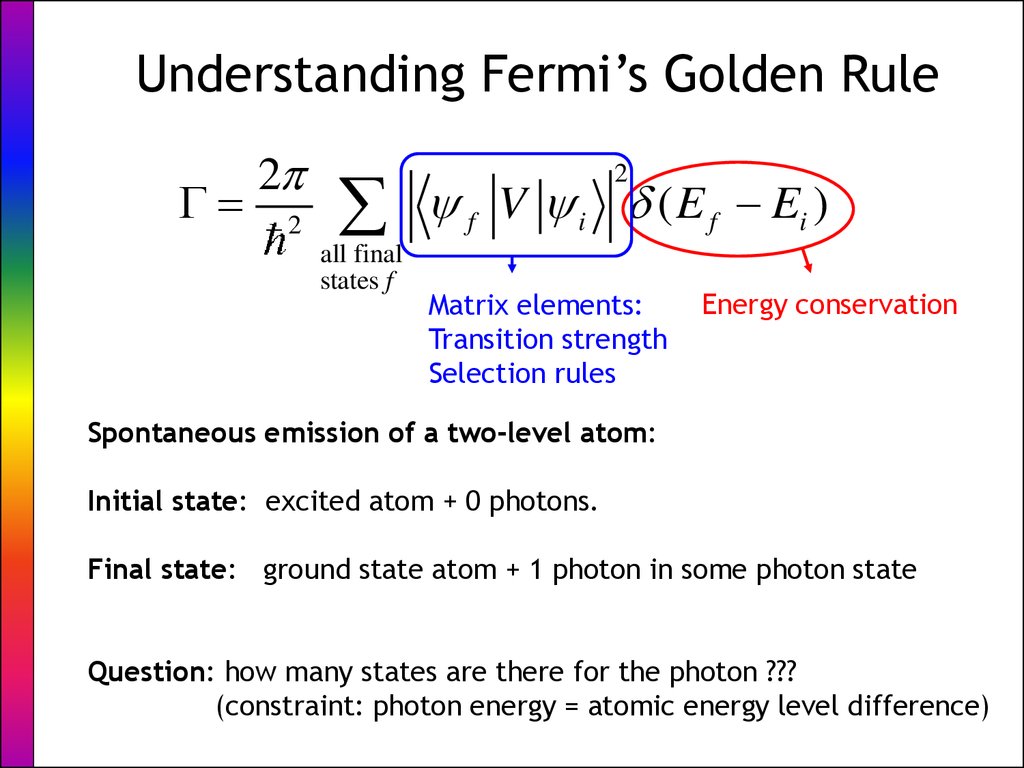
4. Understanding Fermi’s Golden Rule

2
2
all final
states f
2
y f V y i ( E f Ei )
Matrix elements:
Transition strength
Selection rules
Energy conservation
Spontaneous emission of a two-level atom:
Initial state: excited atom + 0 photons.
Final state: ground state atom + 1 photon in some photon state
Question: how many states are there for the photon ???
(constraint: photon energy = atomic energy level difference)

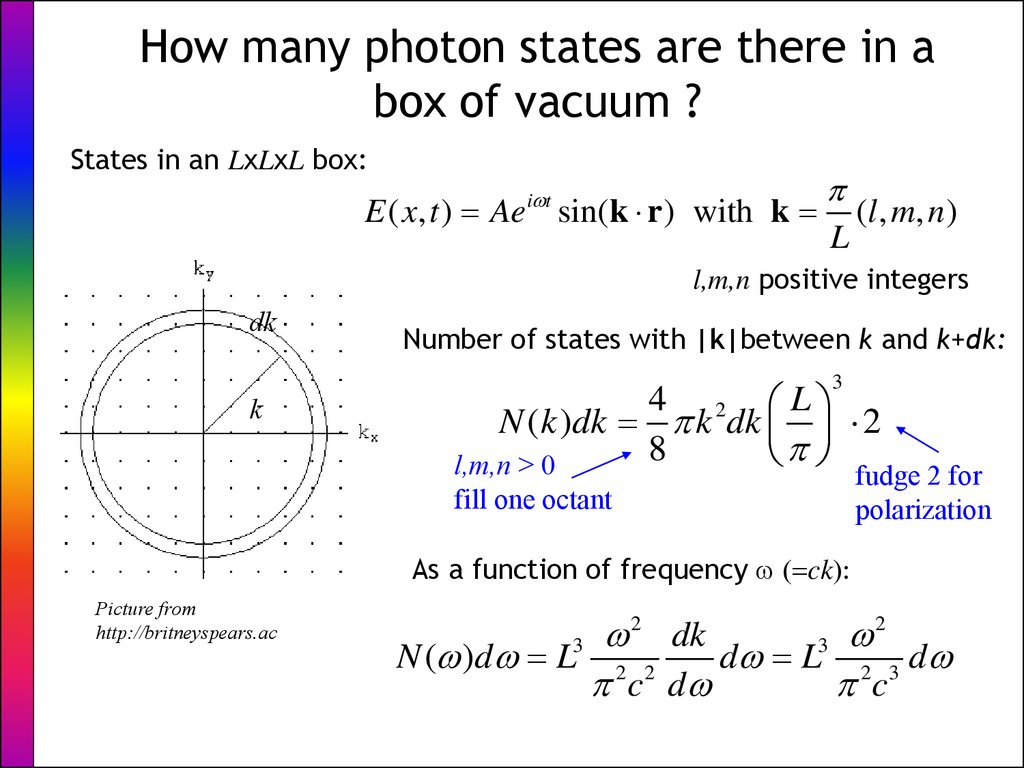
5. How many photon states are there in a box of vacuum ?

States in an LxLxL box:
E ( x, t ) Aei t sin( k r ) with k
L
(l , m, n)
l,m,n positive integers
dk
k
Number of states with |k|between k and k+dk:
3
4 2 L
N (k )dk k dk 2
8
l,m,n > 0
fill one octant
fudge 2 for
polarization
As a function of frequency ( ck):
Picture from
http://britneyspears.ac
2
2
dk
N ( )d L3 2 2
d L3 2 3 d
c d
c

6. Density of states in vacuum

2
2 dk
3
N ( )d L 2 2
d L 2 3 d
c d
c
3
-1
Density of photon states per unit volume (s )
Example: ~50000 photon states per m3 of vacuum per 1 Hz @ l=500 nm
150000
100000
~ 50000 states
50000
l nm
0
0
2
4
15
6
-1
Frequency (10 s )

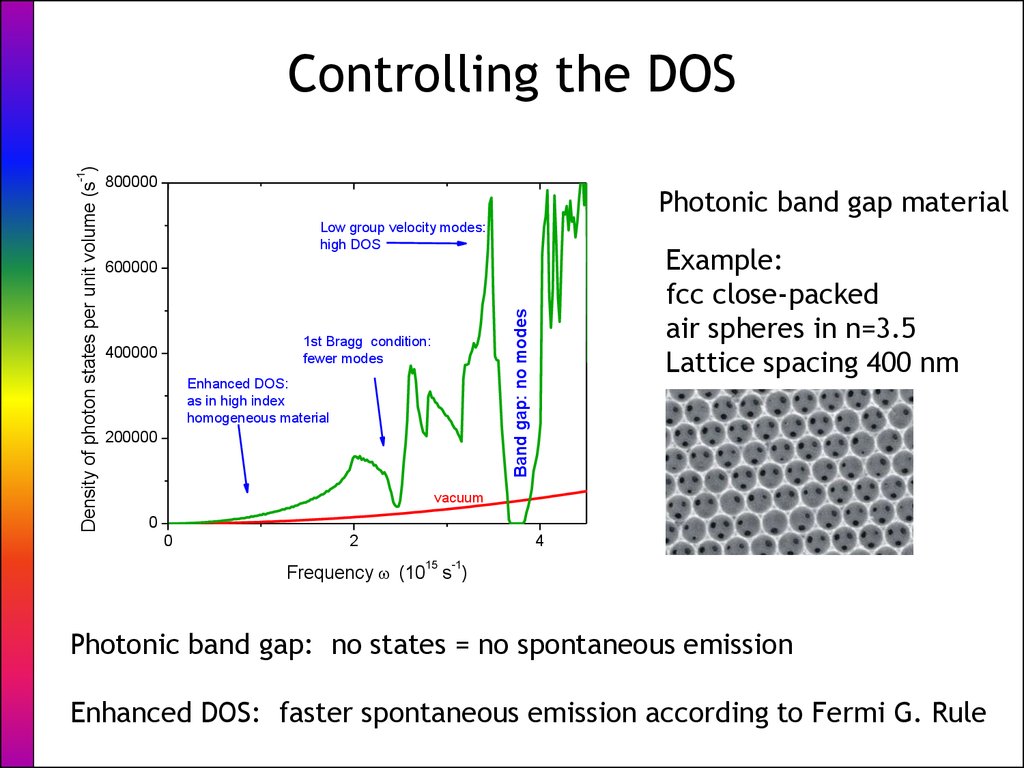
7. Controlling the DOS

800000
Photonic band gap material
Low group velocity modes:
high DOS
Example:
fcc close-packed
air spheres in n=3.5
Lattice spacing 400 nm
600000
Band gap: no modes
-1
Density of photon states per unit volume (s )
Controlling the DOS
1st Bragg condition:
fewer modes
400000
Enhanced DOS:
as in high index
homogeneous material
200000
vacuum
0
0
2
4
15
-1
Frequency (10 s )
1 m
Photonic band gap: no states = no spontaneous emission
Enhanced DOS: faster spontaneous emission according to Fermi G. Rule

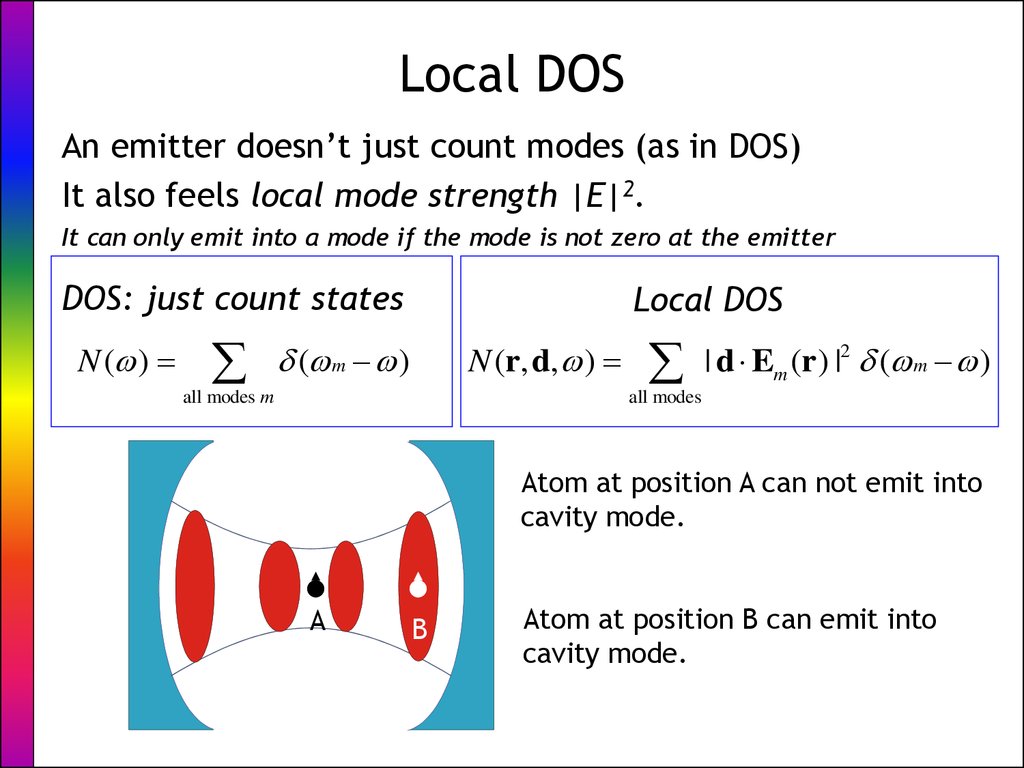
8. Local DOS

An emitter doesn’t just count modes (as in DOS)
It also feels local mode strength |E|2.
It can only emit into a mode if the mode is not zero at the emitter
DOS: just count states
N ( )
Local DOS
N (r, d, )
( m )
all modes m
| d Em (r) |2 ( m )
all modes
Atom at position A can not emit into
cavity mode.
A
B
Atom at position B can emit into
cavity mode.

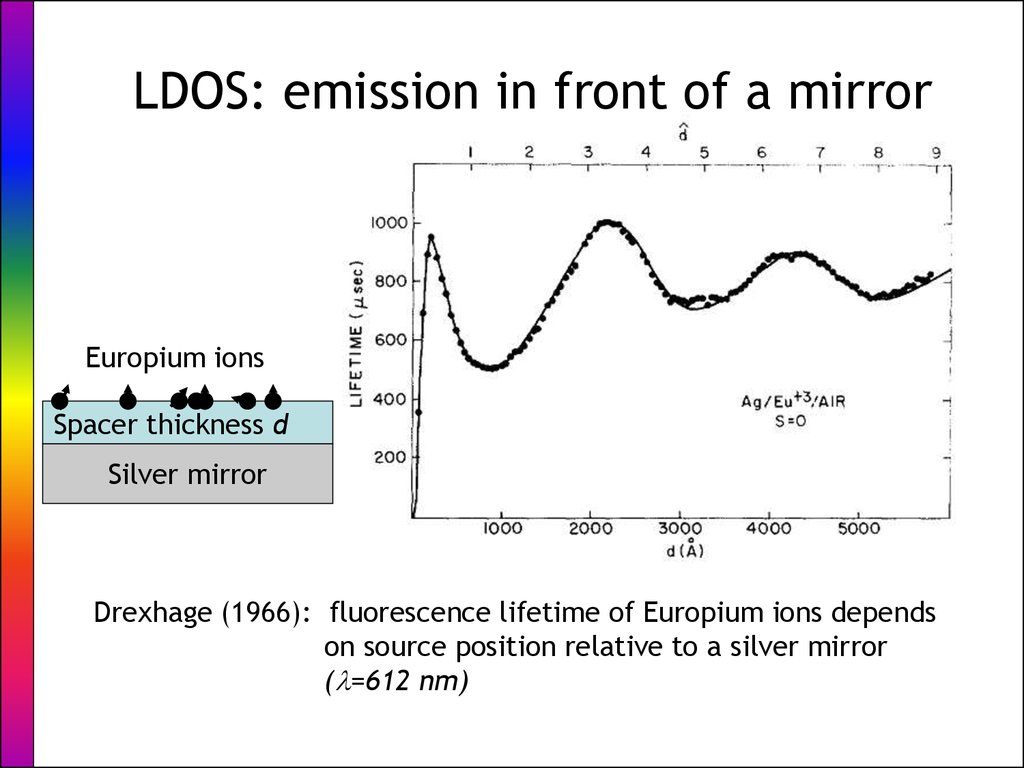
9. LDOS: emission in front of a mirror

Europium ions
Spacer thickness d
Silver mirror
Drexhage (1966): fluorescence lifetime of Europium ions depends
on source position relative to a silver mirror
(l=612 nm)

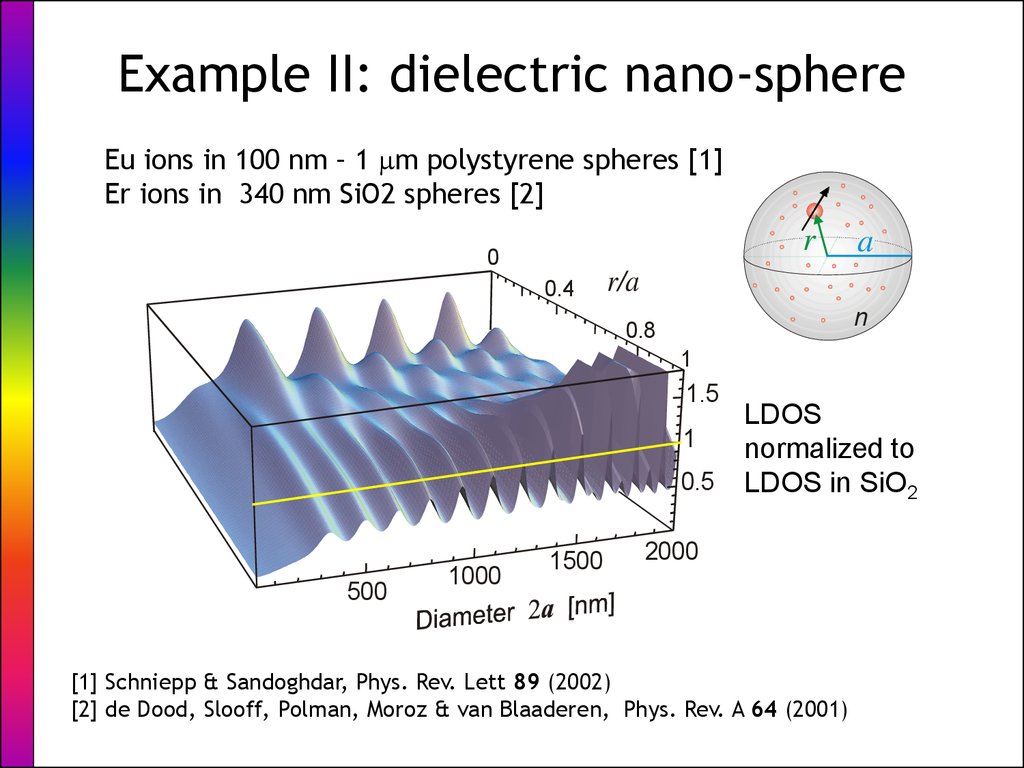
10. Example II: dielectric nano-sphere

Eu ions in 100 nm – 1 m polystyrene spheres [1]
Er ions in 340 nm SiO2 spheres [2]
r
0
0.4
a
r/a
n
0.8
1
1.5
1
0.5
500
1000
1500
RLDOS
normalized to
Rbulk
LDOS in SiO2
2000
[1] Schniepp & Sandoghdar, Phys. Rev. Lett 89 (2002)
[2] de Dood, Slooff, Polman, Moroz & van Blaaderen, Phys. Rev. A 64 (2001)

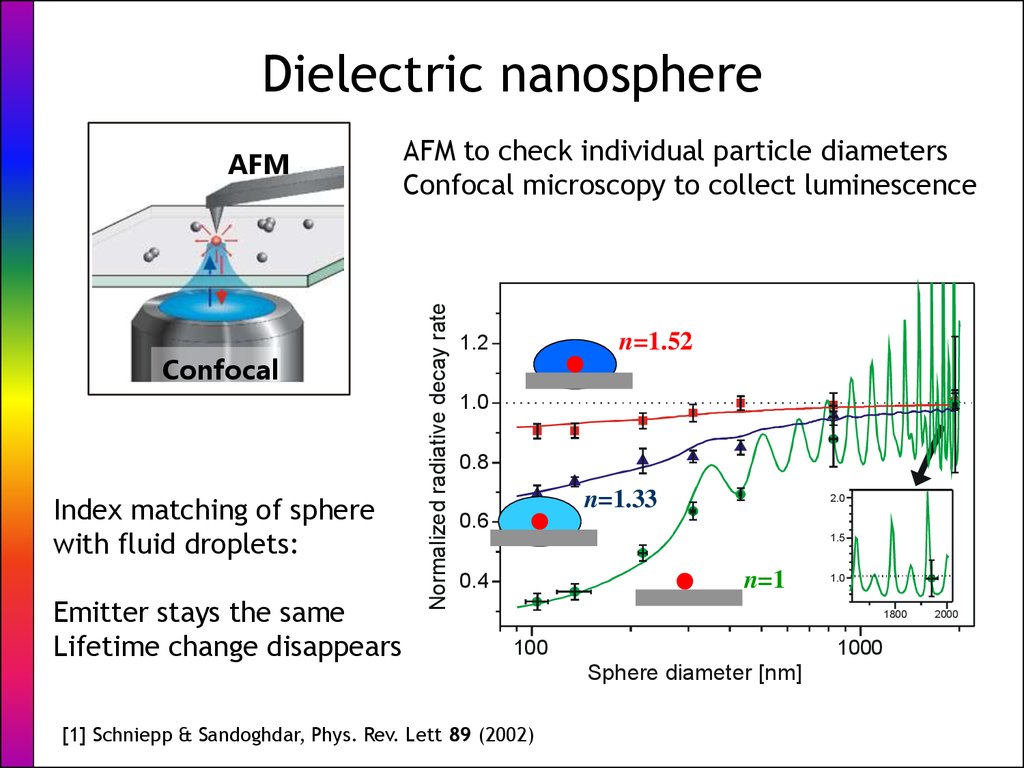
11. Dielectric nanosphere

AFM
Confocal
Index matching of sphere
with fluid droplets:
Emitter stays the same
Lifetime change disappears
AFM to check individual particle diameters
Confocal microscopy to collect luminescence
Normalized radiative decay rate
b)
n=1.52
1.2
1.0
0.8
n=1.33
2.0
0.6
1.5
n=1
0.4
1.0
1800
100
1000
Sphere diameter [nm]
[1] Schniepp & Sandoghdar, Phys. Rev. Lett 89 (2002)
2000

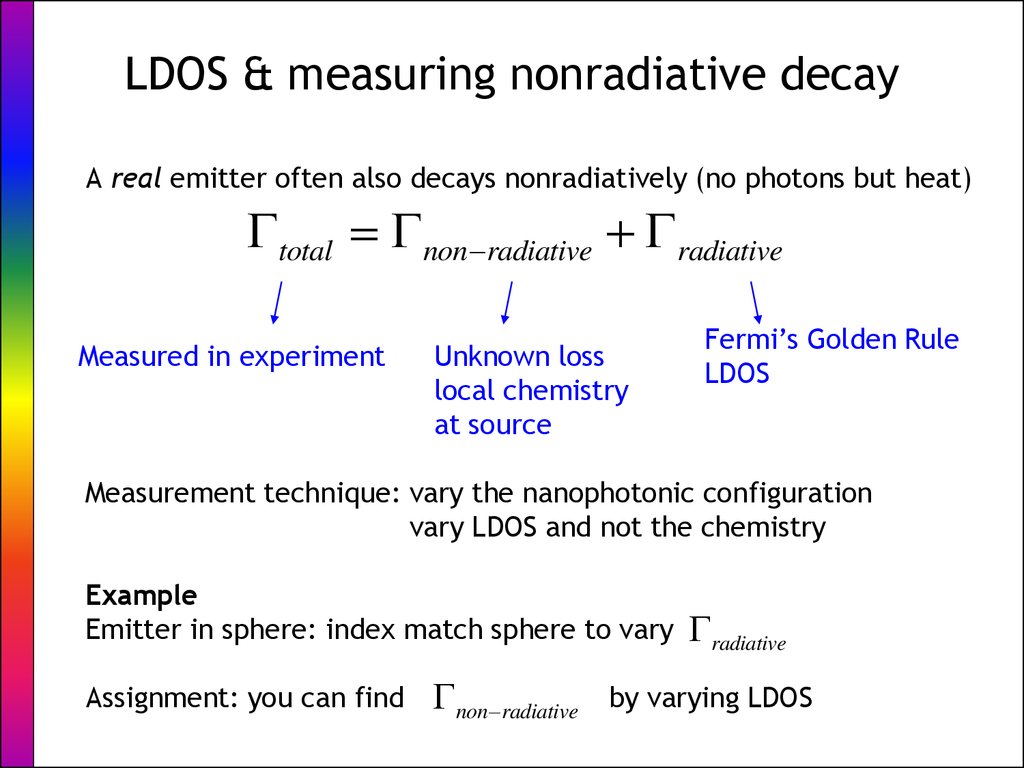
12. LDOS & measuring nonradiative decay

LDOS & measuring nonradiative decay
A real emitter often also decays nonradiatively (no photons but heat)
total non radiative radiative
Measured in experiment
Unknown loss
local chemistry
at source
Fermi’s Golden Rule
LDOS
Measurement technique: vary the nanophotonic configuration
vary LDOS and not the chemistry
Example
Emitter in sphere: index match sphere to vary
Assignment: you can find
non radiative
radiative
by varying LDOS

13. Conclusions

• Spontaneous emission rates are controlled by nanophotonic structures
• Fermi’s Golden Rule: transition rate depends on availability
of final states
• Spontaneous emission: final states for photon ?
• Density of states (DOS): number of photon states depending on frequency
• Local density of states (LDOS): number of photon states available
locally for spontaneous emission
Applications
• Enhance the efficiency of light sources
• Characterize non-radiative mechanisms