Draining Effect of a Tile Line

1. Draining Effect of a Tile Line

Made by : Ospanov Nursultan
Supervised by: Karlygash Alibayeva
Practice Supervisor: Koldas Asetzhan

2. Introduction

The class of problems involving flow of water through permeable media has a wide range and is of
considerable importance to engineers and scientists. The Armfield.Drainage and Seepage Tank, Model S1,
facilitates a detailed study of the movement of water through permeable media.
In the laboratory, we have the advantage of being able to use homogenius materials of known properties.
This simplifies the problem and makes it possible toreduce the number of components involved.
Following experiment has been designed to demonstrate the most typical situations that arise in dealing with
water as it moves through a permeable medium. The situations described are mostly "engineering"
situations. In addition to the water and the medium through which it moves, they usually involve some
artificial, or"engineering" element like a wall, a dam, a tile line etc.

3. Objective

Tile lines, horizontal galleries, trenches and, sometimes, vertical wells are widely
used to control seepage of water through permeable soils.
The objective of this experiment is to show, by visualising the flow lines, how a tile
line works (ie. how it forces the end-sections of flow lines to concentrate on one
point).

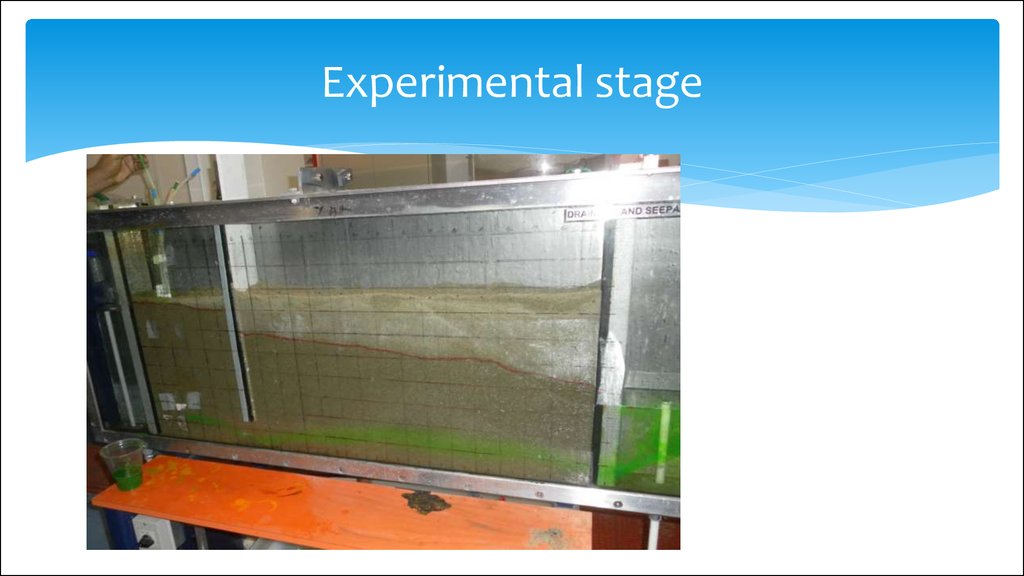
4. The Armfield.Drainage and Seepage Tank, Model S1

5. Tile drainage

Tile drainage is an agriculture practice that removes excess water from soil
subsurface.

6. Equipment Diagrams

1)sand tank
2) water inlet
3) clamp
4) impermeable baffle plate
5) adjustable clamp
6) incorporating six tapping points
7) two independently adjustable overflows (7 & 16)
8) A drain valve
9) the frame
10) adjustable feet
11) sump tank drain
12) sump tank
13) centrifugal pump
14) flow control valve
15) electrical switch

7. Equipment Diagrams

17) aluminium back panel
18) a shelf
19)toughened glass

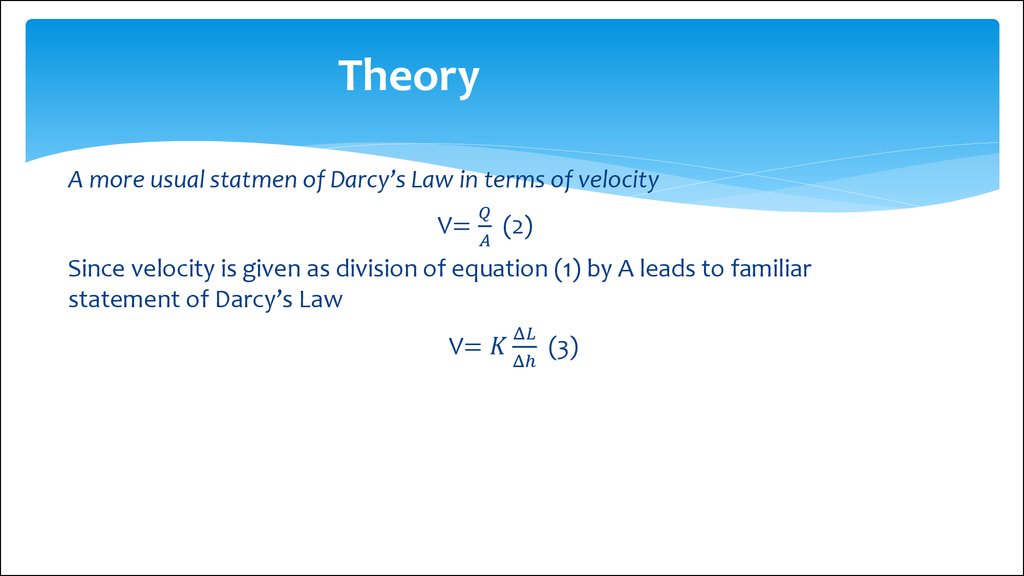
8. Theory

Darcy’s Law
The flow rate through porous media is proportional to head loss and
inversely proportional length of the flow path
Darcy’s Law can be expressed mathmatically as: