Similar presentations:
DE
1.
DIFFERENTIAL EQUATIONS &MATHEMATICAL ANALYSIS
Kiniabaeva Aisha
Vovchik
2.
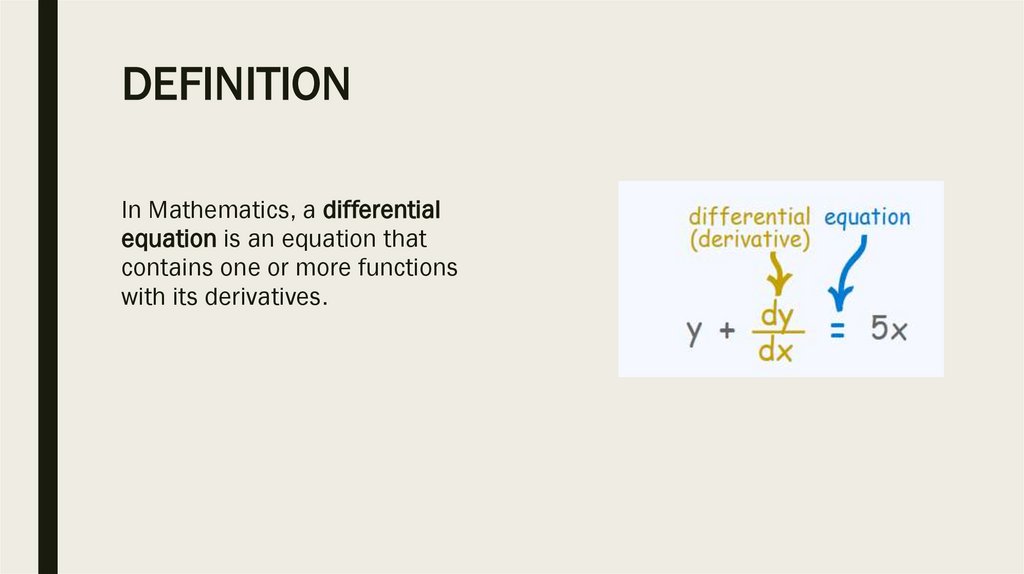
DEFINITIONIn Mathematics, a differential
equation is an equation that
contains one or more functions
with its derivatives.
3.
ORDER AND DEGREE OF DIFFERENTIALEQUATION
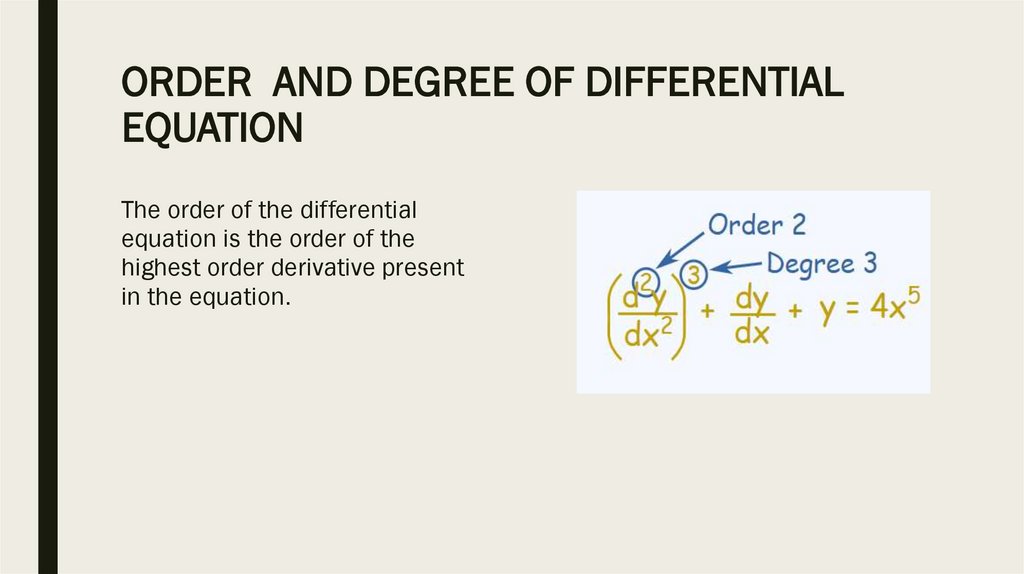
The order of the differential
equation is the order of the
highest order derivative present
in the equation.
4.
ExamplesFirst Order Differential
Equation
Second-Order Differential
Equation
All the linear equations in the form of
derivatives are in the first order. It has
only the first derivative such as dy/dx,
where x and y are the two variables
The equation which includes the
second-order derivative is the secondorder differential equation.
5.
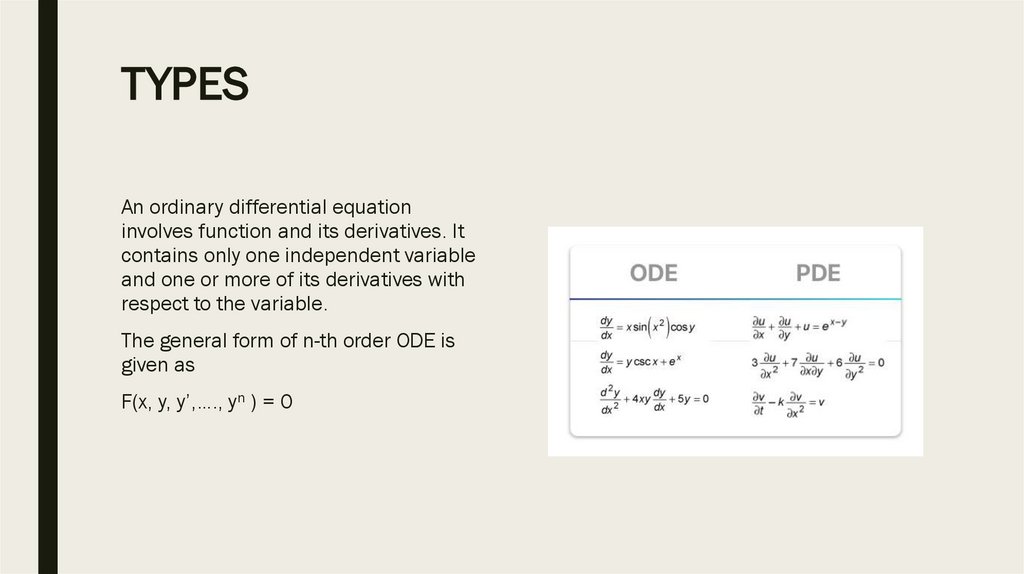
TYPESAn ordinary differential equation
involves function and its derivatives. It
contains only one independent variable
and one or more of its derivatives with
respect to the variable.
The general form of n-th order ODE is
given as
F(x, y, y’,…., yn ) = 0
6.
Applications■ 1) Differential equations describe various exponential
growths and decays.
■ 2) They are also used to describe the change in return on
investment over time.
■ 3) They are used in the field of medical science for modelling
cancer growth or the spread of disease in the body.
■ 4) Movement of electricity can also be described with the
help of it.
■ 5) They help economists in finding optimum investment
strategies.
■ 6) The motion of waves or a pendulum can also be described
using these equations.
7.
Math ModeMathematical modeling is the process of representing real-world
situations through mathematical concepts and structures in order to
analyze and solve problems. This involves creating mathematical
equations, inequalities, graphs, and simulations that capture the
essential features of a phenomenon or system.
8.
PurposeThe primary goals of mathematical modeling include understanding a
system, predicting its future behavior, optimizing certain outcomes, and
simulating different scenarios to assess potential results.
9.
Types of Models■
Descriptive Models: These models describe the characteristics of a system without
making predictions.
■
Predictive Models: These models can predict future states or outputs based on
current data and relationships.
■
Prescriptive Models: These models suggest actions to achieve specific goals, often
used in optimization scenarios.
10.
ApplicationMathematical modeling is widely used in various fields, such as
engineering, physics, biology, economics, and social sciences, to
understand complex systems, optimize processes, and make informed
decisions.
11.
ExampleMathMode in dota 2
Purpose: calculate the ttk with
MIDNIGHT PULSE
Difference between game and real life
– the TIME.
12.
Equation (model)The legend:
h – health
t – time
r - health regen
Δt – time difference
k - % dmg
13.
Solving process14.
Exponential equationr = 3.8
h = 626
k = 0.1