Explore Internal resistance and EMF practise questions

1.

Questions
The E ect of Internal Resistance on Practical Circuits
1.
2.
Define:
a)
Electromotive Force (emf)
b)
Potential Di erence (p.d.)
c)
Terminal Potential Di erence
A battery has an emf of 6.0V and an internal resistance of 2Ω. It is connected to a 15Ω, as shown.
a)
Calculate the current flowing in the circuit.
b)
Calculate the terminal potential di erence across the battery.

2.

Questions Continued
2.
Continued
c)
What is the value of the “lost volts”?
d)
The 15Ω resistor is removed and the battery is short-circuited by connecting a think copper wire across the
terminals. Calculate the short circuit current.
3.
A battery has an emf of 12.0V and an internal resistance of 1.5Ω. It is connected to a 3Ω resistor, in the circuit shown.
a)
Calculate the reading on the ammeter
b)
Calculate the reading on the voltmeter
c)
The 3Ω resistor is replaced with a 1.5Ω resistor. Calculate the new readings on the ammeter and voltmeter.

3.

Questions Continued
4.
A voltmeter is connected across a cell and reads 1.5V. The reading on the voltmeter drops to 1.0V when the cell is
connected to a 20Ω resistor. Find the internal resistance of the cell.
5.
A high voltage power supply delivers an emf of 600V and has an internal resistance of 3Ω. It is connected to a 40Ω
resistor.
a)
Calculate the current flowing in the circuit.
b)
The 40Ω resistor is removed and the power supply is short-circuited. Calculate the short-circuit current.
c)
Suggest why some high voltage power supplies intentionally have a large internal resistance fitted.

4.

Questions Continued
6.
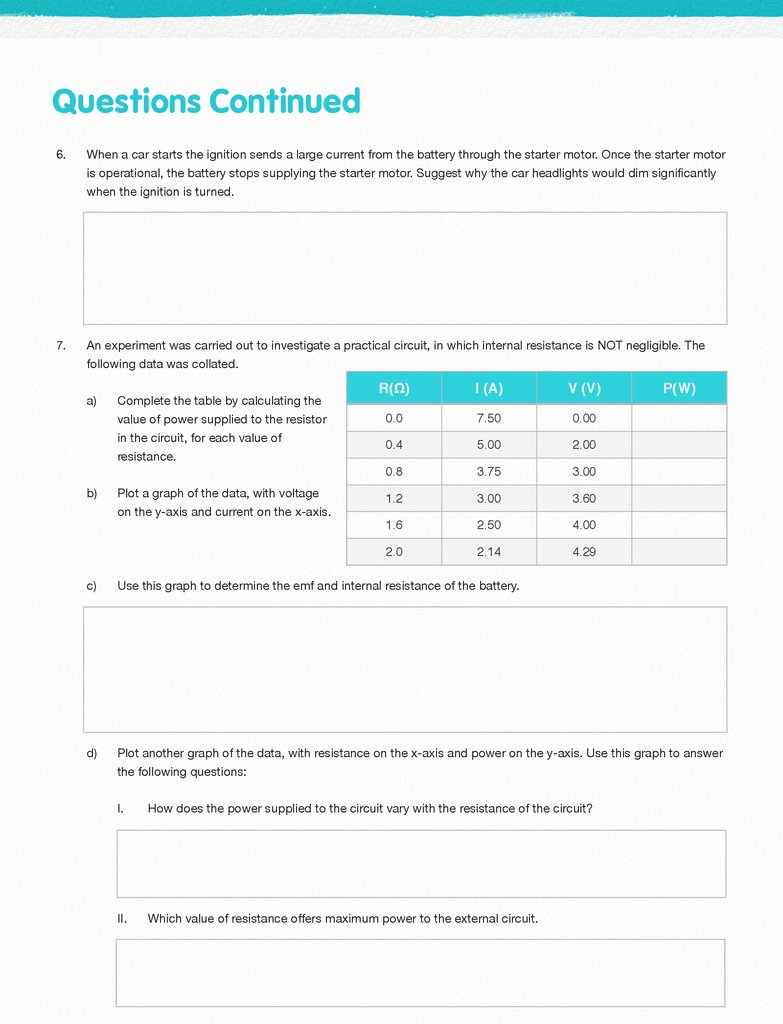
When a car starts the ignition sends a large current from the battery through the starter motor. Once the starter motor
is operational, the battery stops supplying the starter motor. Suggest why the car headlights would dim significantly
when the ignition is turned.
7.
An experiment was carried out to investigate a practical circuit, in which internal resistance is NOT negligible. The
following data was collated.
a)
Complete the table by calculating the
value of power supplied to the resistor
in the circuit, for each value of
resistance.
b)
Plot a graph of the data, with voltage
on the y-axis and current on the x-axis.
R(Ω)
I (A)
V (V)
0.0
7.50
0.00
0.4
5.00
2.00
0.8
3.75
3.00
1.2
3.00
3.60
1.6
2.50
4.00
2.0
2.14
4.29
P(W)
c)
Use this graph to determine the emf and internal resistance of the battery.
d)
Plot another graph of the data, with resistance on the x-axis and power on the y-axis. Use this graph to answer
the following questions:
I.
How does the power supplied to the circuit vary with the resistance of the circuit?
II.
Which value of resistance o ers maximum power to the external circuit.