Determinants, their properties. Minors and cofactors. Lecture 2

1.

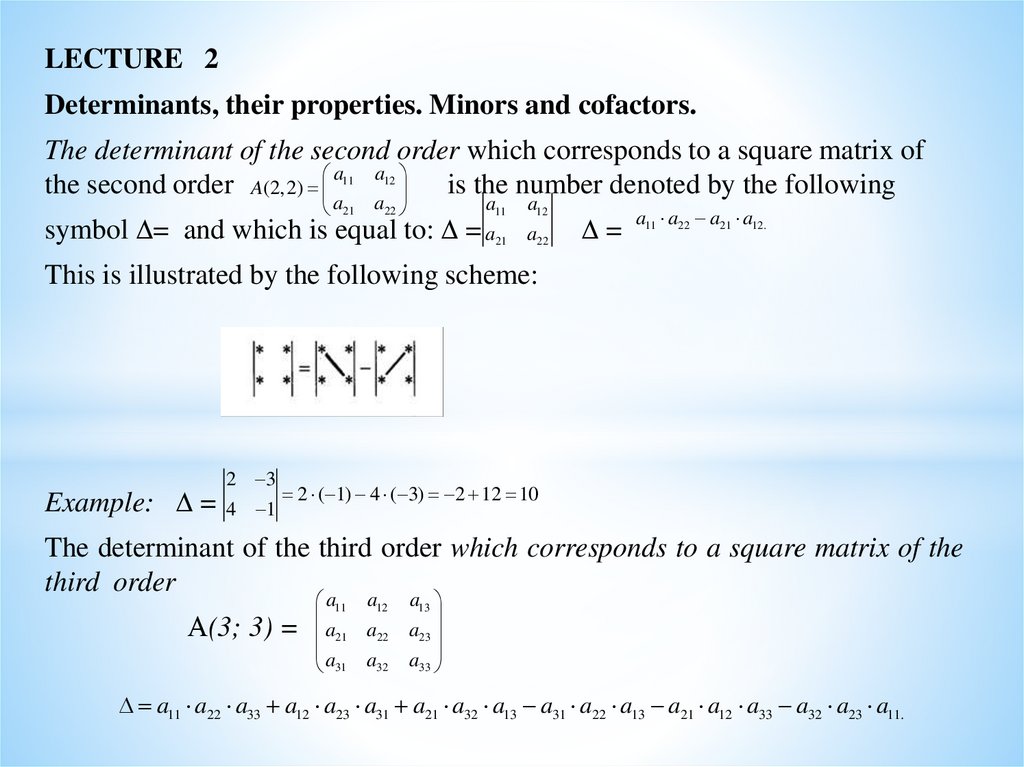
LECTURE 2
Determinants, their properties. Minors and cofactors.
The determinant of the second order which corresponds to a square matrix of
the second order A(2,2) a11 a12 is the number denoted by the following
a21 a22
a11
symbol = and which is equal to: = a21
a12
a22
=
a11 a22 a21 a12.
This is illustrated by the following scheme:
Example: =
2 3
4 1
2 ( 1) 4 ( 3) 2 12 10
The determinant of the third order which corresponds to a square matrix of the
third order
А(3; 3) =
a11 a12
a
21 a22
a
31 a32
a13
a23
a33
a11 a22 a33 a12 a23 a31 a21 a32 a13 a31 a22 a13 a21 a12 a33 a32 a23 a11.

2.

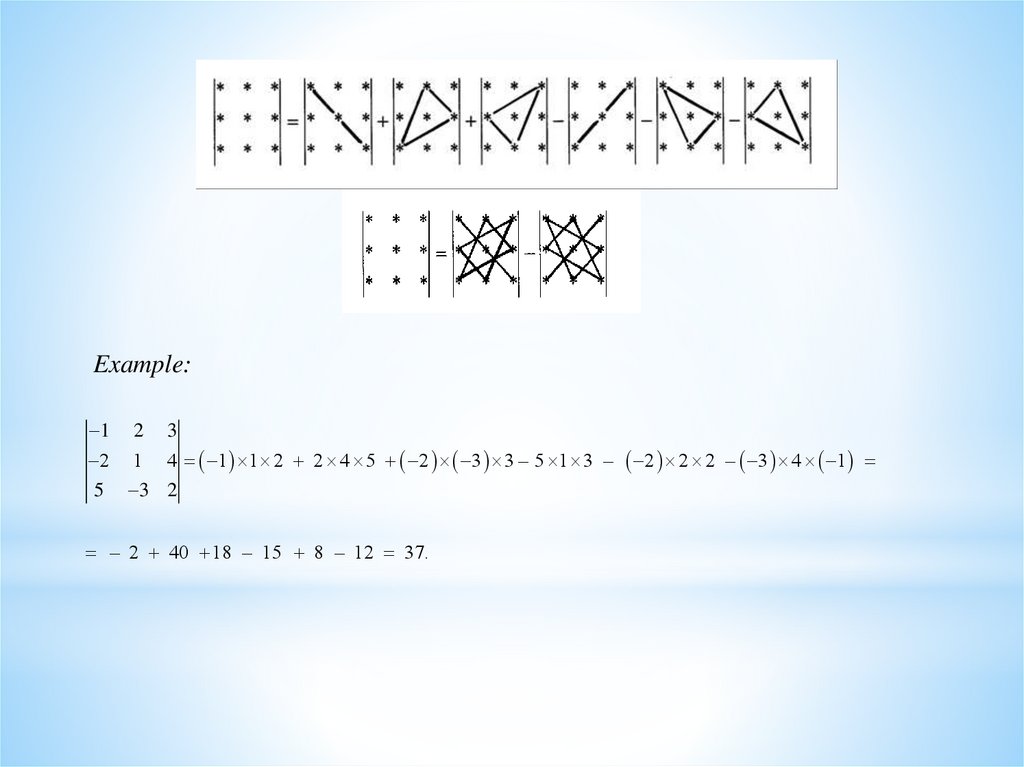
Example:
1
2
3
2
1
4 1 1 2 2 4 5 2 3 3 – 5 1 3 –
5
3 2
– 2 40 18 – 15 8 – 12 37.
2 2 2 – 3 4 1

3.

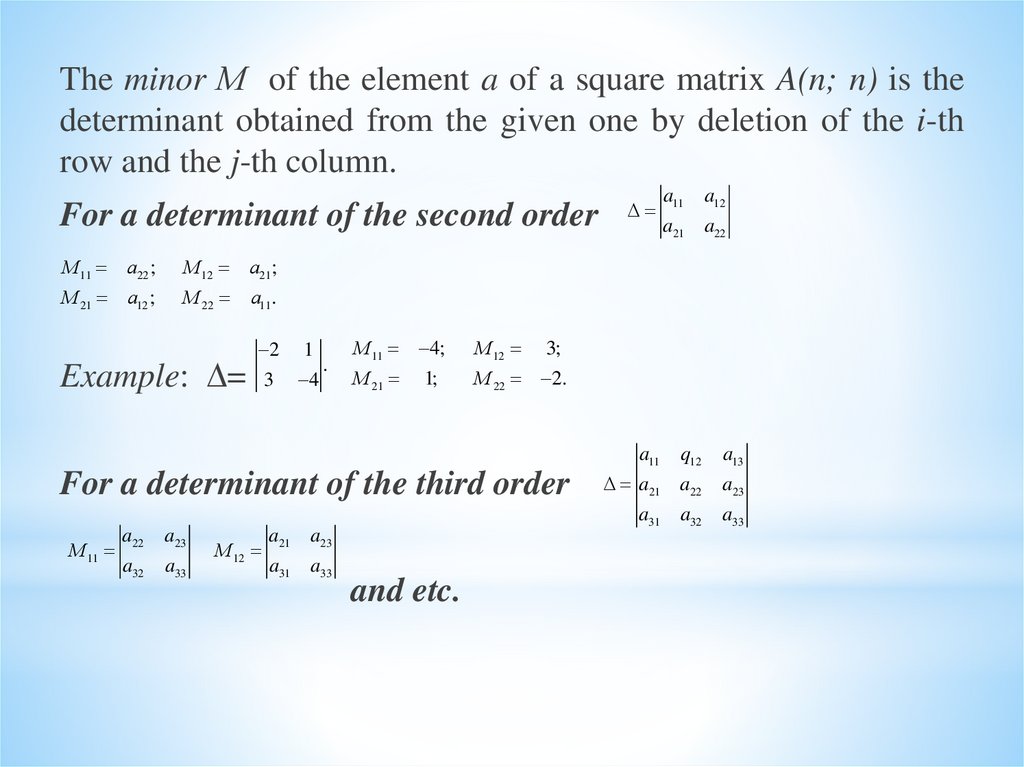
The minor М of the element a of a square matrix A(n; n) is the
determinant obtained from the given one by deletion of the i-th
row and the j-th column.
For a determinant of the second order
М11 а22 ;
М12 а21;
М 21 а12 ;
М 22
Example: =
2
1
3
4
.
М 11 4;
М 12
a23
a32
a33
a21 a22
М 21
М 22 2.
1;
3;
For a determinant of the third order
a22
a12
а11.
a11
М 11
a11
М 12
a21 a23
a31
a33
and etc.
q12
a13
a21 a22
a23
a31
a33
a32

4.

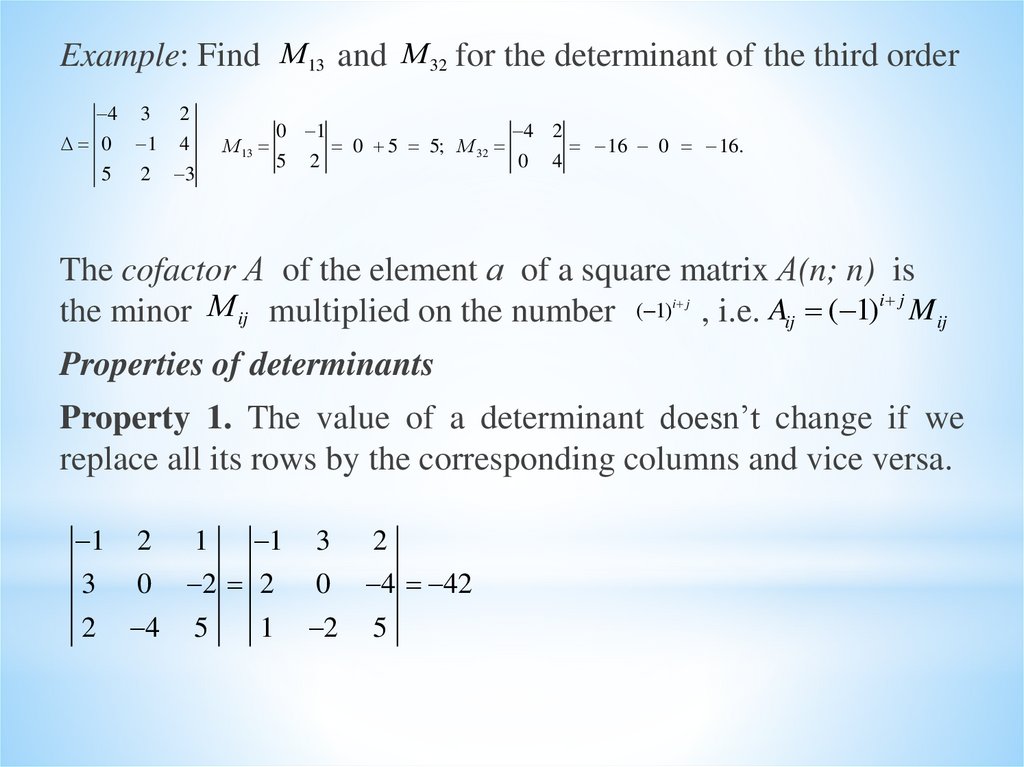
Example: Find М 13 and М 32 for the determinant of the third order
4
0
5
3
2
1
4
2
3
М 13
0 1
5
2
0 5 5; М 32
4 2
0
4
16 0 16.
The cofactor А of the element а of a square matrix А(n; n) is
the minor М ij multiplied on the number ( 1) , i.e. Aij ( 1)i j M ij
i j
Properties of determinants
Property 1. The value of a determinant doesn’t change if we
replace all its rows by the corresponding columns and vice versa.
1
2
1
3
0
2
4
1
3
2
2 2
0
4 42
5
2
5
1

5.

Property 2. If we replace any two neighbouring rows (columns)
then the determinant will change a sign.
1 0
1 0
1
1
1 0
1
1
0 1
2
1 3 4
5
0
2
1 3 3 1
2
4
5
1 3
4
5
4
0
2
0
0
5
Property 3. If all the elements of a row (column) of a
determinant are multiplied on the same non-zero number «m»
then the determinant value increases (decreases) in «m» times.
Example:
1
1 2
3
1
7
Corollary 1. If all the elements of a row (column) of a
determinant have a non-zero common multiplier, it can be taken
out the determinant sign.
3
5
4
3
1
0
6 2 1
2
1 8
2
5
2
0
3
1 4

6.

Corollary 2. A determinant at which elements of two arbitrary rows (columns)
are proportional respectively is equal to zero.
1 4
2
2
0 4 0
3
1 6
Property 4. Let each element of an arbitrary row (column) be the sum of two
addends. Then the determinant is equal to the sum of two determinants such
that the corresponding row (column) of the first determinant consists of the
first addends, and the corresponding row (column) of the second determinant
consists of the second addends.
Example: For example, let the first column of the determinant be the sum of
two addends. Then
1 2 3
4
1 3
4
2
3
4
1 3
0 2 1
0 2 3 0 2 .
4 5
7 3
7 3
4
5
7 3

7.

Theorem 1. If all the elements of an arbitrary i–th row (either j–th column) of
a determinant but aij are equal to zero, the determinant is equal to the product
of this non-zero element aij on its cofactor Aij, i.e. = aij Aij.
Theorem 2. A determinant is equal to the sum of products of elements of an
arbitrary row (column) on their cofactors. This operation is called a
decomposition of a determinant on a row (column).
Notion of a determinant of the n-th order
The determinant of the n–th order corresponding to a square matrix of the n–th
order A(n; n) is the number which is equal to the sum of products of elements
of an arbitrary row (column) on its cofactors, i.e.
a11
a12
... a1 j
... a1n
a21
a22 ... a2 j
... a2 n
...
...
...
...
...
ai1
ai 2
...
aij
... ain
...
...
...
...
...
an1 an 2 ... anj
...
...
... ann
ai1 Ai1 ai 2 Ai 2 ... aij Aij ... ain Ain