How to use tables of tolerances and fundamental deviations (Seminar 3)

1. Seminar 3: How to use tables of tolerances and fundamental deviations

Standardization and measurement
assurance of engineering production

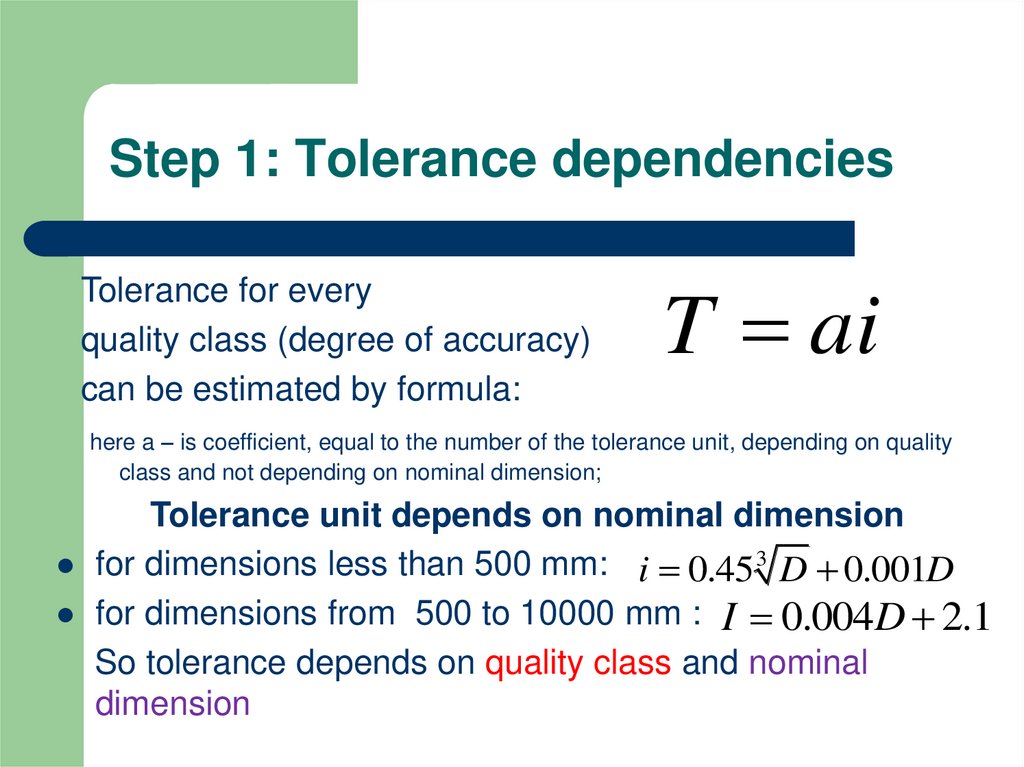
2. Step 1: Tolerance dependencies

Tolerance for every
quality class (degree of accuracy)
can be estimated by formula:
T ai
here a – is coefficient, equal to the number of the tolerance unit, depending on quality
class and not depending on nominal dimension;
Tolerance unit depends on nominal dimension
for dimensions less than 500 mm: i 0.45 3 D 0.001D
for dimensions from 500 to 10000 mm : I 0.004D 2.1
So tolerance depends on quality class and nominal
dimension

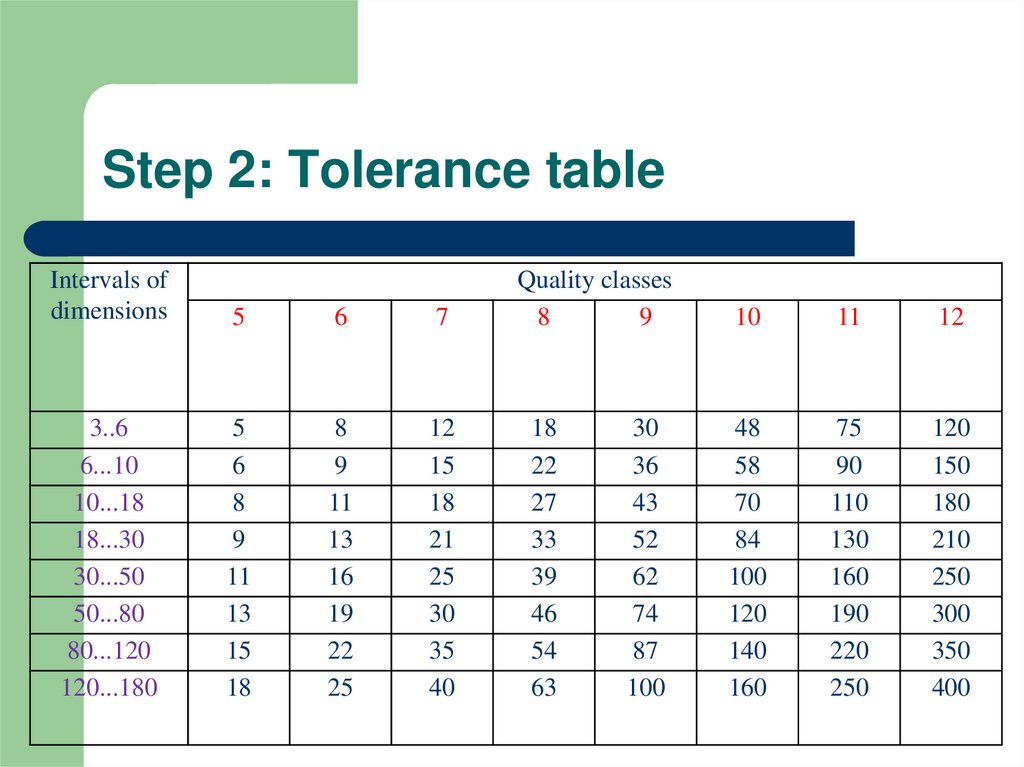
3. Step 2: Tolerance table

Intervals of
dimensions
5
6
7
3..6
6...10
10...18
18...30
30...50
50...80
80...120
120...180
5
6
8
9
11
13
15
18
8
9
11
13
16
19
22
25
12
15
18
21
25
30
35
40
Quality classes
8
9
18
22
27
33
39
46
54
63
30
36
43
52
62
74
87
100
10
11
12
48
58
70
84
100
120
140
160
75
90
110
130
160
190
220
250
120
150
180
210
250
300
350
400

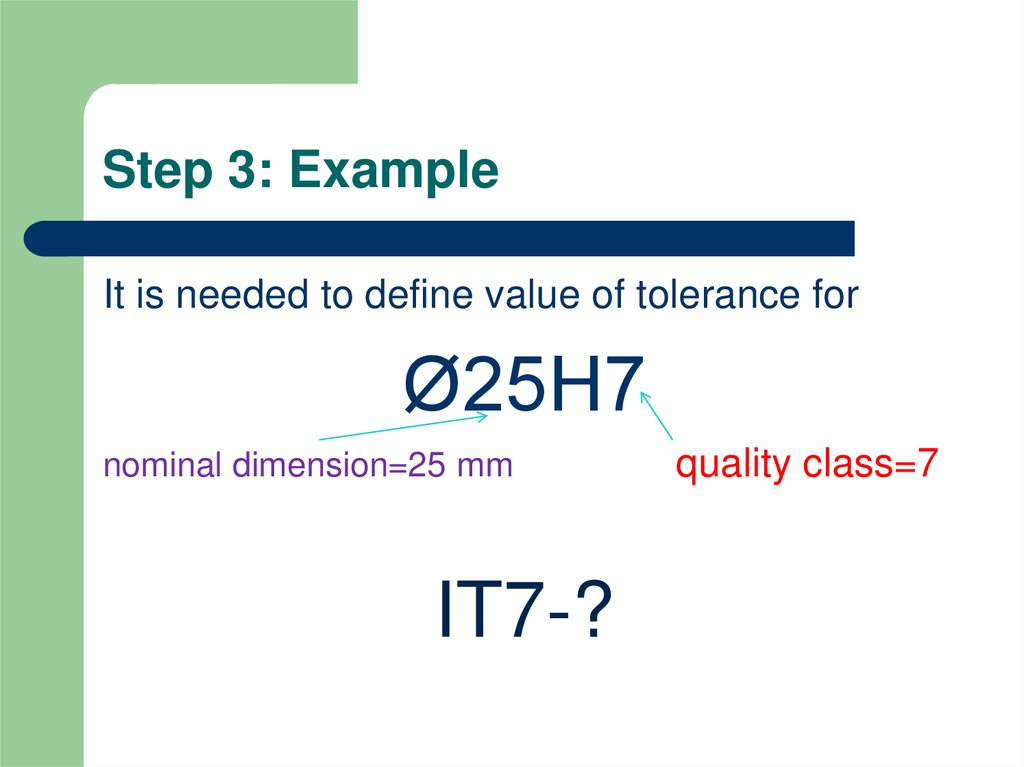
4. Step 3: Example

It is needed to define value of tolerance for
Ø25H7
nominal dimension=25 mm
IT7-?
quality class=7

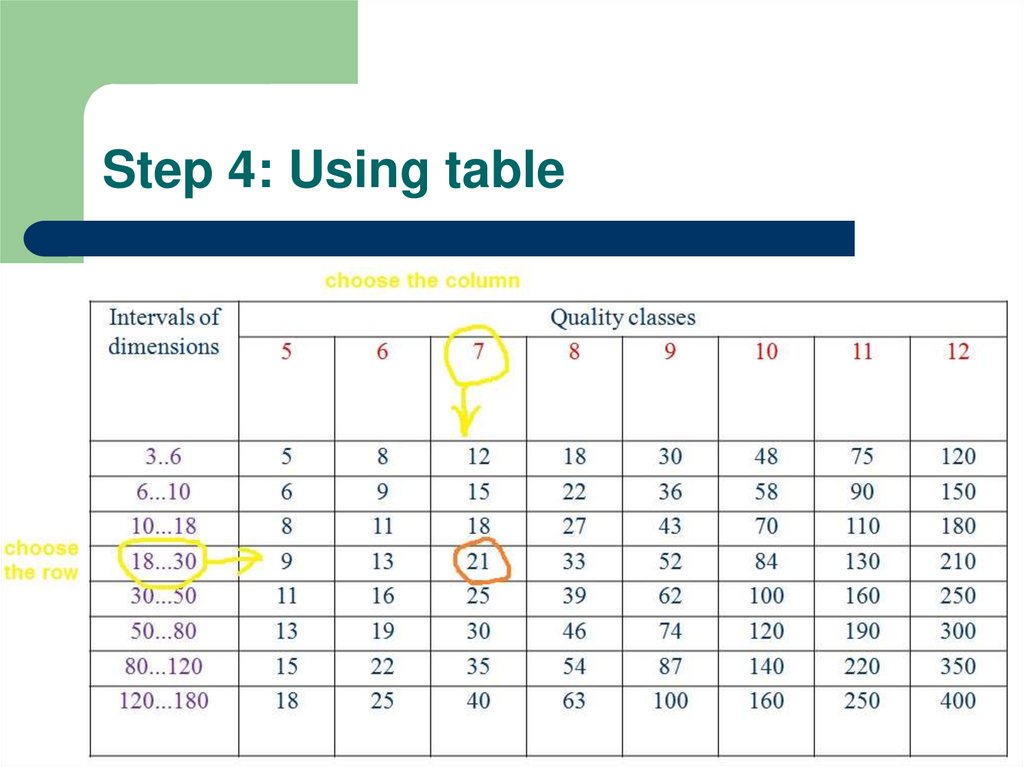
5. Step 4: Using table

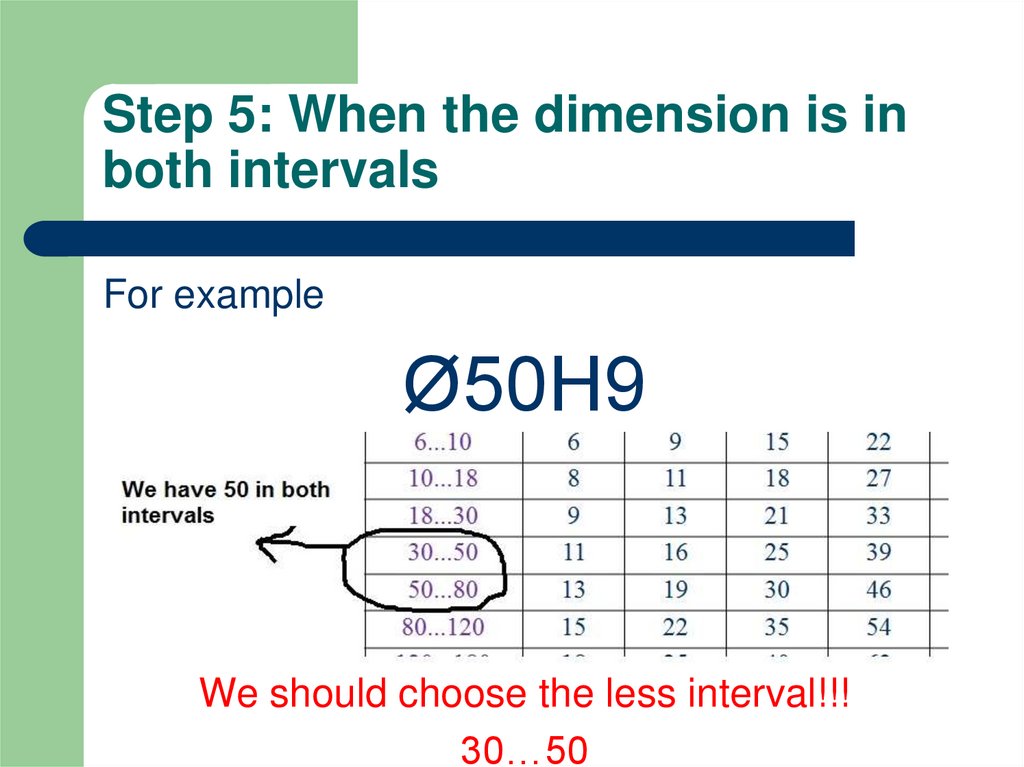
6. Step 5: When the dimension is in both intervals

For example
Ø50H9
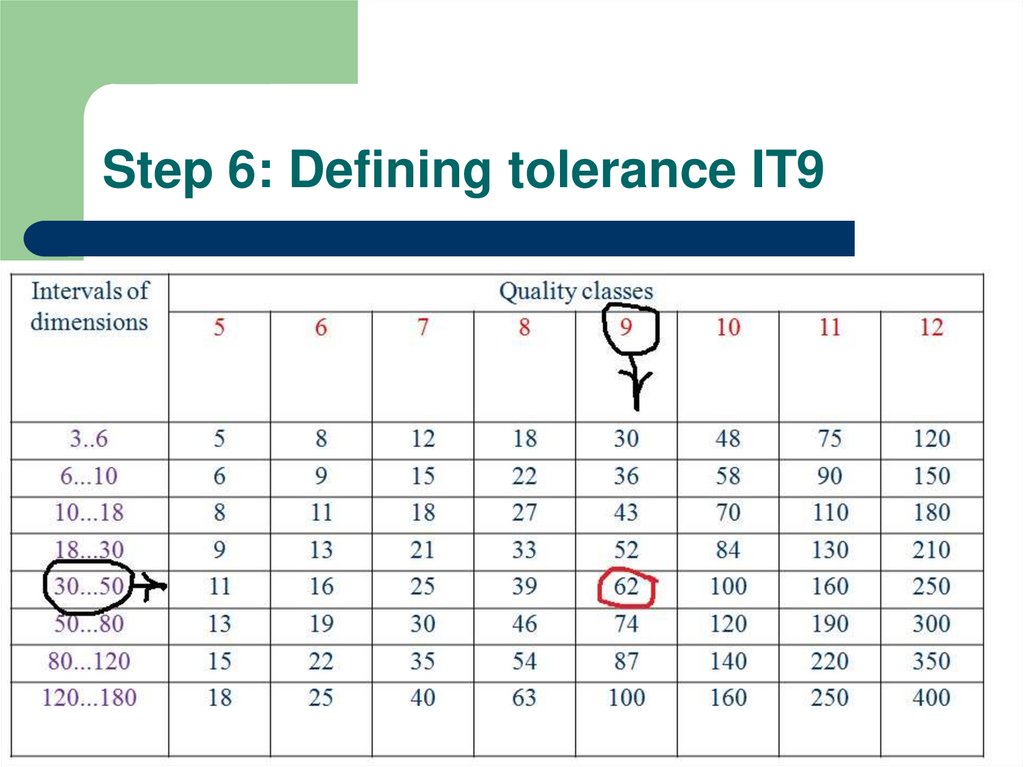
We should choose the less interval!!!
30…50

7. Step 6: Defining tolerance IT9

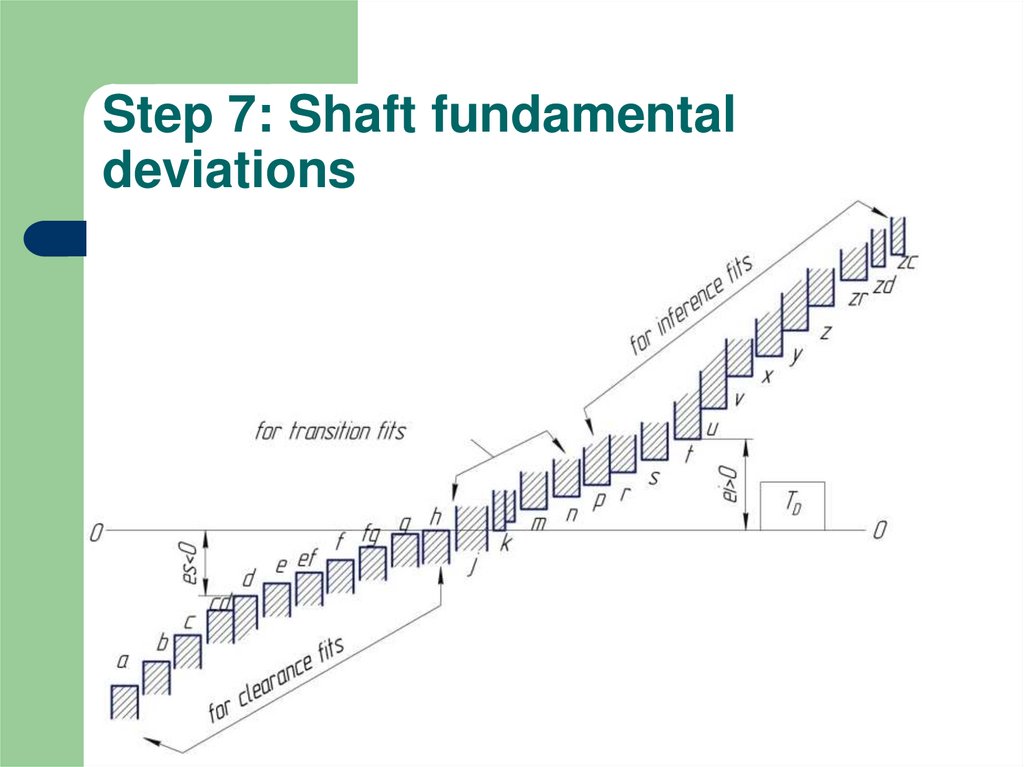
8. Step 7: Shaft fundamental deviations

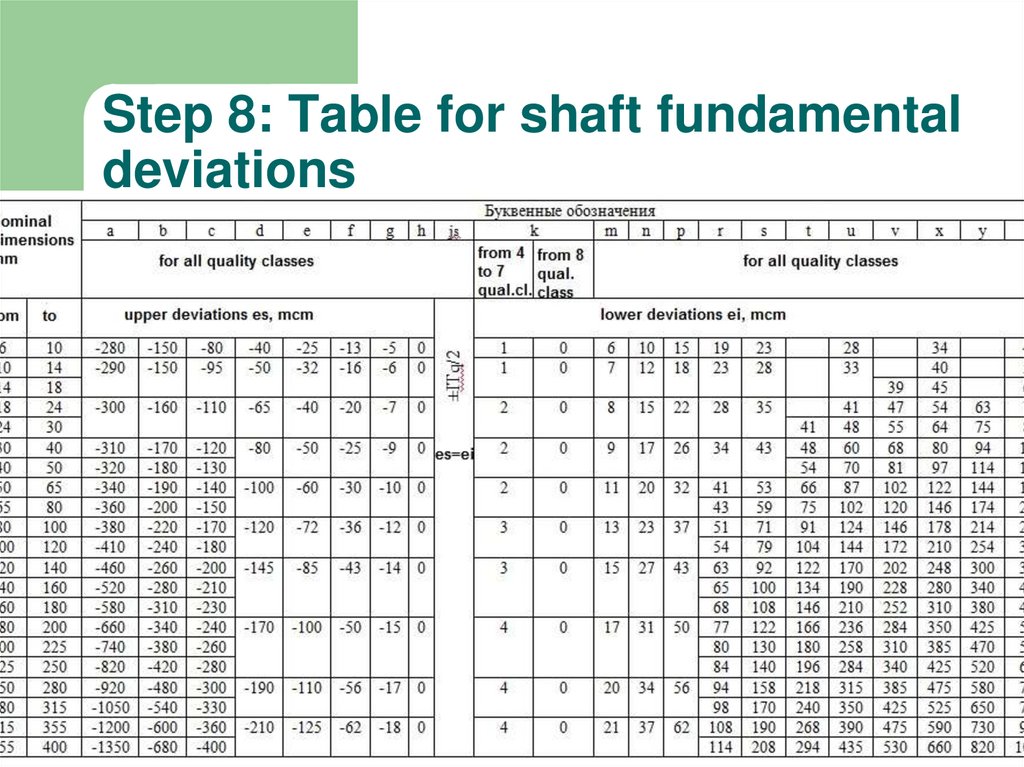
9. Step 8: Table for shaft fundamental deviations

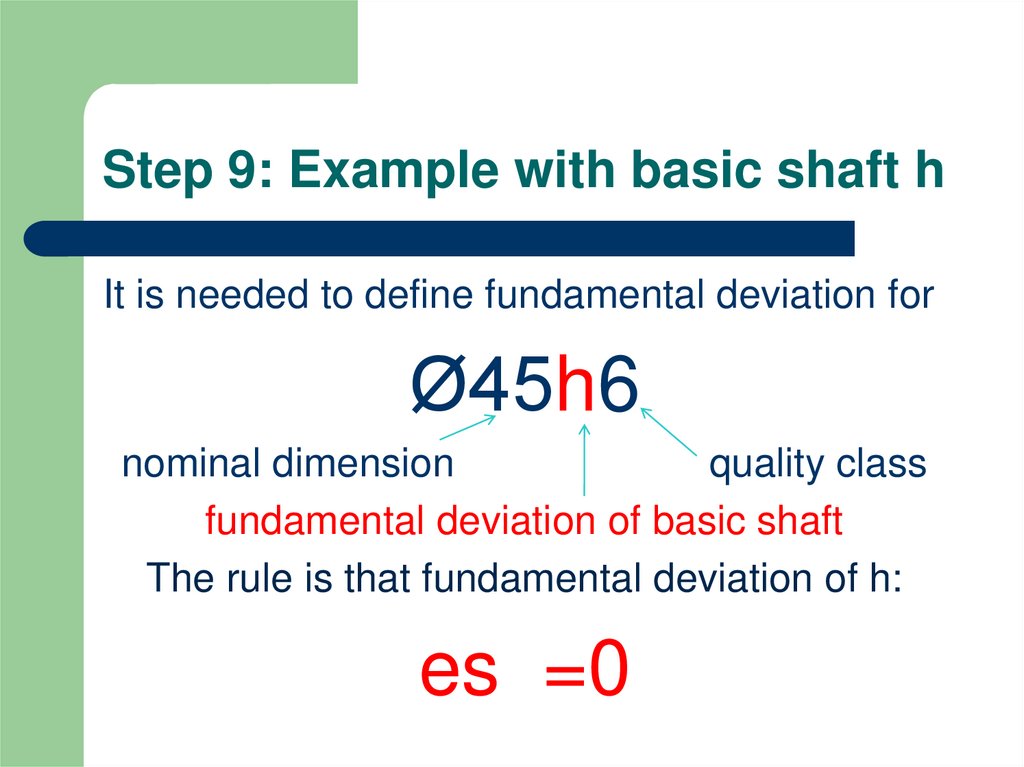
10. Step 9: Example with basic shaft h

It is needed to define fundamental deviation for
Ø45h6
nominal dimension
quality class
fundamental deviation of basic shaft
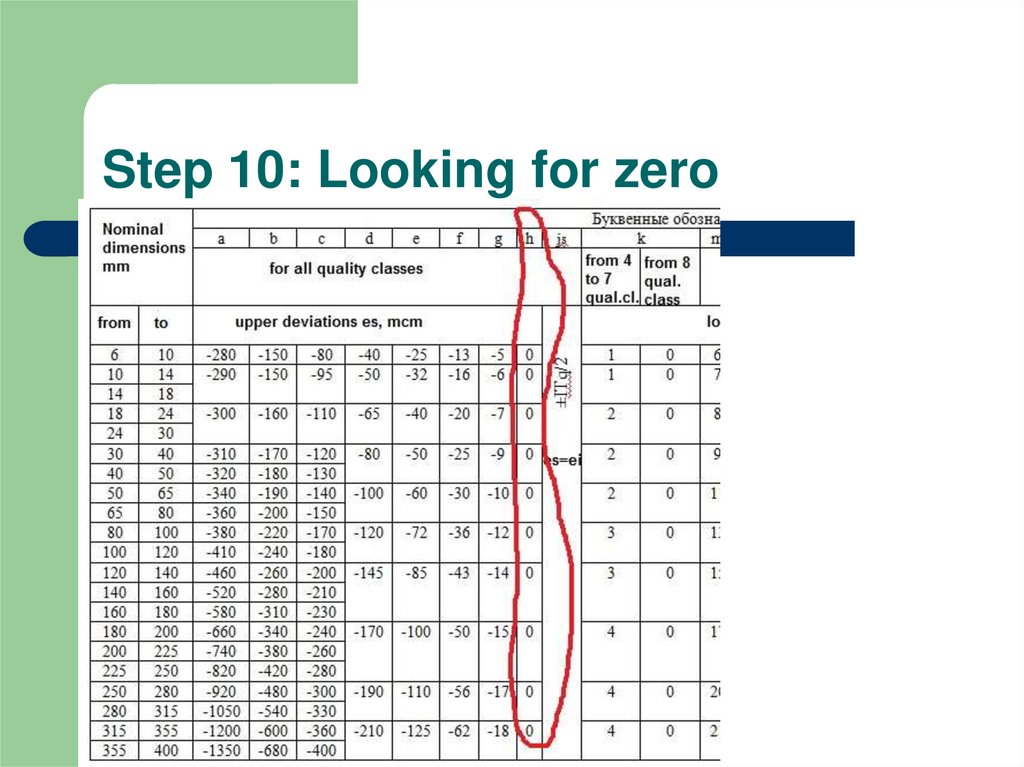
The rule is that fundamental deviation of h:
es =0

11. Step 10: Looking for zero

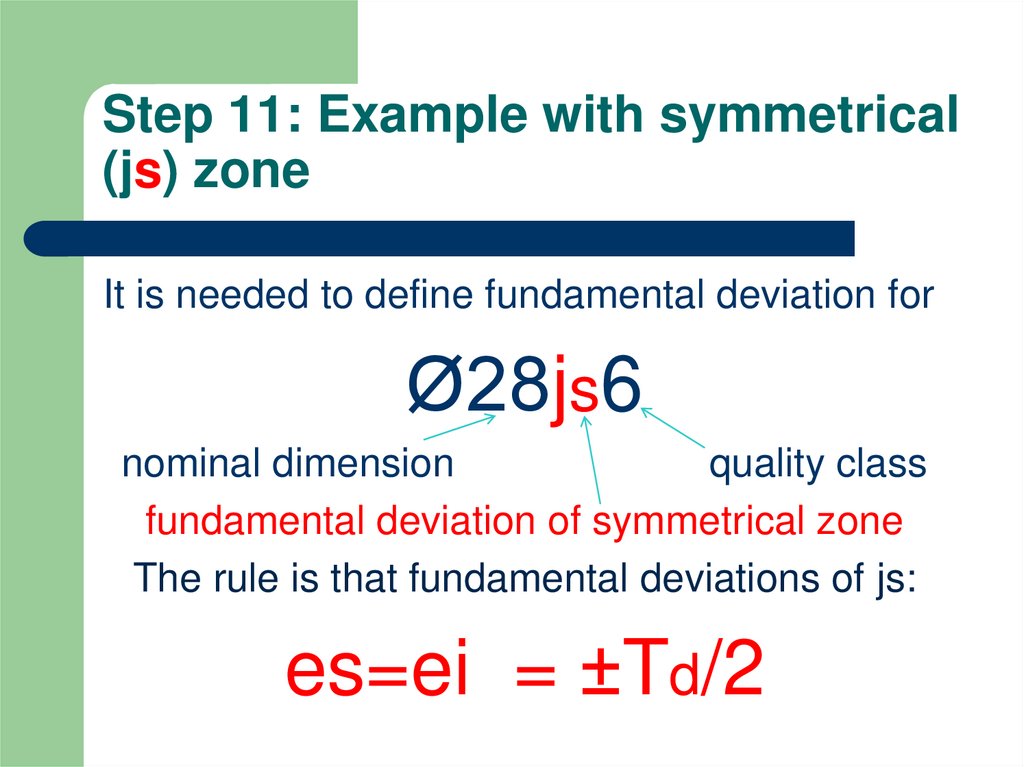
12. Step 11: Example with symmetrical (js) zone

It is needed to define fundamental deviation for
Ø28js6
nominal dimension
quality class
fundamental deviation of symmetrical zone
The rule is that fundamental deviations of js:
es=ei = ±Td/2

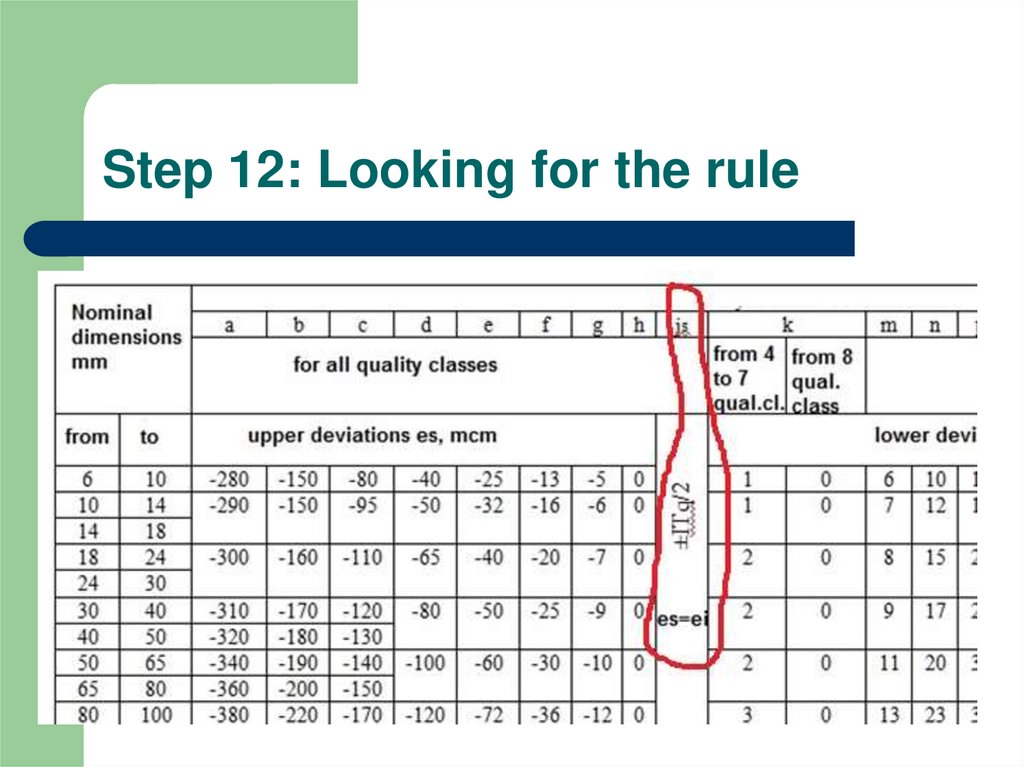
13. Step 12: Looking for the rule

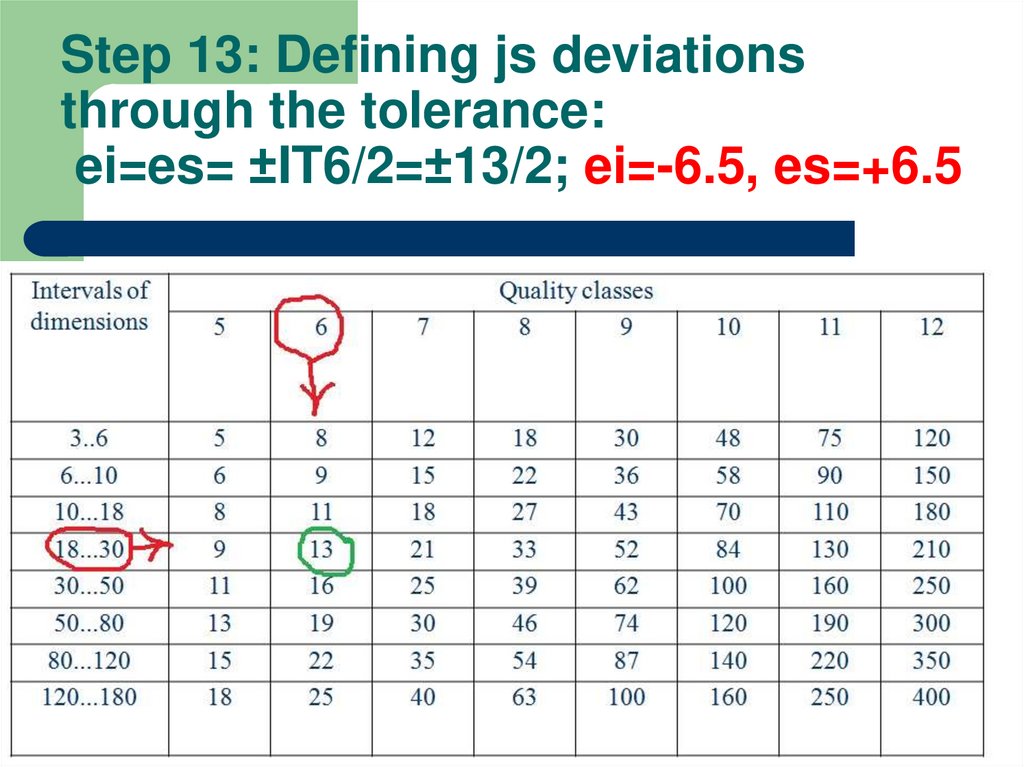
14. Step 13: Defining js deviations through the tolerance: ei=es= ±IT6/2=±13/2; ei=-6.5, es=+6.5

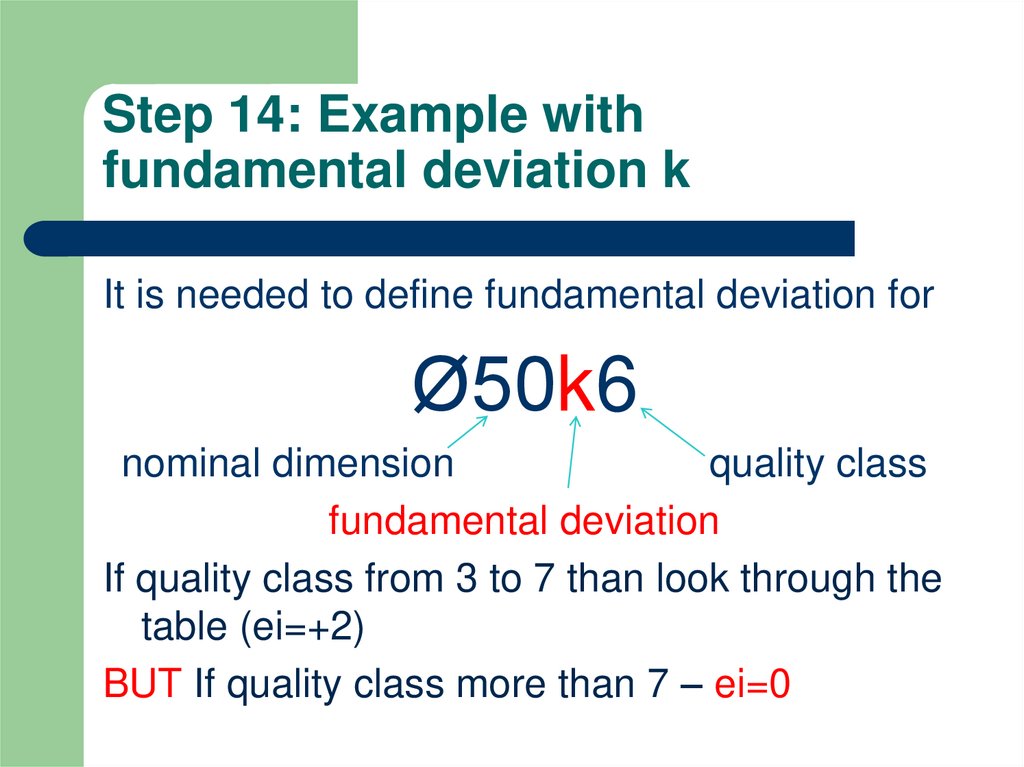
15. Step 14: Example with fundamental deviation k

It is needed to define fundamental deviation for
Ø50k6
nominal dimension
quality class
fundamental deviation
If quality class from 3 to 7 than look through the
table (ei=+2)
BUT If quality class more than 7 – ei=0

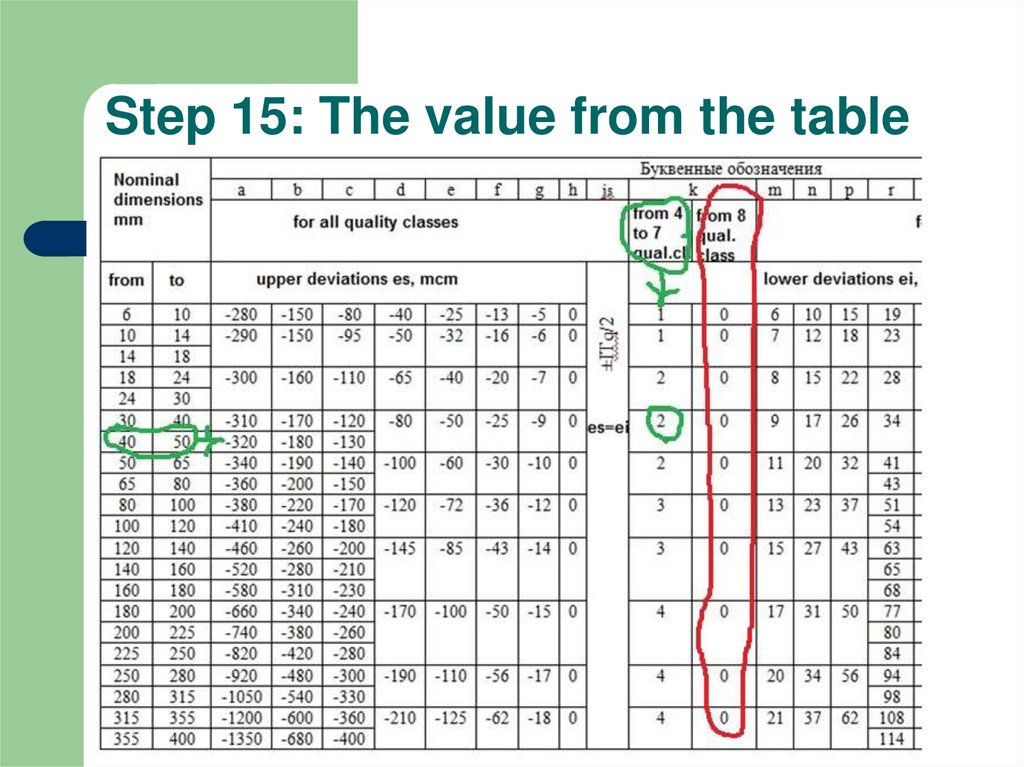
16. Step 15: The value from the table

17. Step 16: Find the values

Ø55h8
Ø80js10
Ø100k9
Ø125p6
Ø10a5
You should find both: fundamental deviation
and tolerance