Similar presentations:
Criterii de congruentă a triunghiurilor
1.
2.
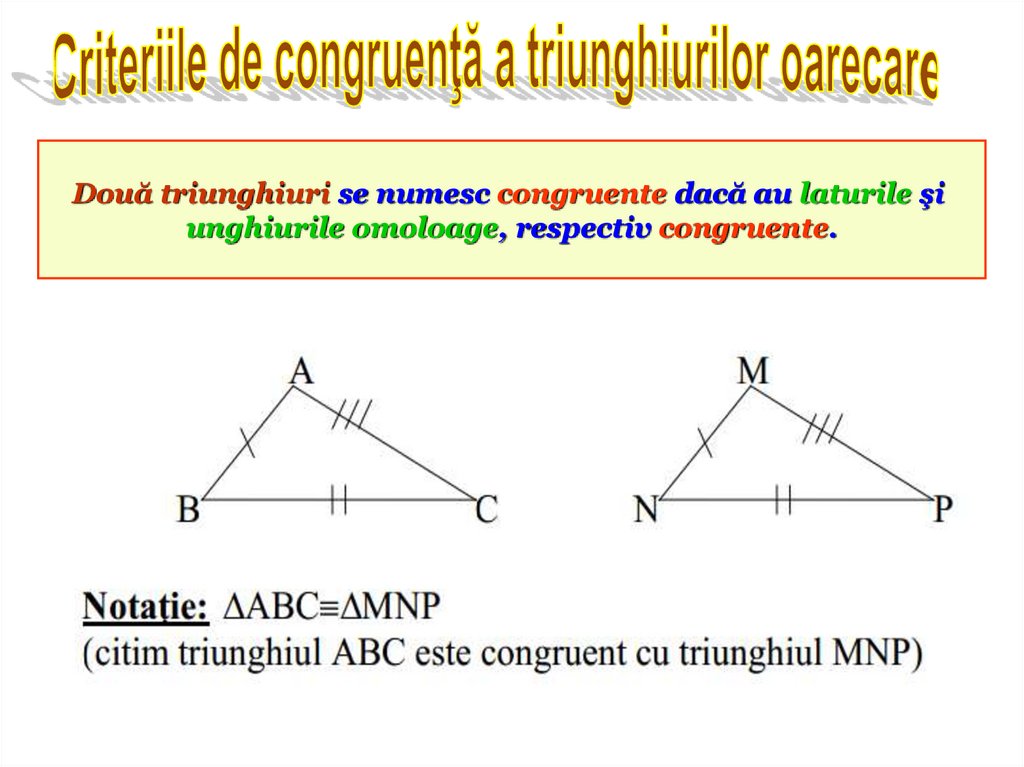
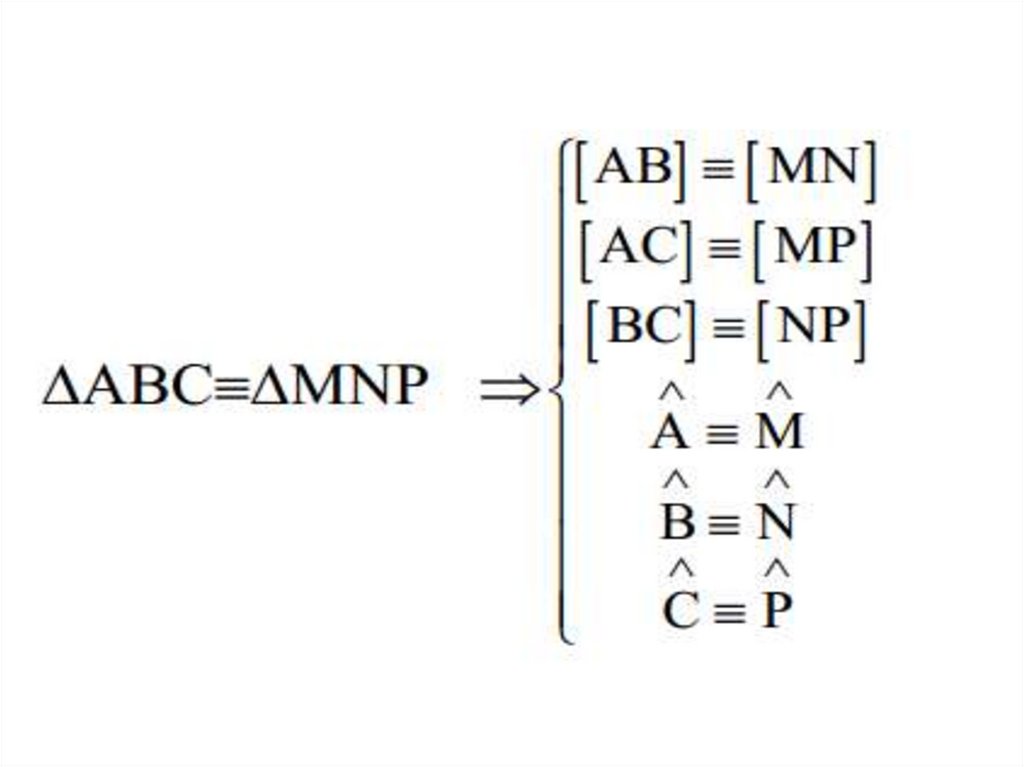
Două triunghiuri se numesc congruente dacă au laturile şiunghiurile omoloage, respectiv congruente.
3.
4.
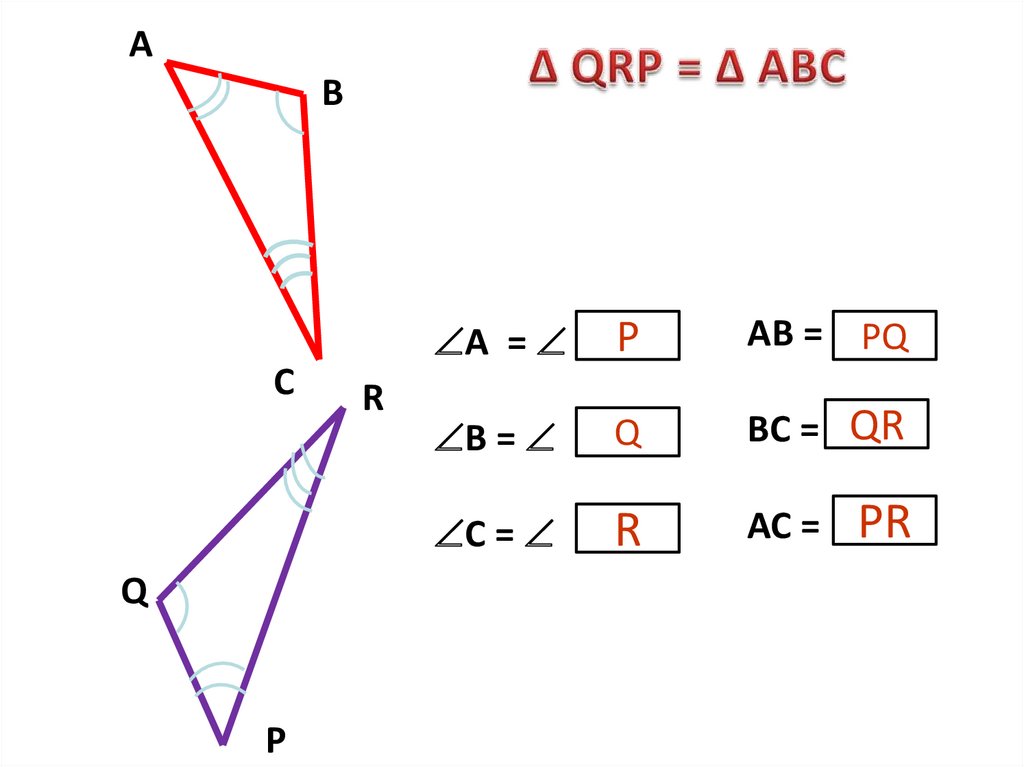
АВ
С
Q
P
R
А =
P
АВ = PQ
В =
Q
ВС = QR
С =
R
АС =
PR
5.
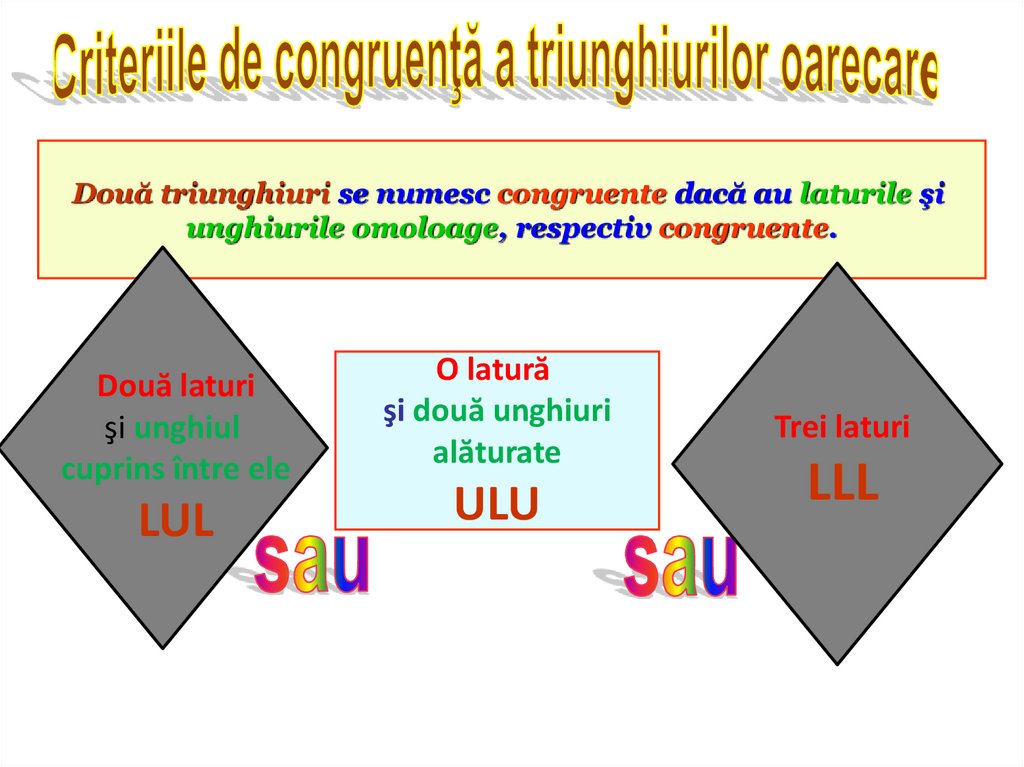
Două triunghiuri se numesc congruente dacă au laturile şiunghiurile omoloage, respectiv congruente.
Două laturi
şi unghiul
cuprins între ele
LUL
O latură
şi două unghiuri
alăturate
ULU
Trei laturi
LLL
6.
7.
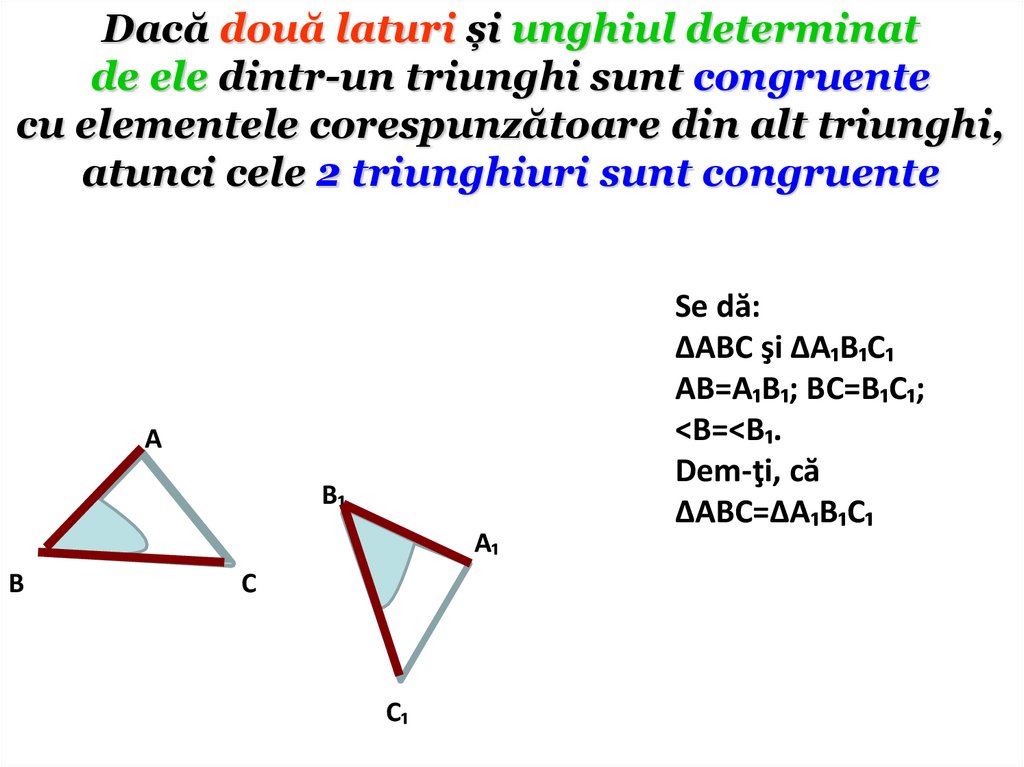
Dacă două laturi și unghiul determinatde ele dintr-un triunghi sunt congruente
cu elementele corespunzătoare din alt triunghi,
atunci cele 2 triunghiuri sunt congruente
А
В₁
А₁
В
С
С₁
Se dă:
∆АВС şi ∆А₁В₁С₁
АВ=А₁В₁; ВС=В₁С₁;
<В=<В₁.
Dem-ţi, că
∆АВС=∆А₁В₁С₁
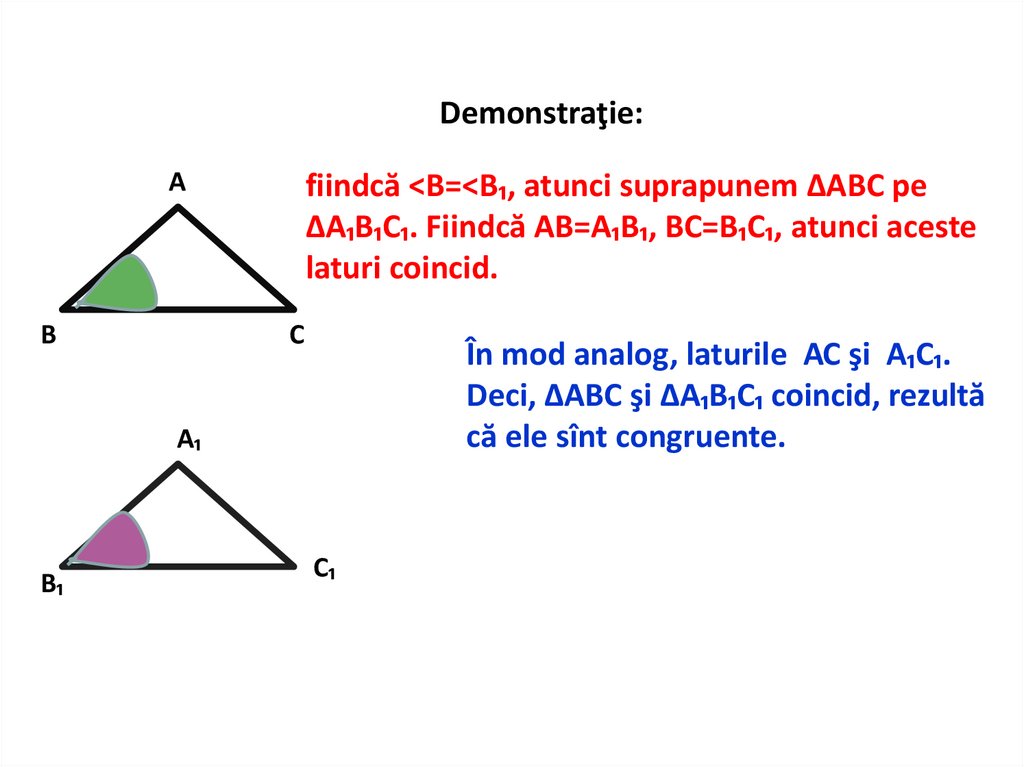
8.
Demonstraţie:А
В
fiindcă <В=<В₁, atunci suprapunem ∆АВС pe
∆А₁В₁С₁. Fiindcă АВ=А₁В₁, ВС=В₁С₁, atunci aceste
laturi coincid.
С
În mod analog, laturile АС şi А₁С₁.
Deci, ∆АВС şi ∆А₁В₁С₁ coincid, rezultă
că ele sînt congruente.
А₁
В₁
С₁
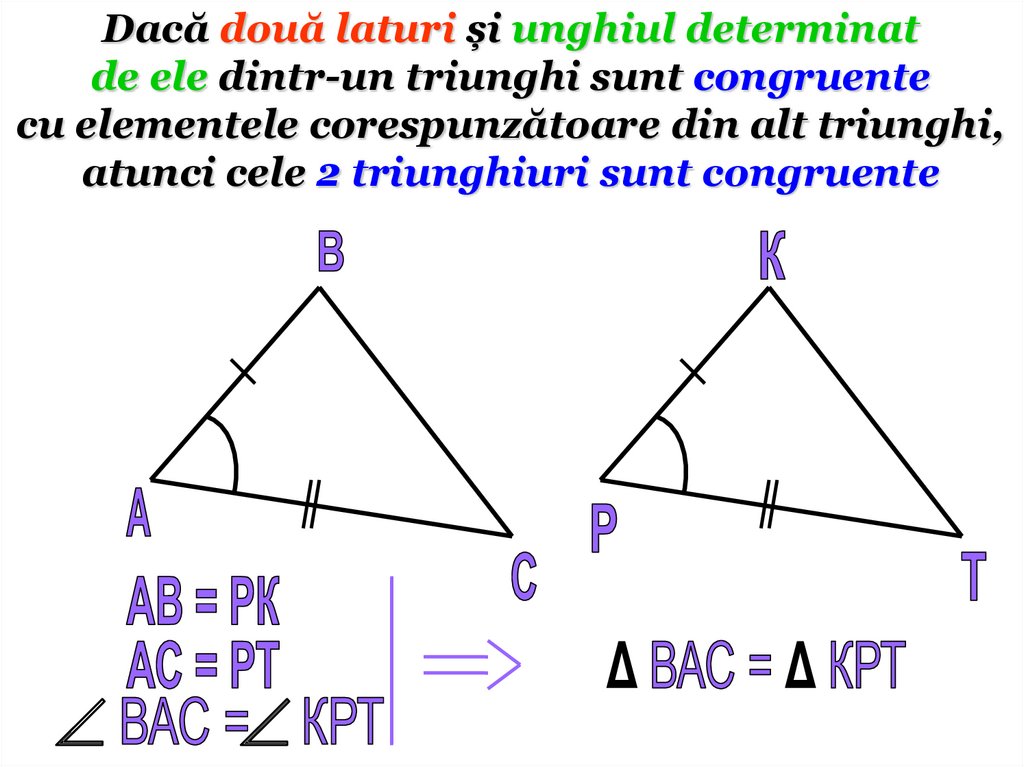
9.
Dacă două laturi și unghiul determinatde ele dintr-un triunghi sunt congruente
cu elementele corespunzătoare din alt triunghi,
atunci cele 2 triunghiuri sunt congruente
10.
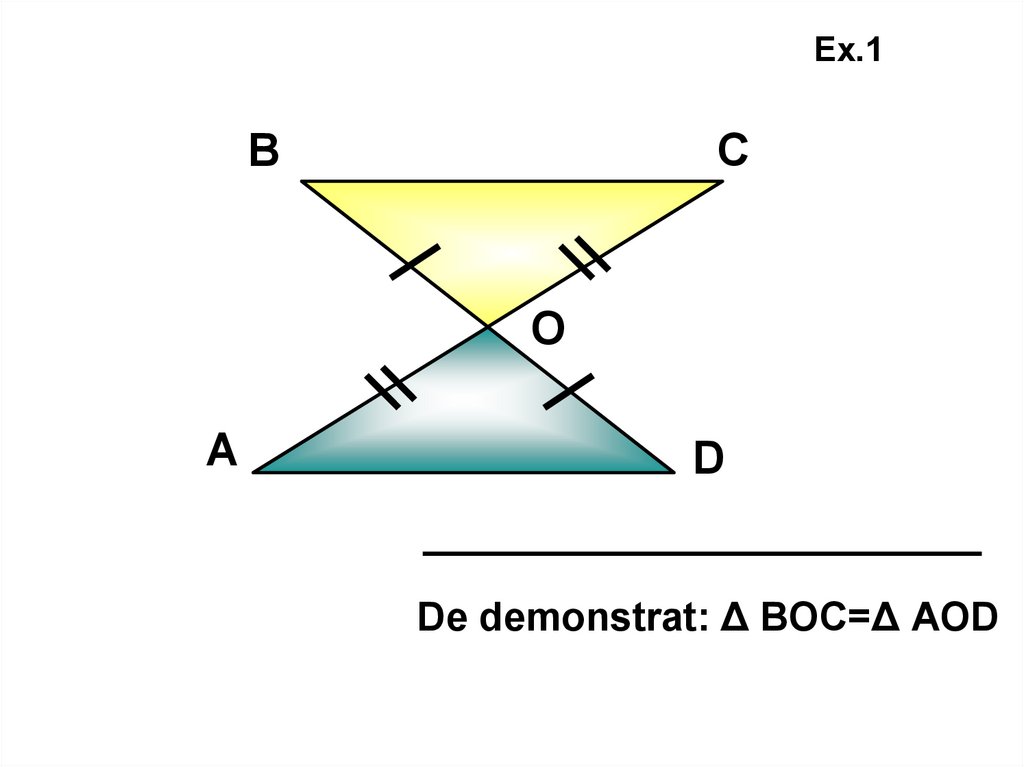
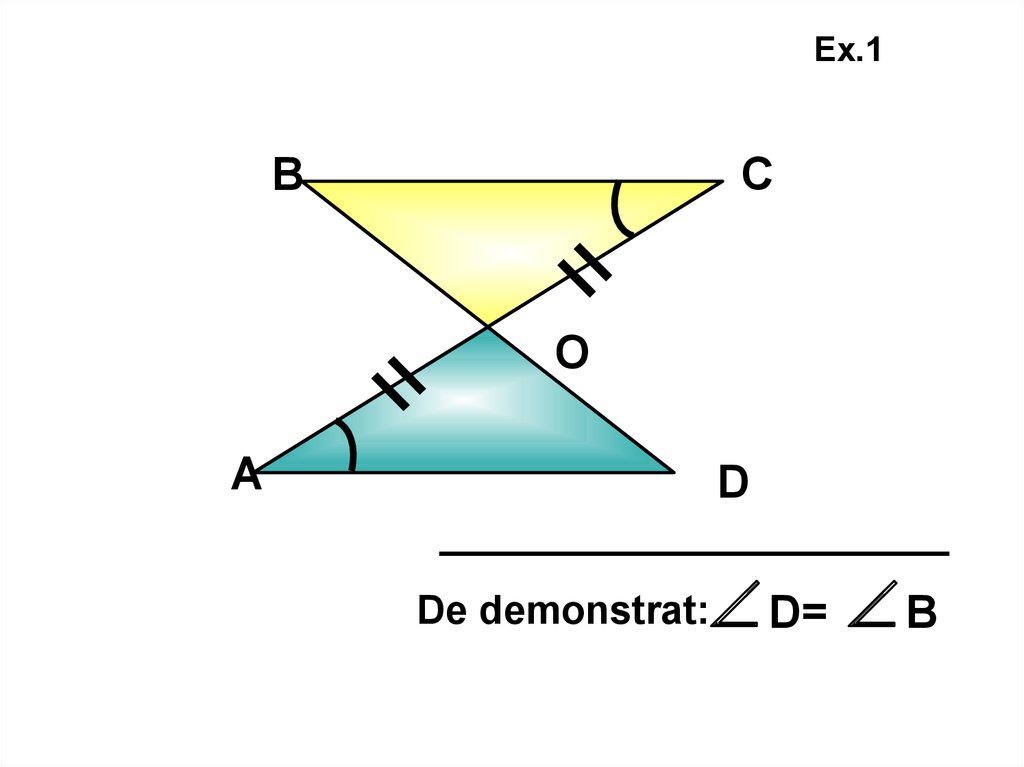
Ex.1В
С
О
А
D
De demonstrat: Δ ВОС=Δ АОD
11.
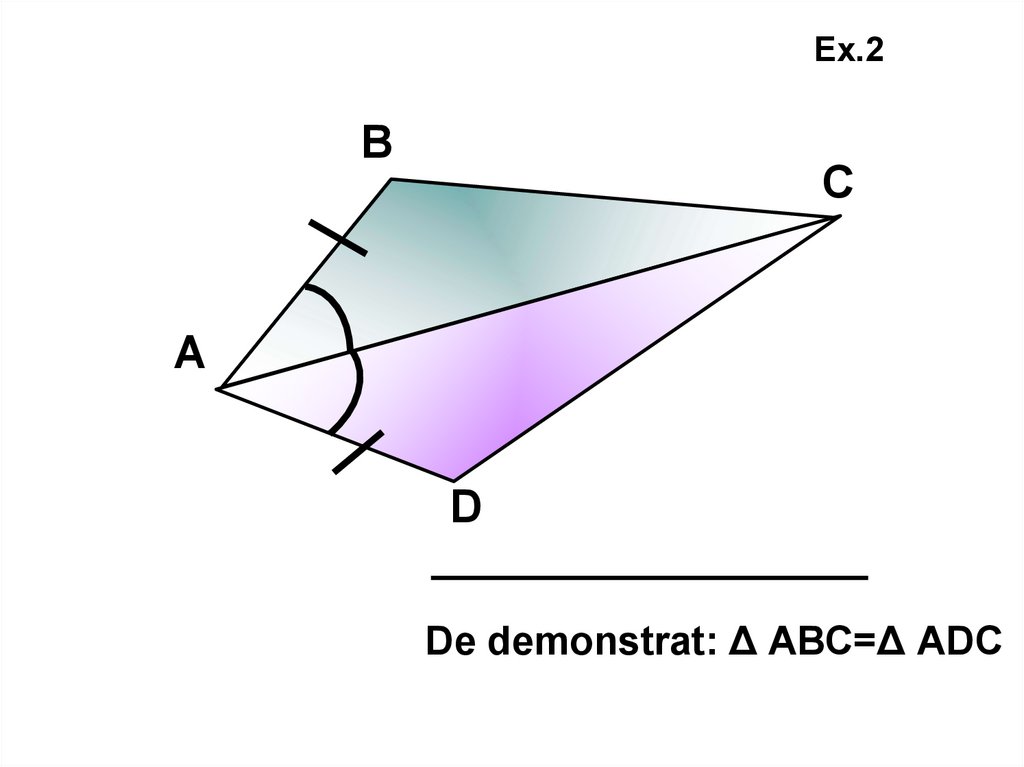
Ex.2В
С
А
D
De demonstrat: Δ АВС=Δ АDС
12.
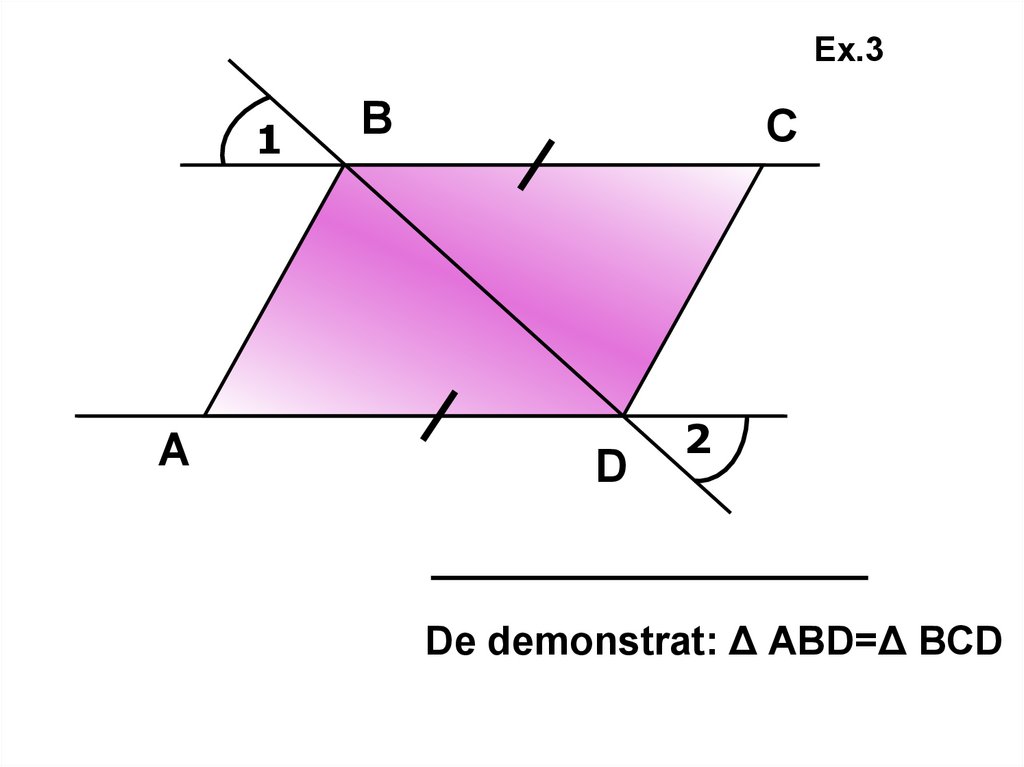
Ex.31
А
В
С
D
2
De demonstrat: Δ АВD=Δ ВСD
13.
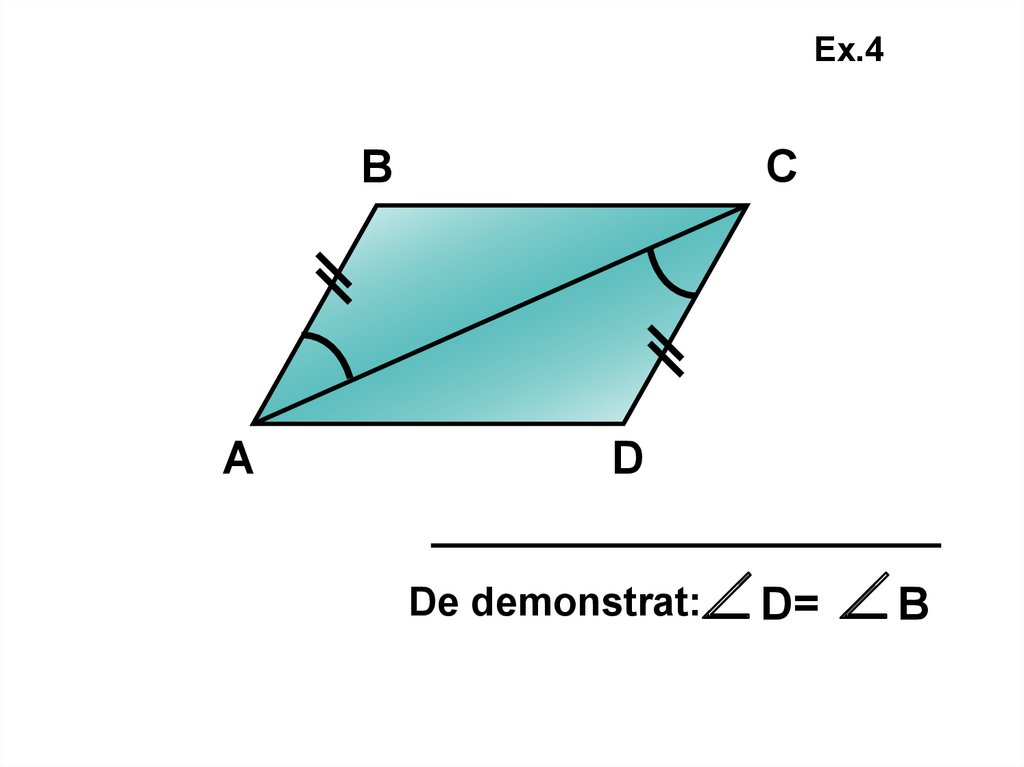
Ex.4В
А
С
D
D= В
De demonstrat:
14.
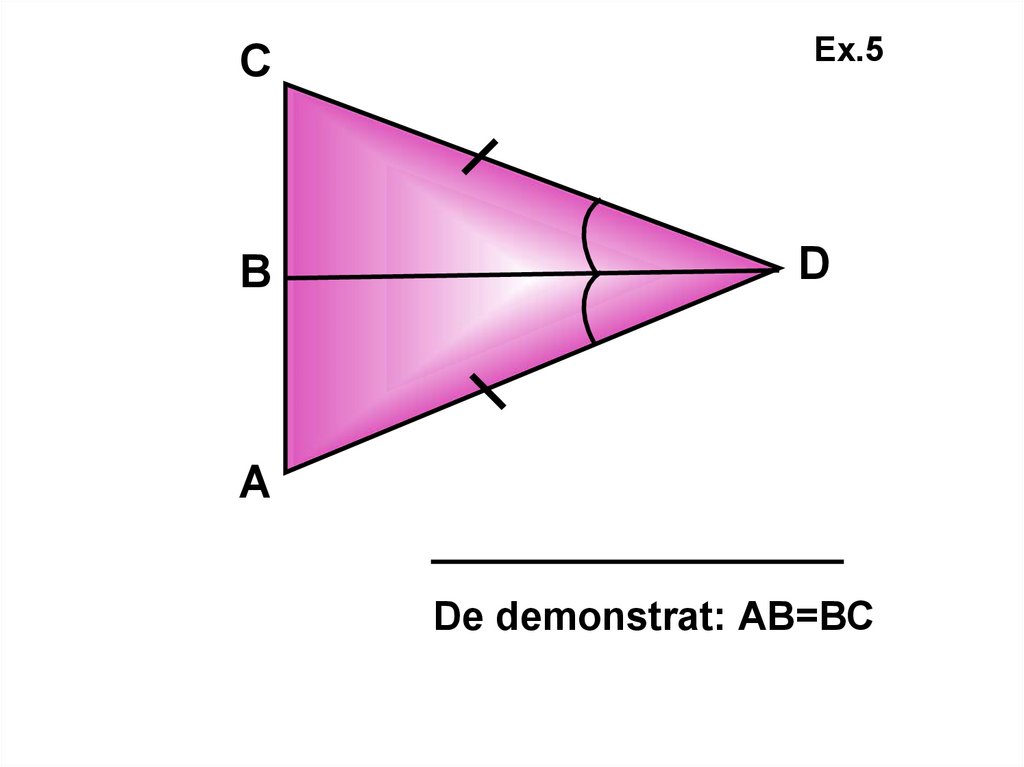
CВ
Ex.5
D
А
De demonstrat: АВ=ВС
15.
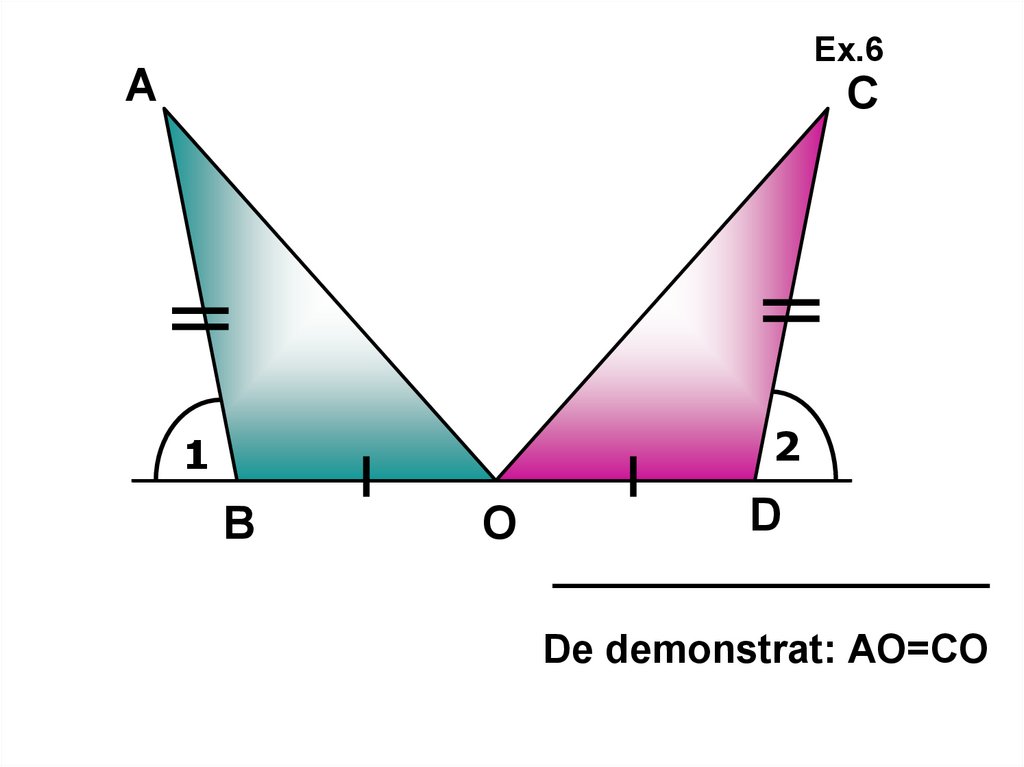
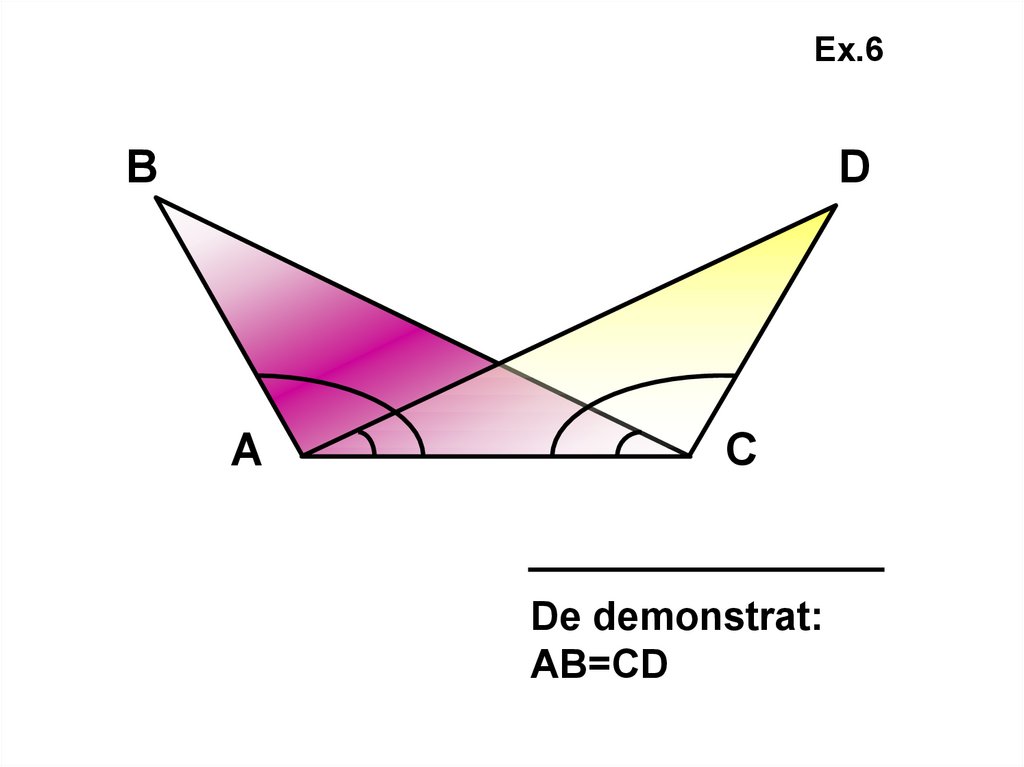
Ex.6А
С
2
1
В
О
D
De demonstrat: АО=СО
16.
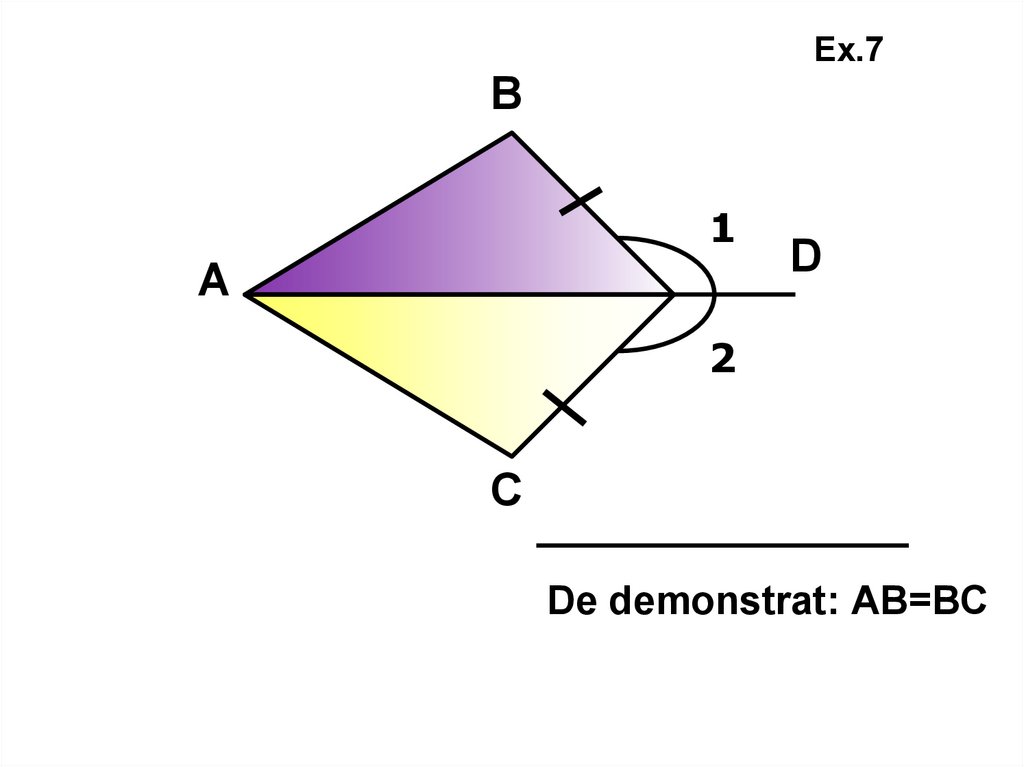
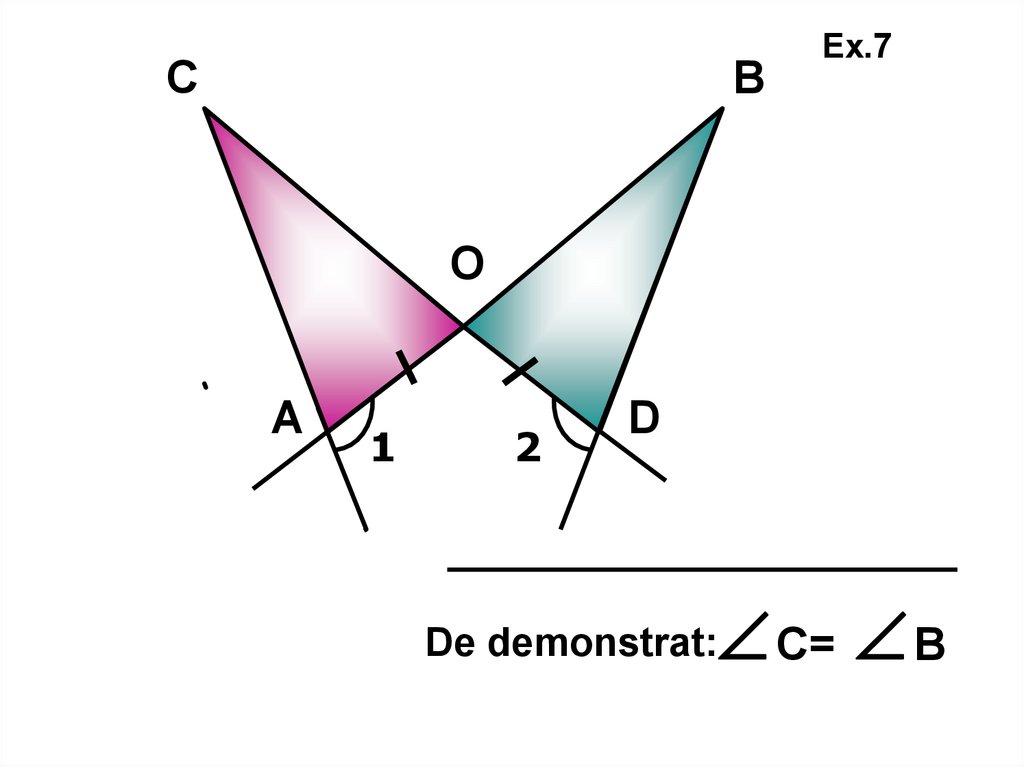
Ex.7В
1
А
D
2
С
De demonstrat: АВ=ВС
17.
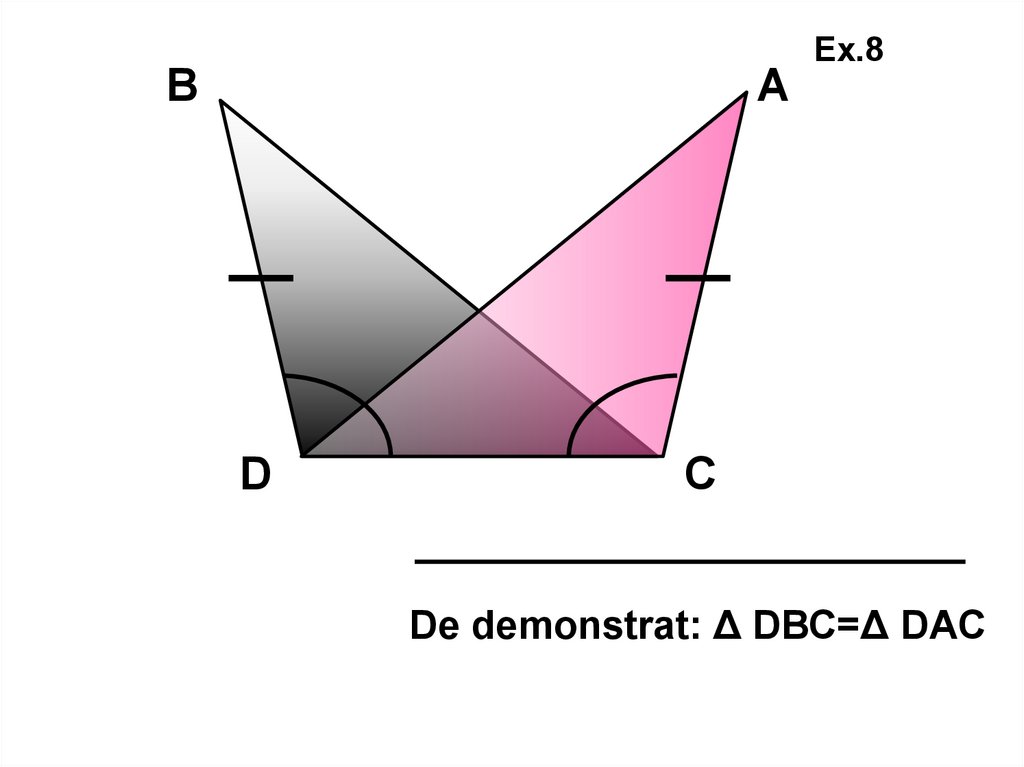
ВА
D
Ex.8
С
De demonstrat: Δ DВС=Δ DАС
18.
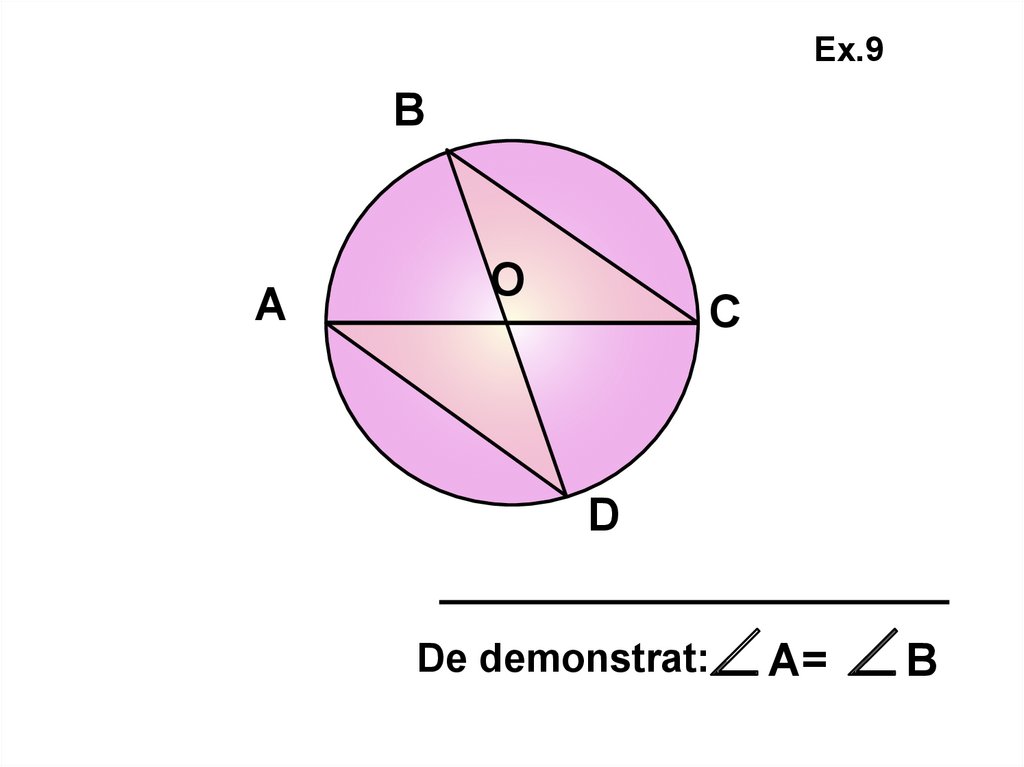
Ex.9В
А
О
C
D
А= В
De demonstrat:
19.
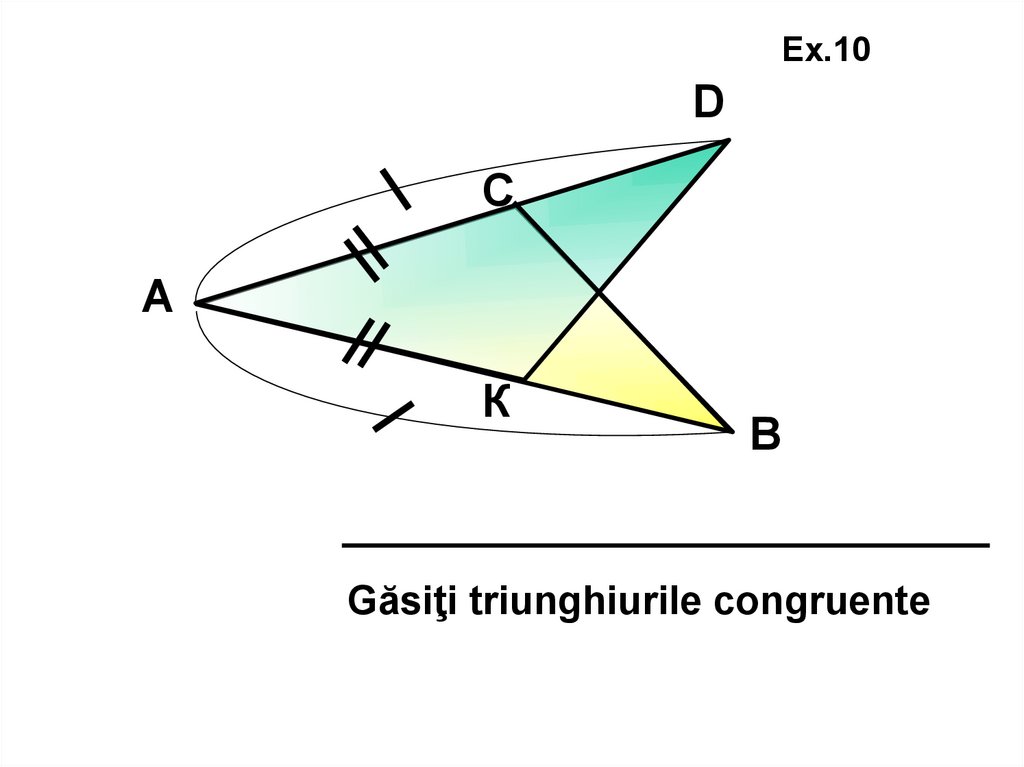
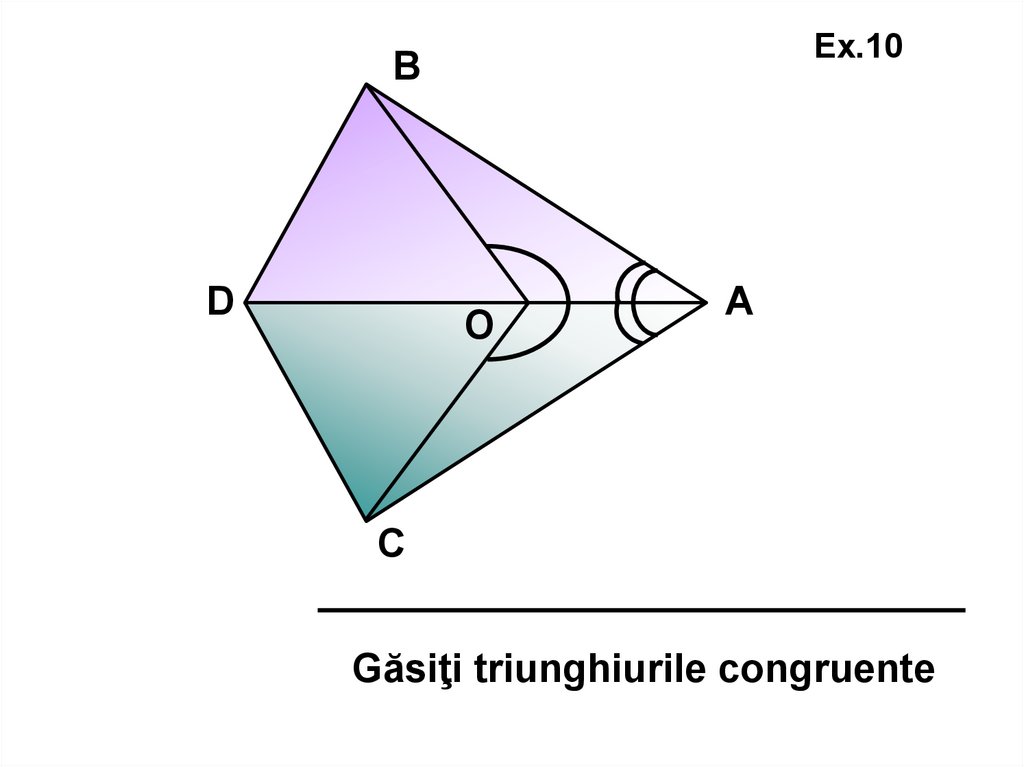
Ex.10D
С
А
К
В
Găsiţi triunghiurile congruente
20.
21.
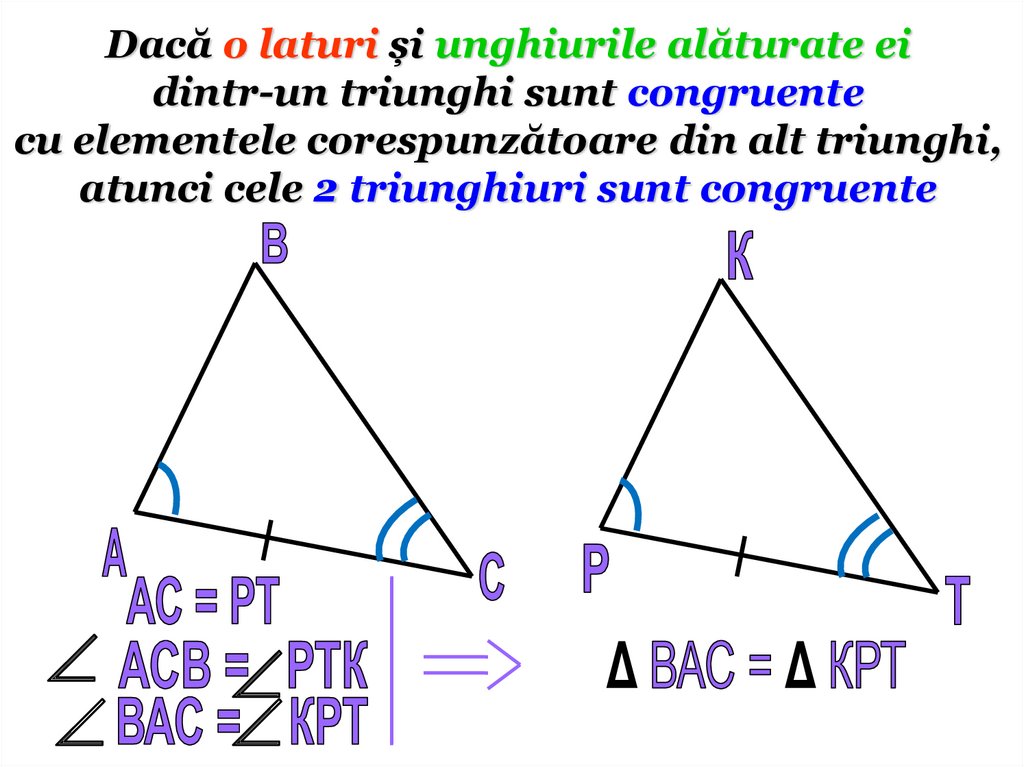
Dacă o laturi și unghiurile alăturate eidintr-un triunghi sunt congruente
cu elementele corespunzătoare din alt triunghi,
atunci cele 2 triunghiuri sunt congruente
22.
23.
24.
25.
26.
27.
Ex.1В
С
О
А
D
D= В
De demonstrat:
28.
ВО
А
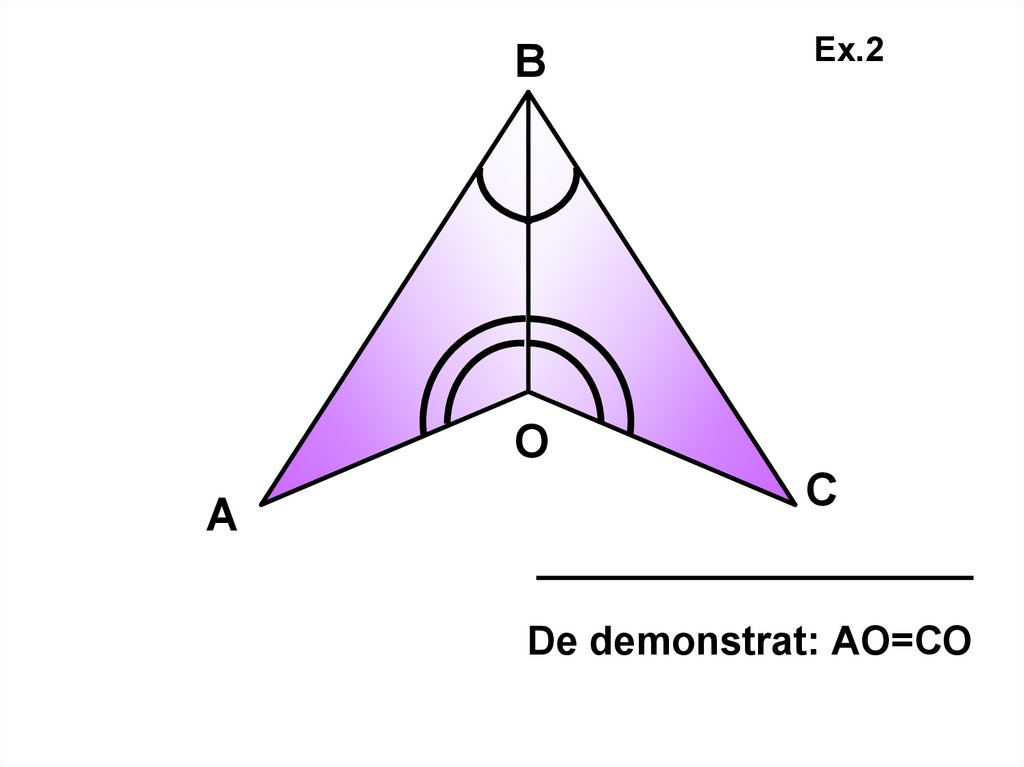
Ex.2
С
De demonstrat: АО=СО
29.
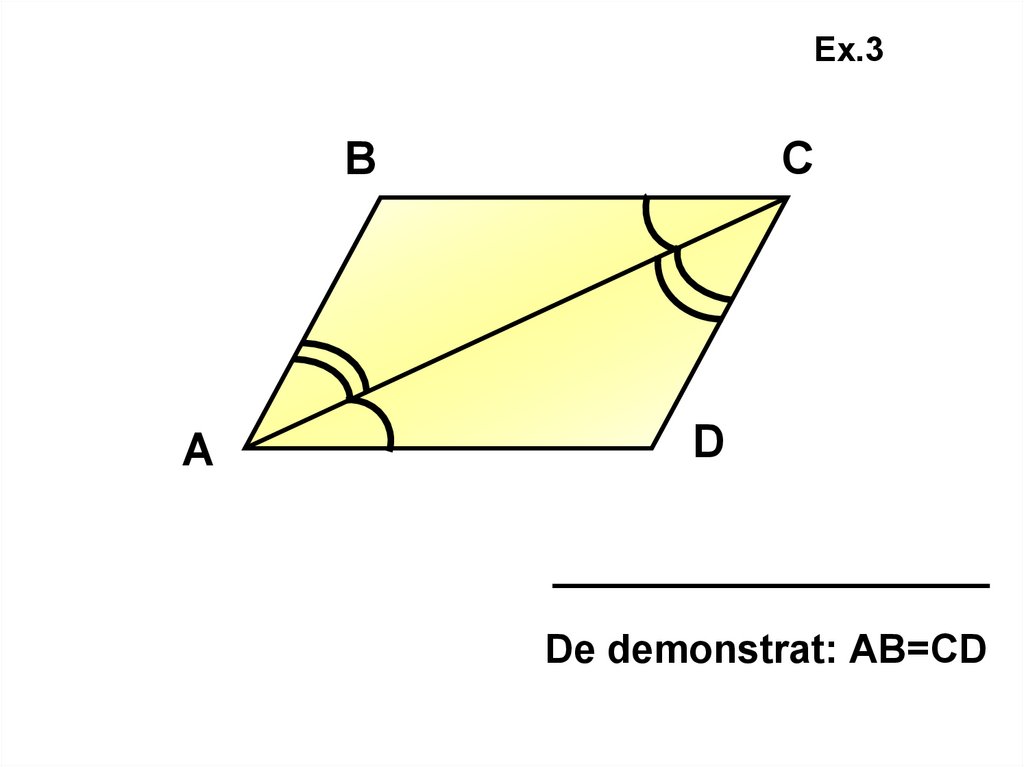
Ex.3В
А
С
D
De demonstrat: АВ=СD
30.
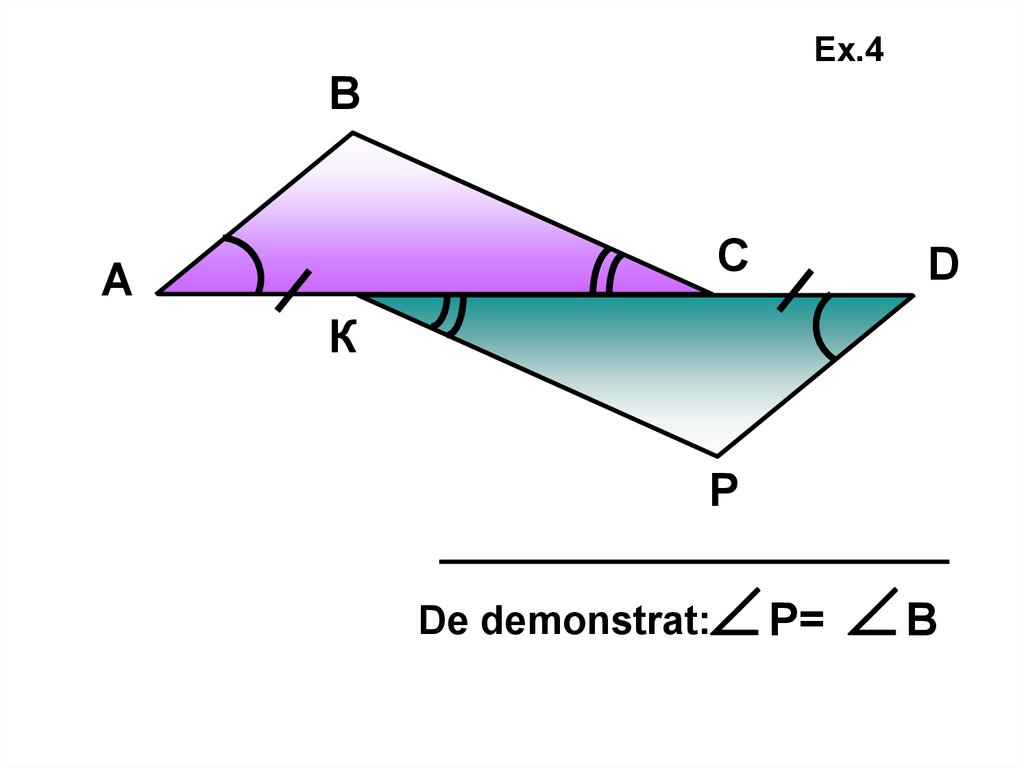
Ex.4В
С
А
D
К
Р
Р= В
De demonstrat:
31.
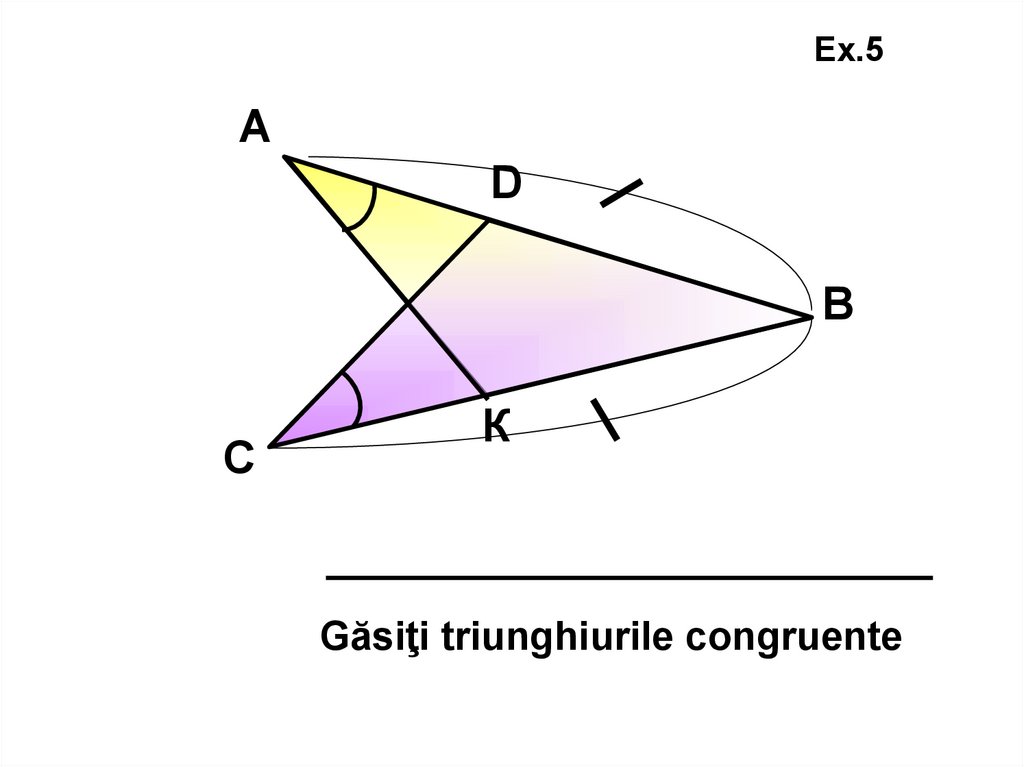
Ex.5А
D
В
С
К
Găsiţi triunghiurile congruente
32.
Ex.6В
D
А
С
De demonstrat:
АВ=СD
33.
СВ
Ex.7
О
А
1
2
D
С= В
De demonstrat:
34.
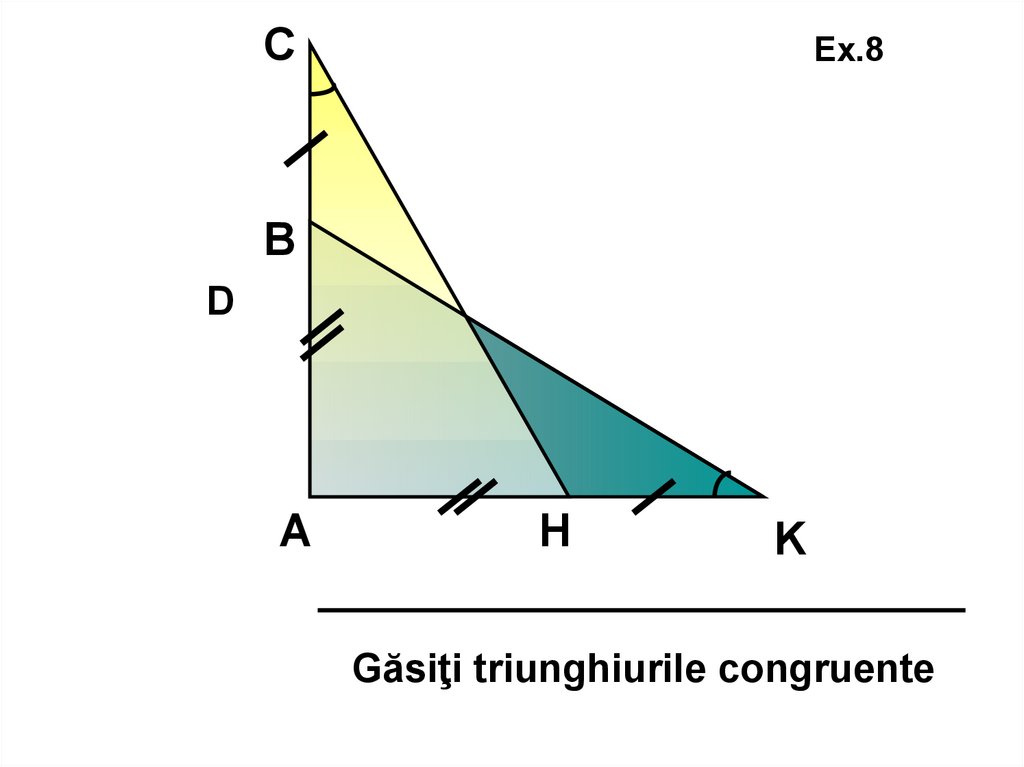
СEx.8
В
D
А
H
K
Găsiţi triunghiurile congruente
35.
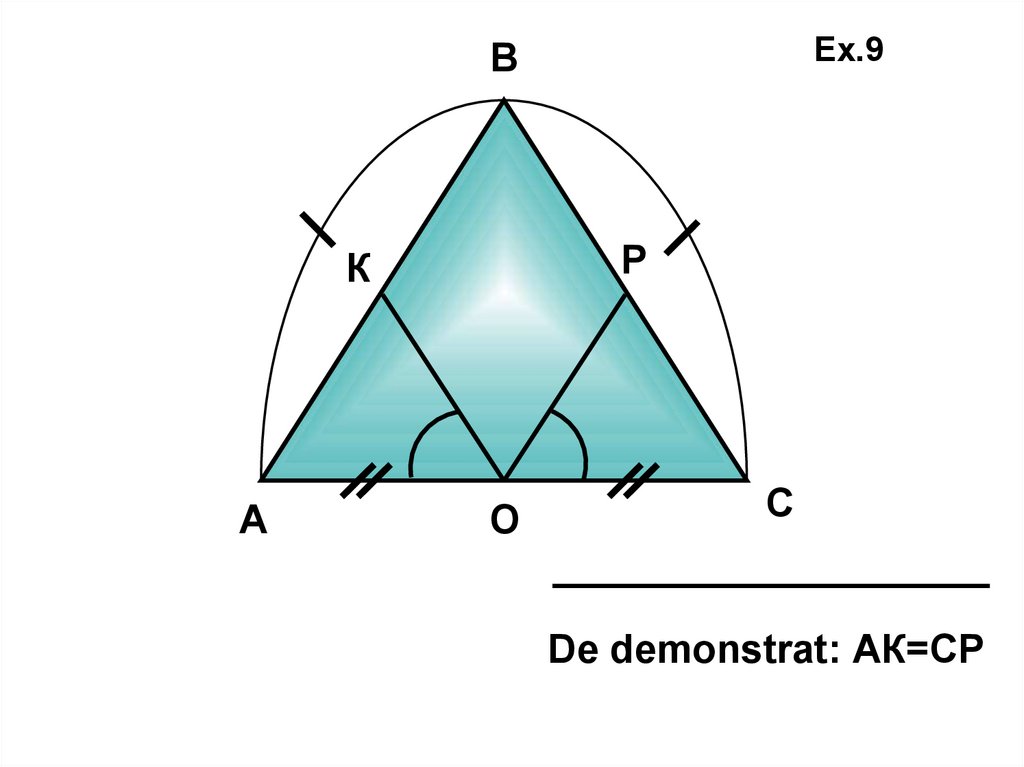
ВР
К
А
Ex.9
О
С
De demonstrat: АК=СР
36.
Ex.10В
D
О
А
С
Găsiţi triunghiurile congruente
37.
ВD
Ex.11
С
О
А
Găsiţi triunghiurile congruente
38.
39.
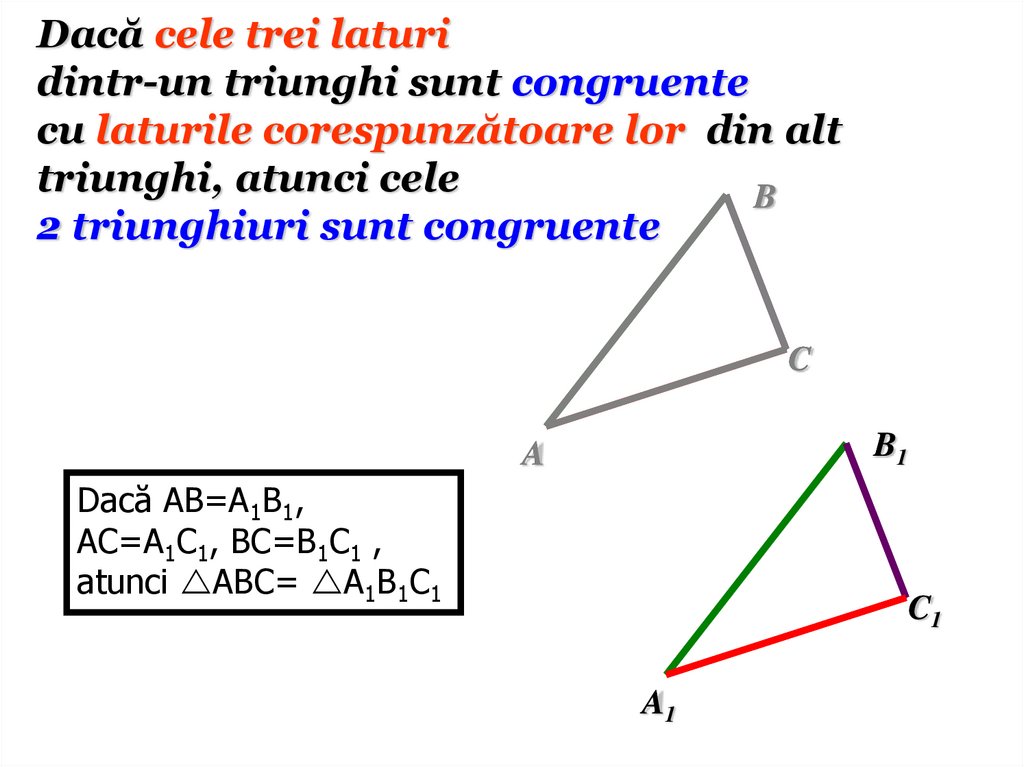
Dacă cele trei laturidintr-un triunghi sunt congruente
cu laturile corespunzătoare lor din alt
triunghi, atunci cele
B
2 triunghiuri sunt congruente
C
B1
A
Dacă AB=A1B1,
AC=A1C1, BC=B1C1 ,
atunci ABC= A1B1C1
C1
A1
40.
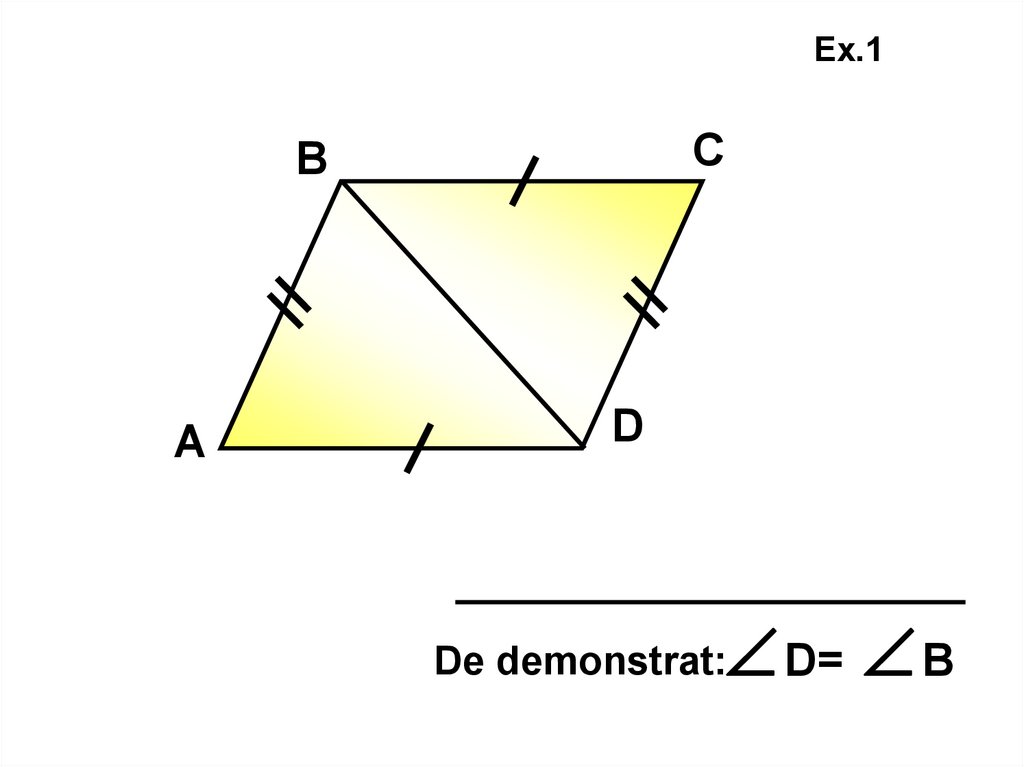
Ex.1С
В
А
D
D= В
De demonstrat:
41.
ВА
D
Ex.2
С
De demonstrat: Δ АВD=Δ ВСD
42.
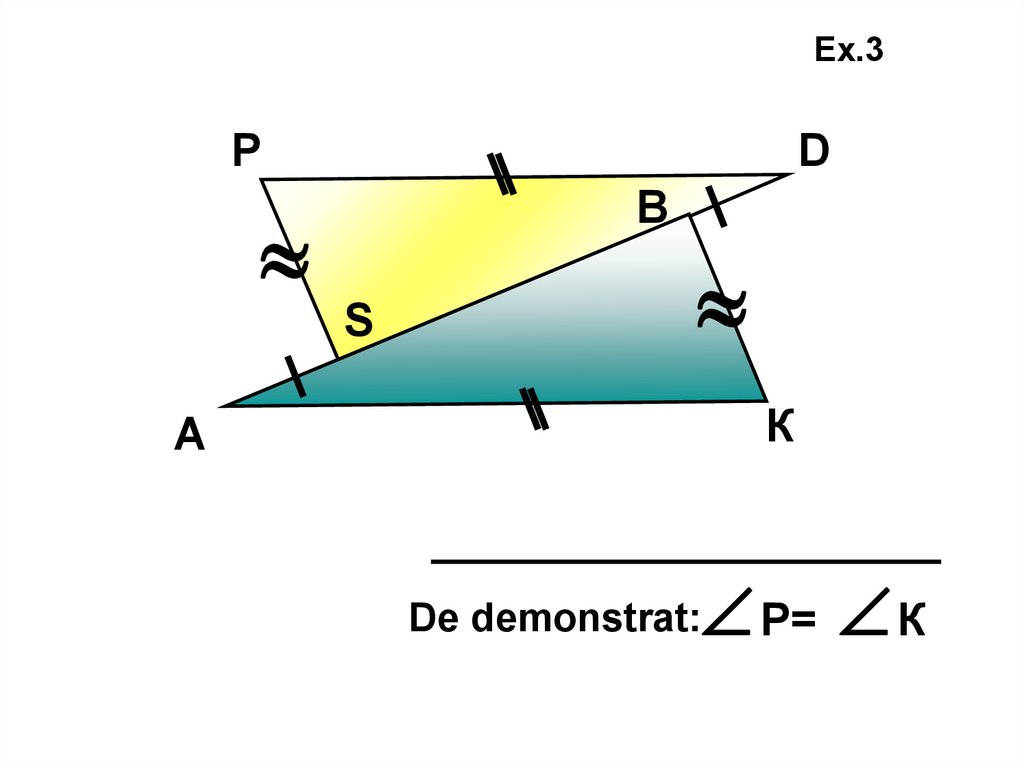
Ex.3Р
D
В
S
К
А
Р= К
De demonstrat:
43.
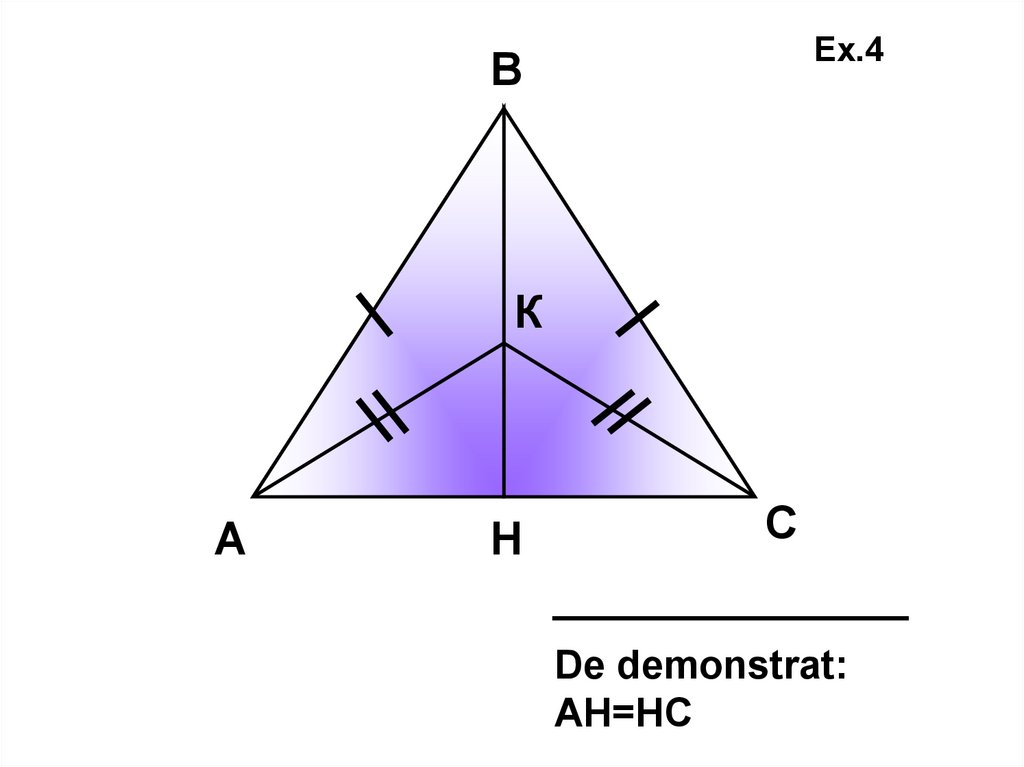
Ex.4В
К
А
Н
С
De demonstrat:
АН=НС
44.
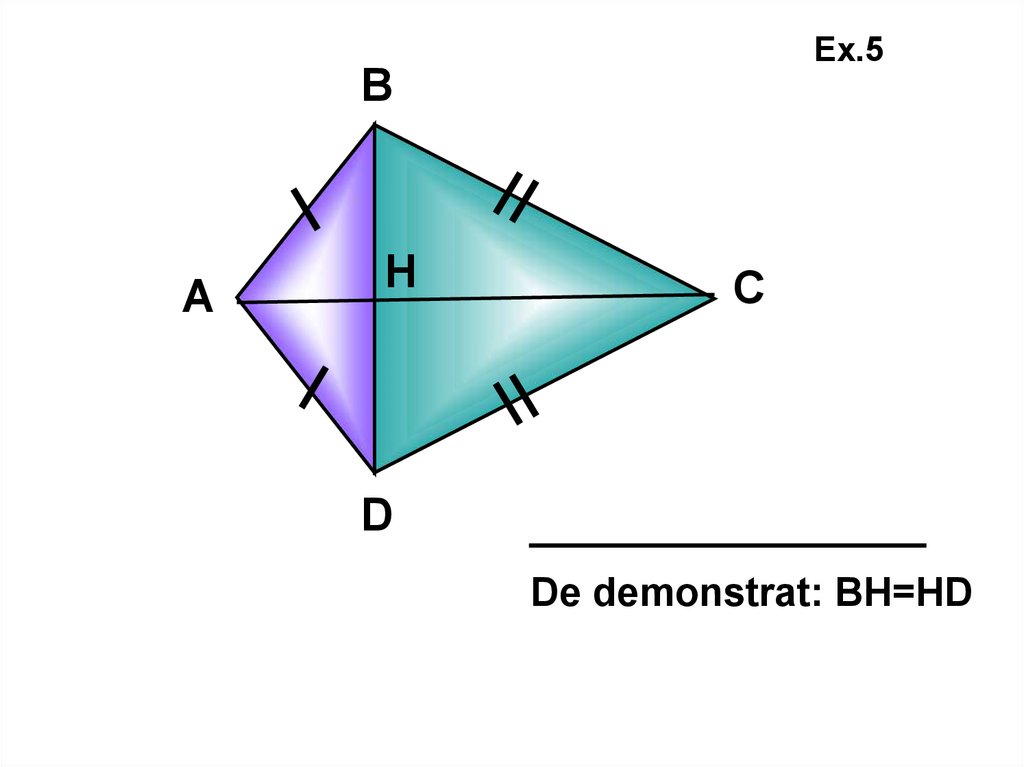
Ex.5В
А
Н
C
D
De demonstrat: ВН=НD
45.
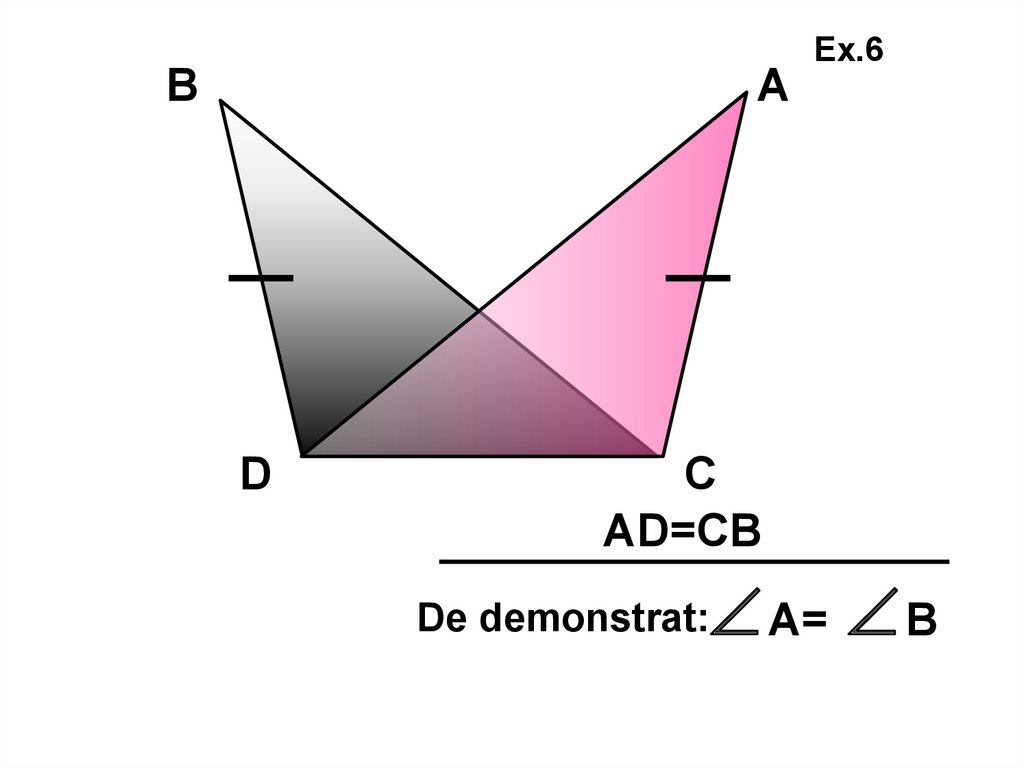
ВА
D
Ex.6
С
АD=СВ
А= В
De demonstrat:
46.
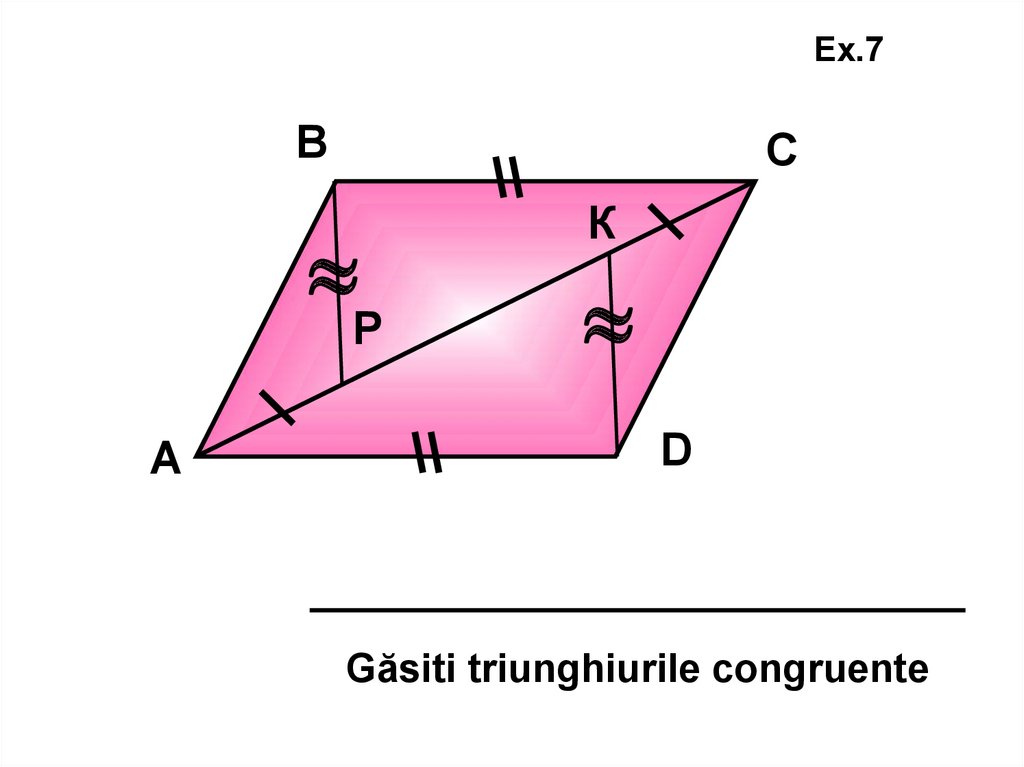
Ex.7В
С
Р
А
К
D
Găsiti triunghiurile congruente
47.
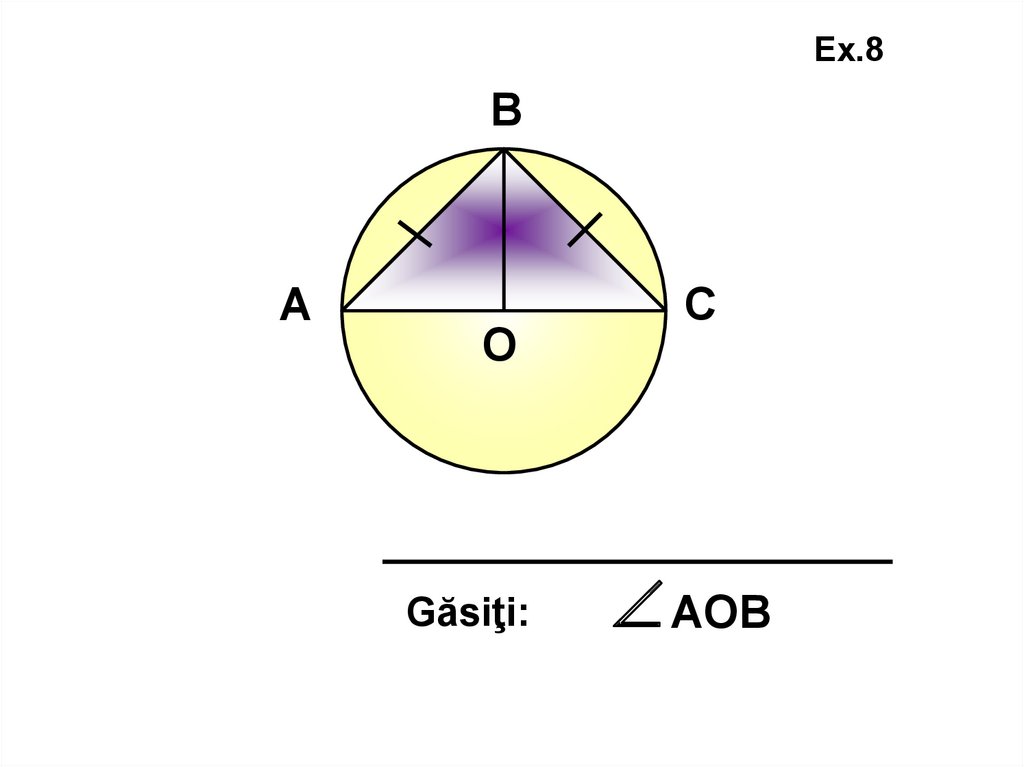
Ex.8В
А
О
Găsiţi:
С
АОВ
48.
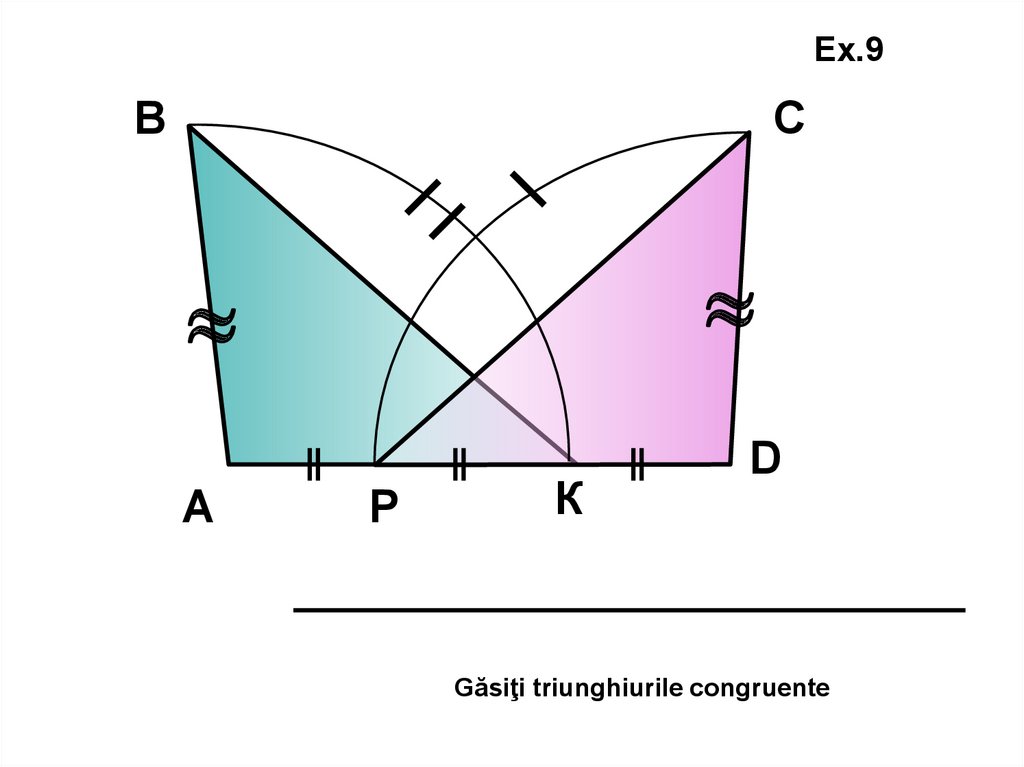
Ex.9В
С
А
Р
К
D
Găsiţi triunghiurile congruente
49.
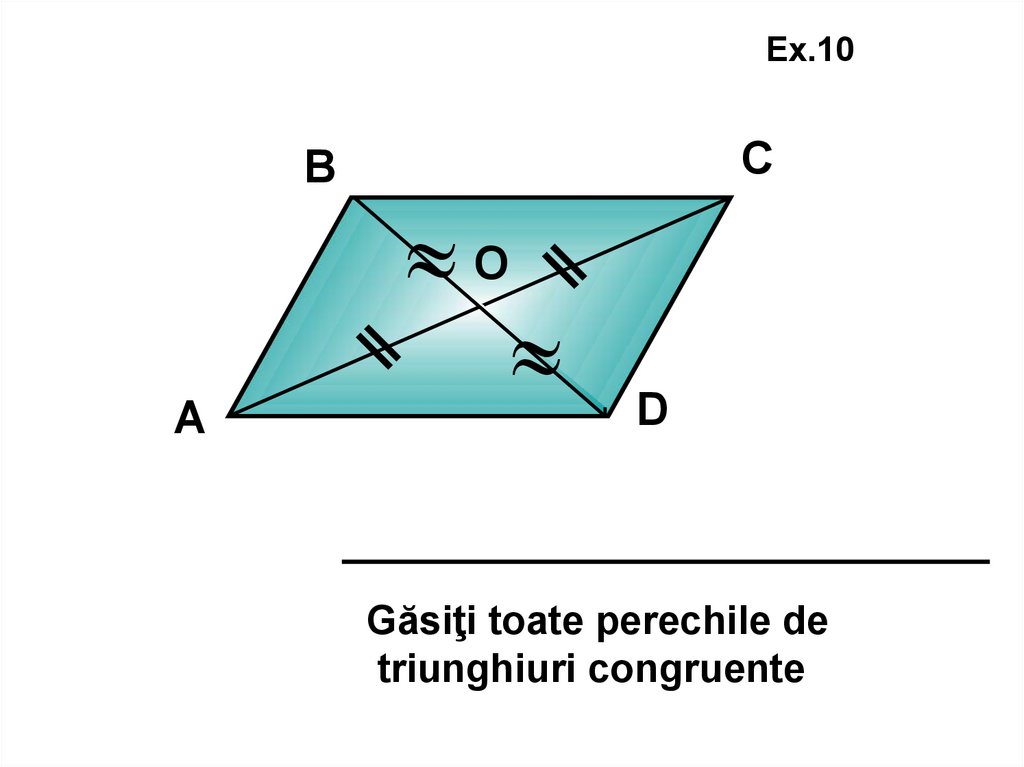
Ex.10В
А
С
О
D
Găsiţi toate perechile de
triunghiuri congruente






 mathematics
mathematics

