Similar presentations:
Tukey Boxplots
1.
CONCEPTS TO BE INCLUDEDWhy Box plots? Distributions of various groups ... Three distributions
Tukey box plot
Median
1st and 3d quarter
IQR
Whiskers
Outliers
2.
TUKEY BOXPLOTS3.
AIMTukey boxplot for ratio and interval variables
Usefullness of the boxplot
Box, whiskers, median, 1st and 3d quarter, IQR, outliers
4.
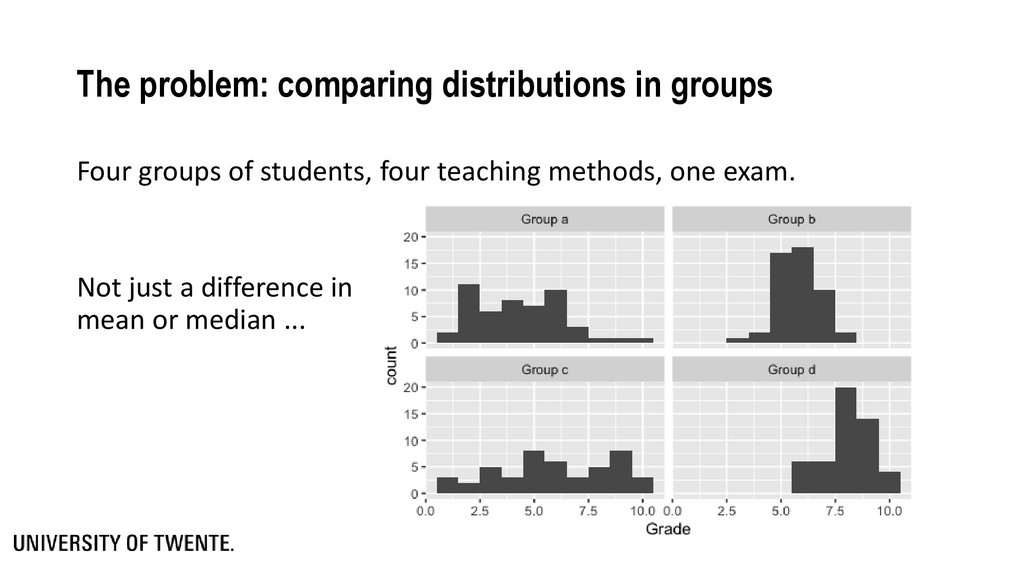
The problem: comparing distributions in groupsFour groups of students, four teaching methods, one exam.
Not just a difference in
mean or median ...
5.
John Wilder Tukey (1915-2000)Invented the word ‘bit’ in 1947 (from
binary and digit).
Came up with the word ‘software’ (1953/1958)
to mark the difference between computer
programs and computers (‘hardware’).
Introduced the boxplot in 1969.
Confusingly, nowadays various versions of
‘boxplots’.
6.
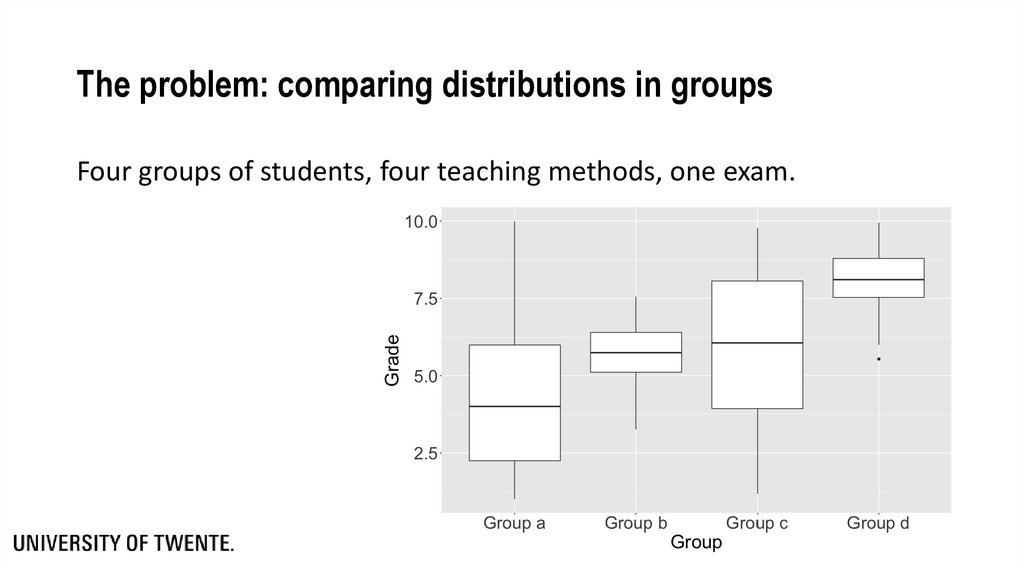
The problem: comparing distributions in groupsFour groups of students, four teaching methods, one exam.
7.
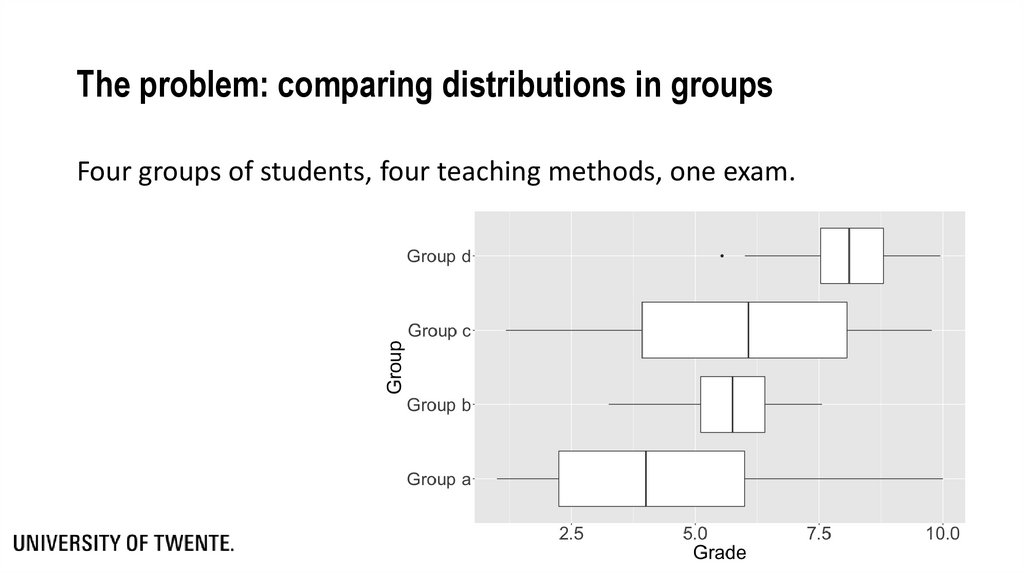
The problem: comparing distributions in groupsFour groups of students, four teaching methods, one exam.
8.
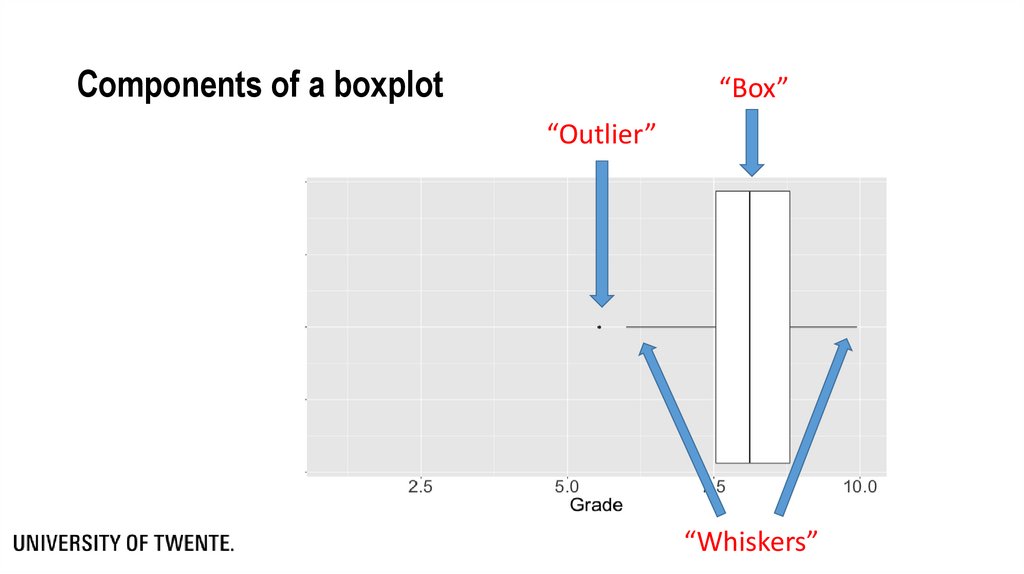
Components of a boxplot“Box”
“Outlier”
“Whiskers”
9.
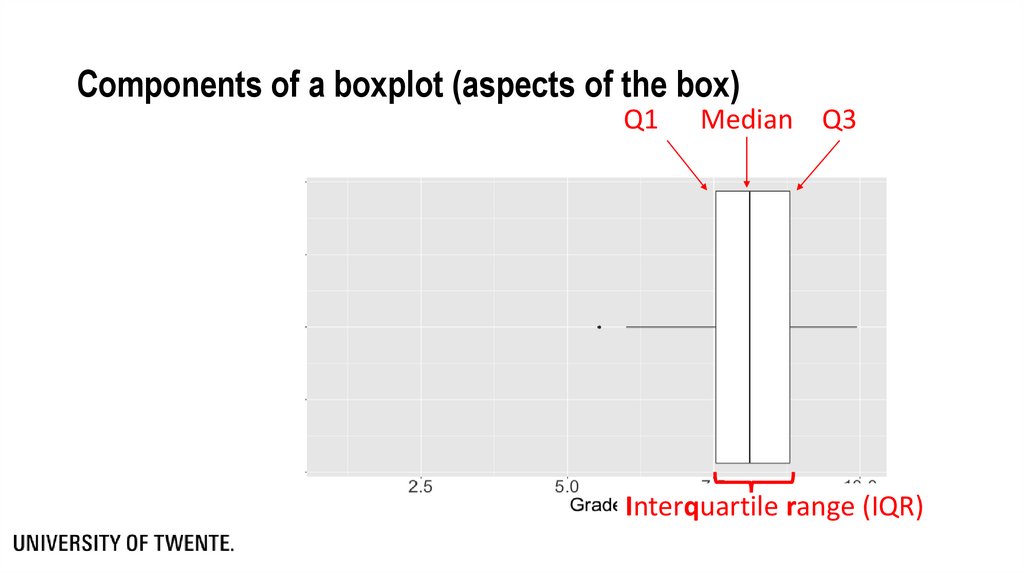
Components of a boxplot (aspects of the box)Q1
Median Q3
Interquartile range (IQR)
10.
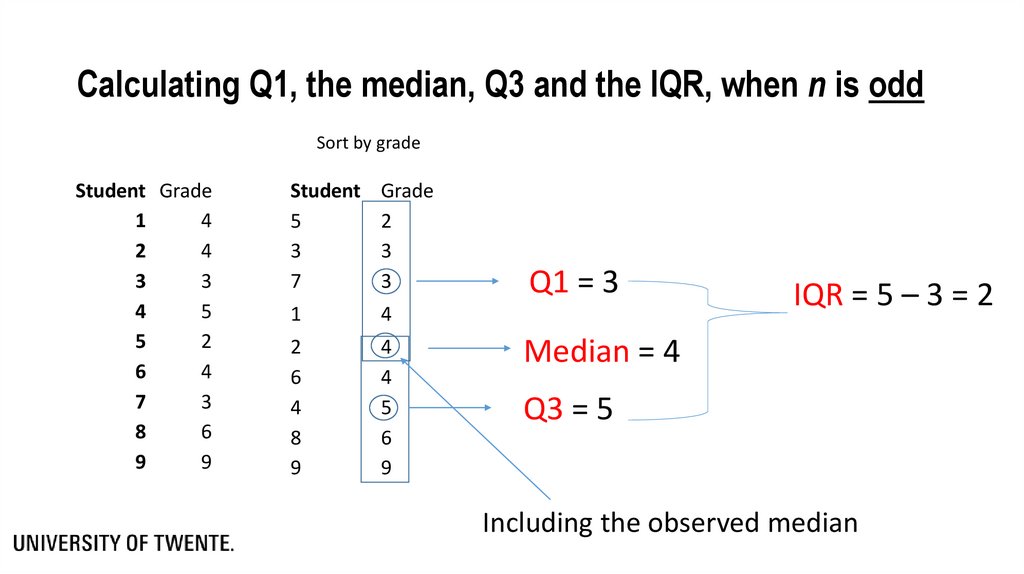
Calculating Q1, the median, Q3 and the IQR, when n is oddSort by grade
Student Grade
1
4
2
4
3
3
4
5
5
2
6
4
7
3
8
6
9
9
Student
5
3
7
Grade
2
3
3
1
4
2
6
4
8
9
4
4
5
6
9
Q1 = 3
IQR = 5 – 3 = 2
Median = 4
Q3 = 5
Including the observed median
11.
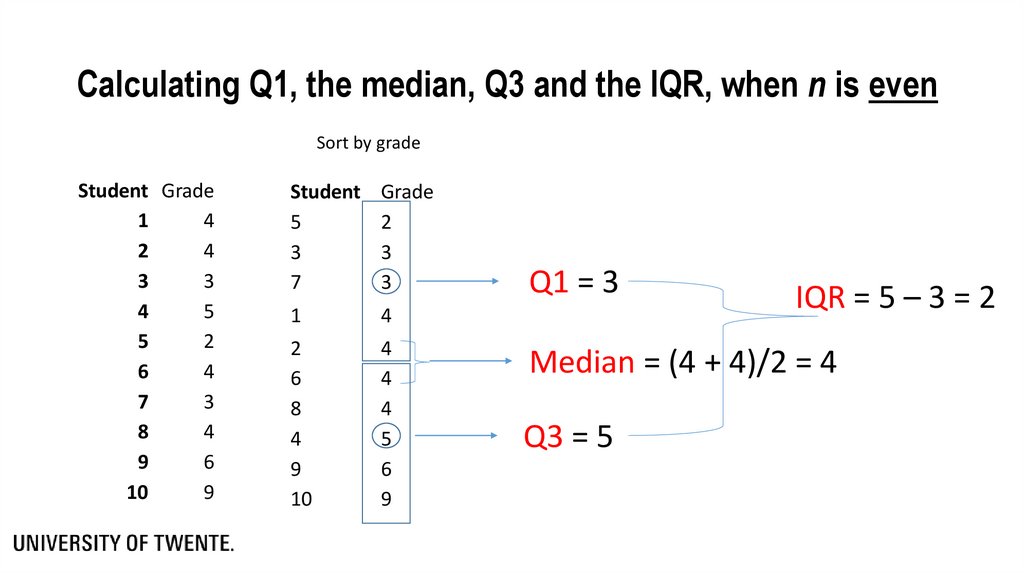
Calculating Q1, the median, Q3 and the IQR, when n is evenSort by grade
Student Grade
1
4
2
4
3
3
4
5
5
2
6
4
7
3
8
4
9
6
10
9
Student
5
3
7
Grade
2
3
3
1
4
2
6
8
4
9
10
4
4
4
5
6
9
Q1 = 3
IQR = 5 – 3 = 2
Median = (4 + 4)/2 = 4
Q3 = 5
12.
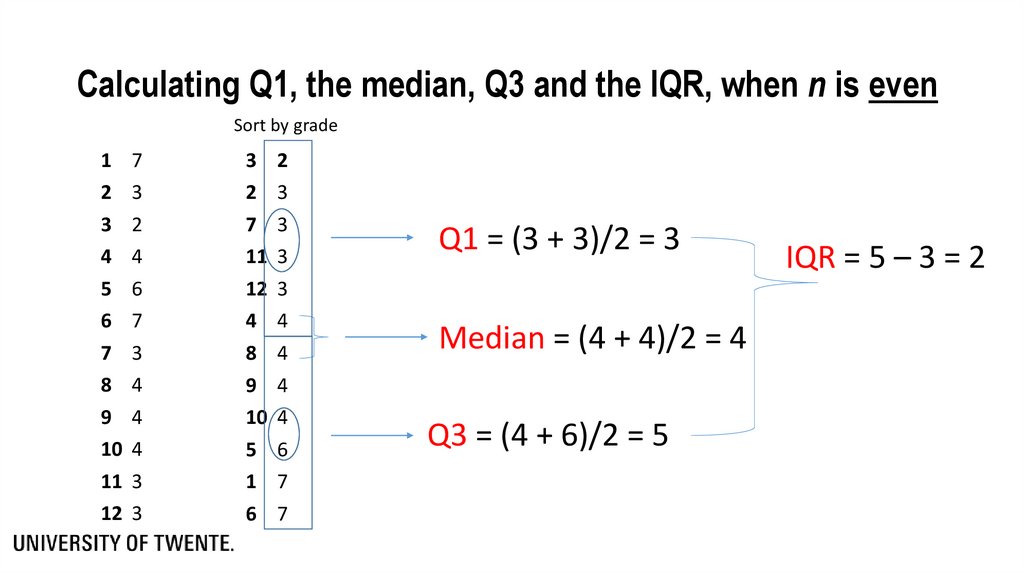
Calculating Q1, the median, Q3 and the IQR, when n is evenSort by grade
1
2
3
4
5
6
7
8
9
10
11
12
7
3
2
4
6
7
3
4
4
4
3
3
3
2
7
11
12
4
8
9
10
5
1
6
2
3
3
3
3
4
4
4
4
6
7
7
Q1 = (3 + 3)/2 = 3
Median = (4 + 4)/2 = 4
Q3 = (4 + 6)/2 = 5
IQR = 5 – 3 = 2
13.
IQR and whiskersWhiskers are max 1.5 IQR long
If there are no observations at 1.5 IQR,
the whiskers end at the largest observation within that range.
14.
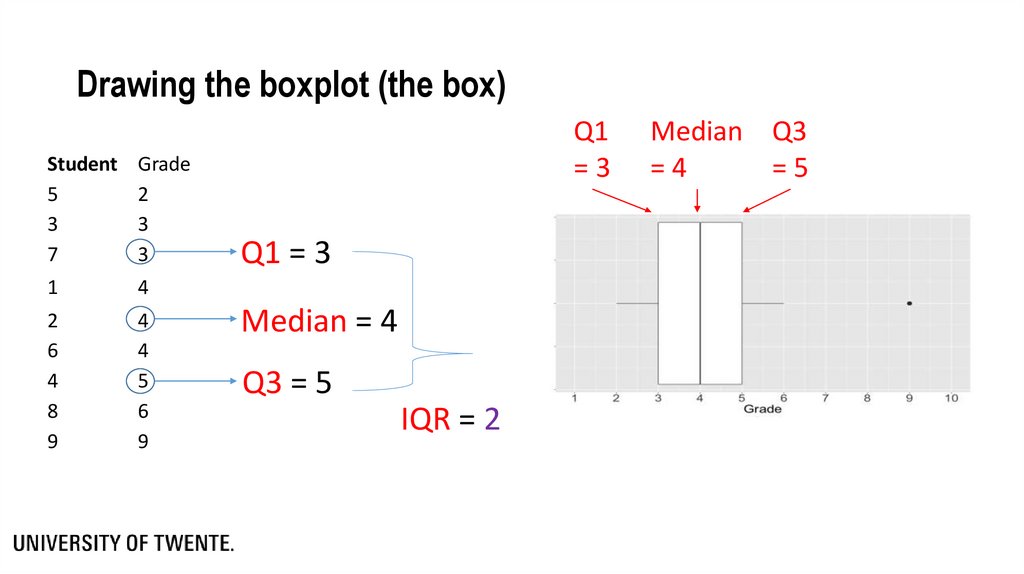
Drawing the boxplot (the box)Student
5
3
7
Grade
2
3
3
1
4
2
6
4
8
9
4
4
5
6
9
Q1
=3
Q1 = 3
Median = 4
Q3 = 5
IQR = 2
Median Q3
=4
=5
15.
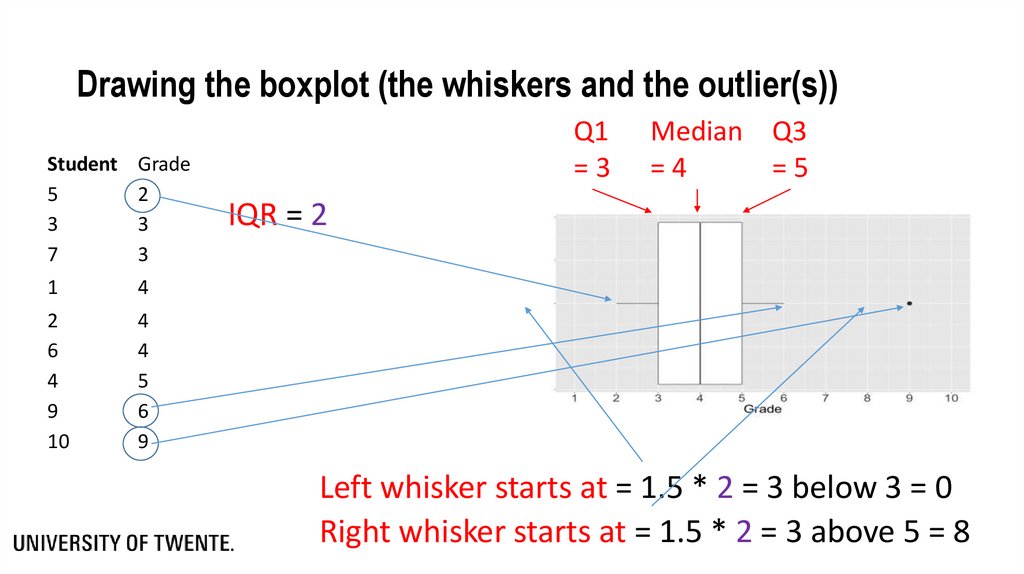
Drawing the boxplot (the whiskers and the outlier(s))Student
5
3
7
Grade
2
3
3
1
4
2
6
4
9
10
4
4
5
6
9
Q1
=3
Median Q3
=4
=5
IQR = 2
Left whisker starts at = 1.5 * 2 = 3 below 3 = 0
Right whisker starts at = 1.5 * 2 = 3 above 5 = 8
16.
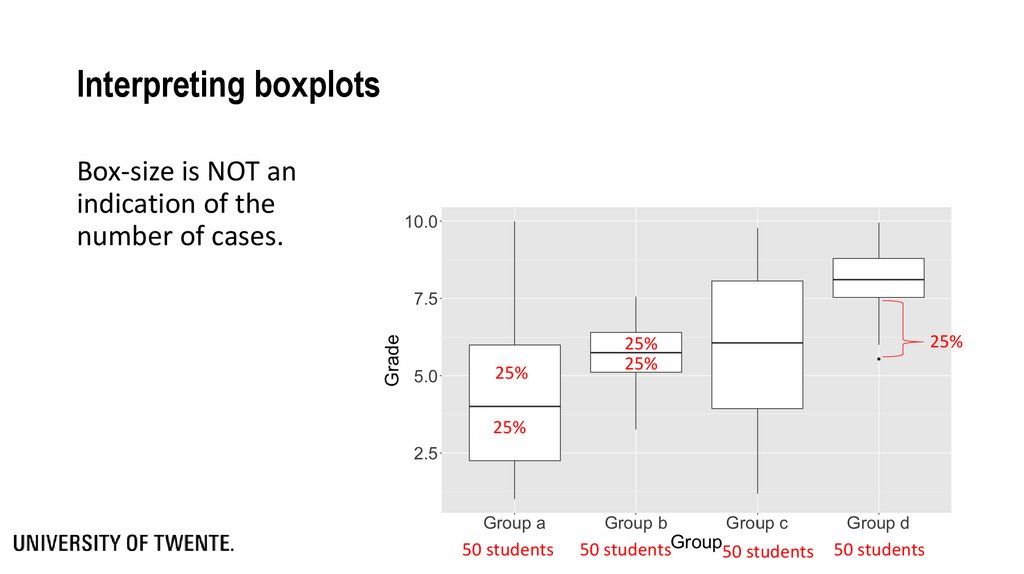
Interpreting boxplotsBox-size is NOT an
indication of the
number of cases.
25%
25%
25%
25%
25%
50 students
50 students
50 students
50 students
17.
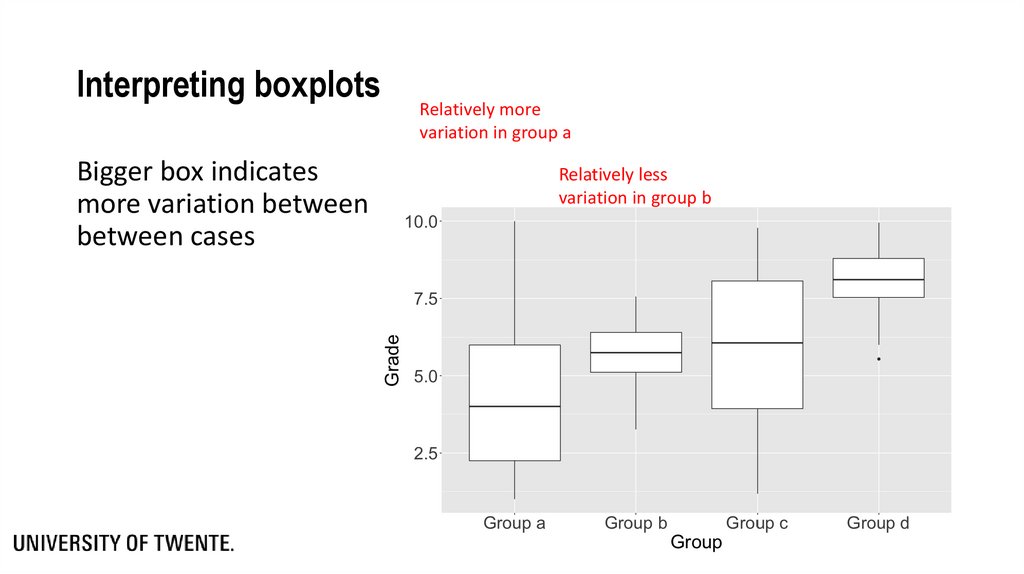
Interpreting boxplotsBigger box indicates
more variation between
between cases
Relatively more
variation in group a
Relatively less
variation in group b
18.
This micro lectureTukey boxplot for ratio and interval variables
Box, whiskers, median, 1st and 3d quarter, IQR, outliers
Usefullness of the boxplot







 mathematics
mathematics








