Similar presentations:
Trigonometriýa
1. Trigonometriýa
2.
Sinusyň we kosinusyňkesgitlenişi
y
M cos ; sin
1
M o 1;0
0
1
x
cos xM
sin yM
3.
Tangensiň kesgitlenişiy
tg
1
0
1
x
sin
tg
cos
4.
Kotangensiň kesgitlenişiy
ctg
1
0
1
x
cos
ctg
sin
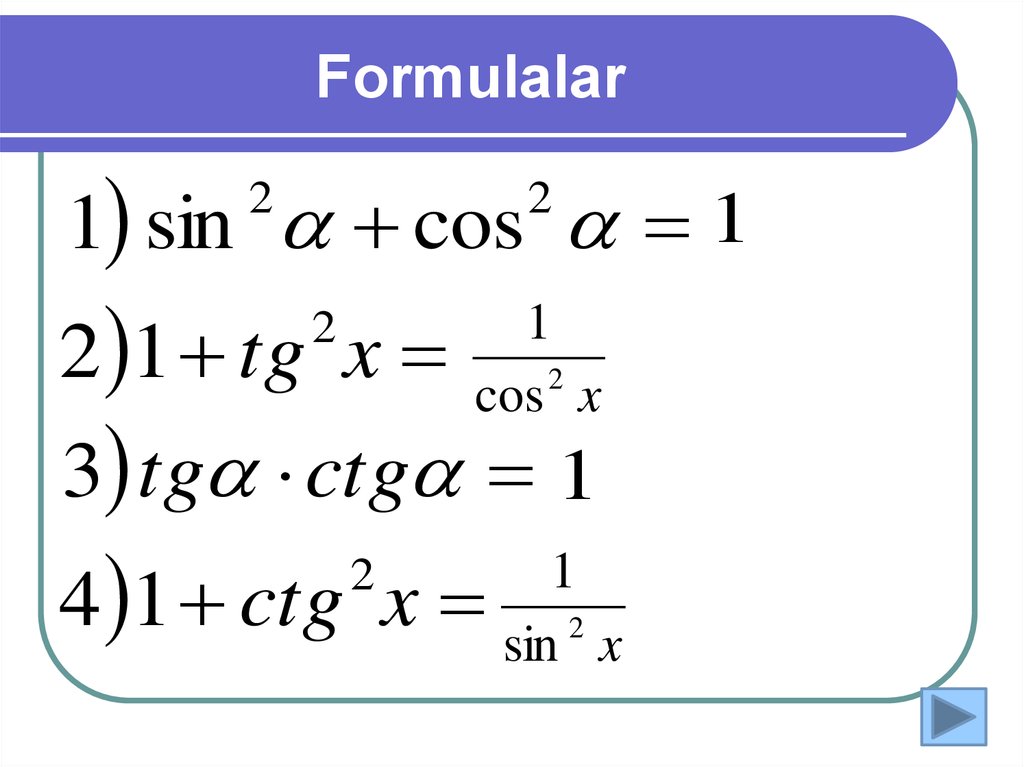
5. Formulalar
1 sin cos 12
2
2 1 tg x
2
1
2
cos x
3 tg ctg 1
4 1 ctg x
2
1
2
sin x
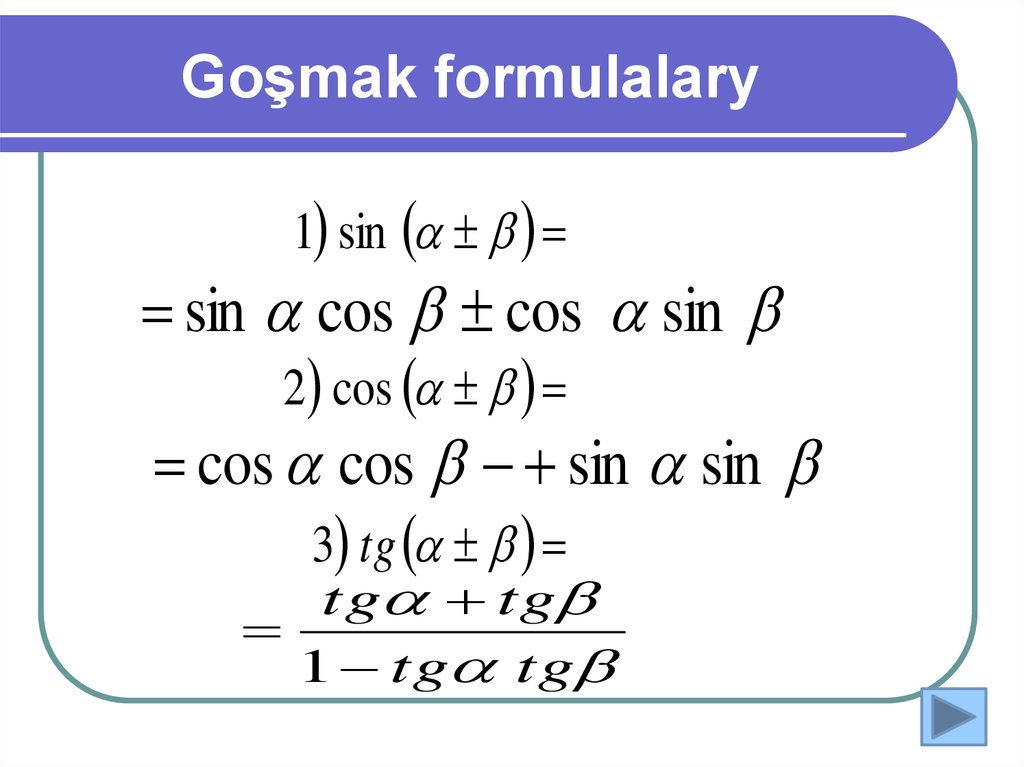
6.
Goşmak formulalary1 sin
sin cos cos sin
2 cos
cos cos sin sin
3 tg
tg tg
1 tg tg
7. Ikeldilen burçuň trigonometrik funksiýalary
1 sin 2 2 sin cos2
2
2 cos 2 cos sin
3 tg 2
2tg
2
1 tg
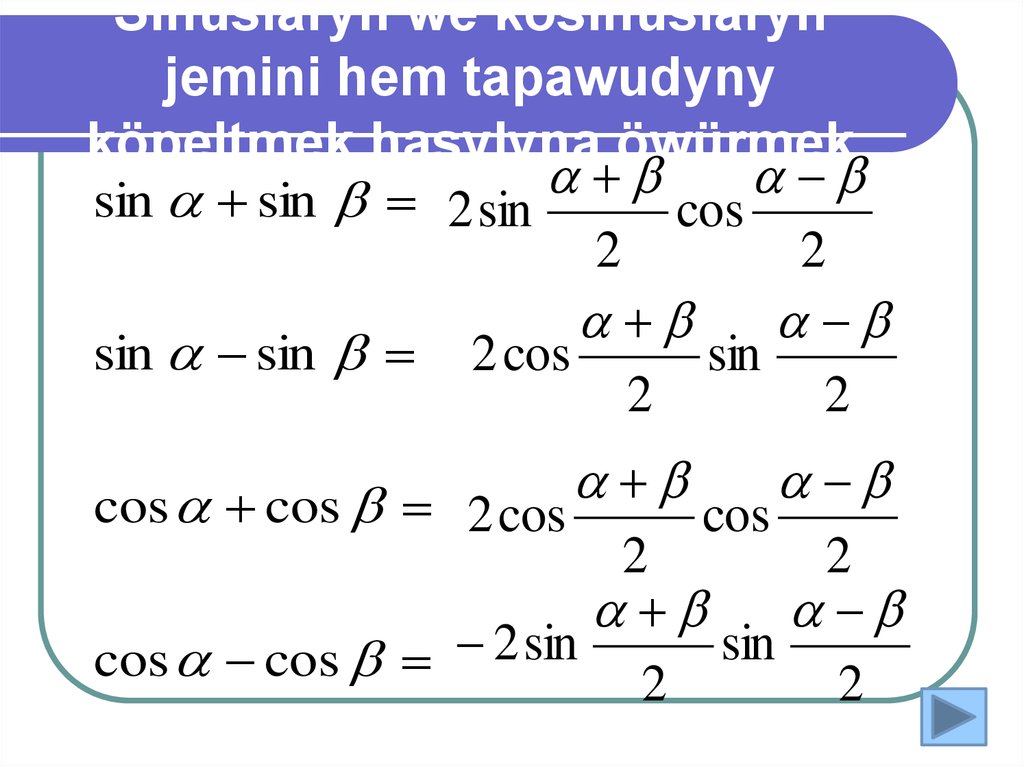
8. Sinuslaryň we kosinuslaryň jemini hem tapawudyny köpeltmek hasylyna öwürmek
sin sin 2 sincos
2
2
sin sin 2 cos
sin
2
2
cos cos 2 cos
cos
2
2
cos cos 2 sin 2 sin 2
9. Ýarym burçuň trigonometrik funksiýalary
22
1 cos
sin
2
2
cos
tg
2
2
2
1 cos
2
1 cos
1 cos
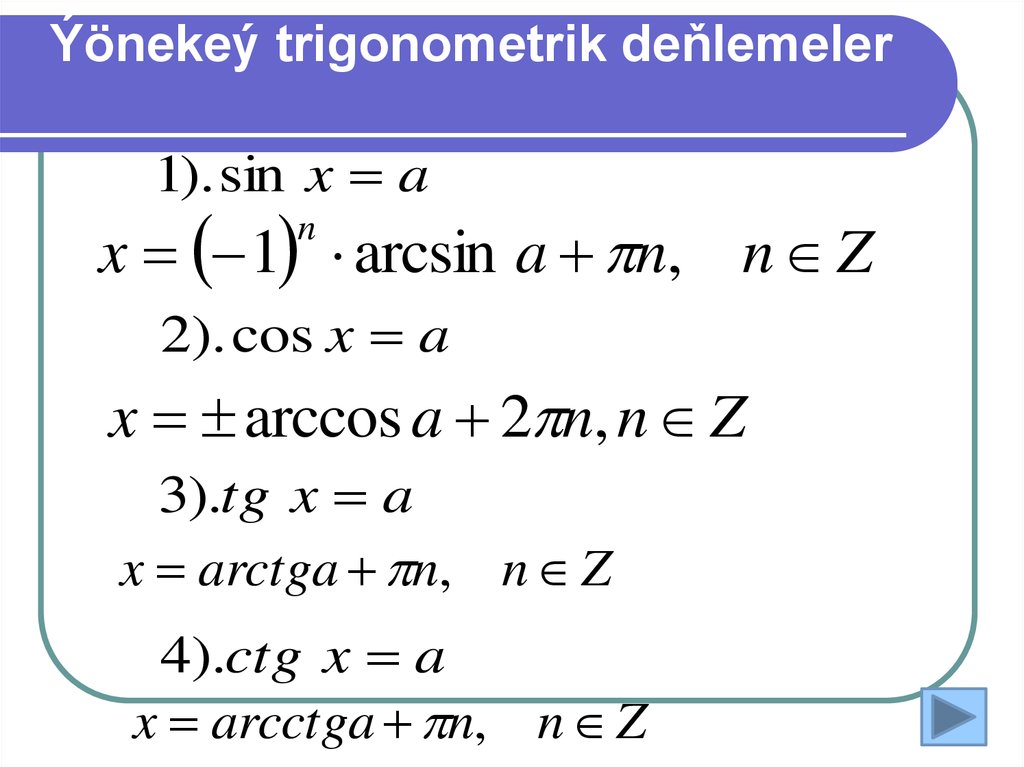
10.
Ýönekeý trigonometrik deňlemeler1). sin x a
x 1 arcsin a n, n Z
n
2). cos x a
x arccos a 2 n, n Z
3).tg x a
x arctga n, n Z
4).ctg x a
x arcctga n, n Z
11.
Trigonometrik deňlemeleriň hususy hallary1). sin x 1
у
1
х
0
1
x 2 n, n Z
2
2). sin x 0
x n, n Z
3). sin x 1
x
2
2 n, n Z
12.
Trigonometrik deňlemeleriň hususy hallary1). cos x 1
у
1
х
0
1
x 2 n, n Z
2). cos x 0
x
2
n, n Z
3). cos x 1
x 2 n, n Z
13.
Trigonometrik deňlemeleriň hususy hallaryу
1
1
х
0
0
-1
1).tgx 1
x n, n Z
4
2).tgx 0
x n, n Z
3).tgx 1
x
4
n, n Z
14.
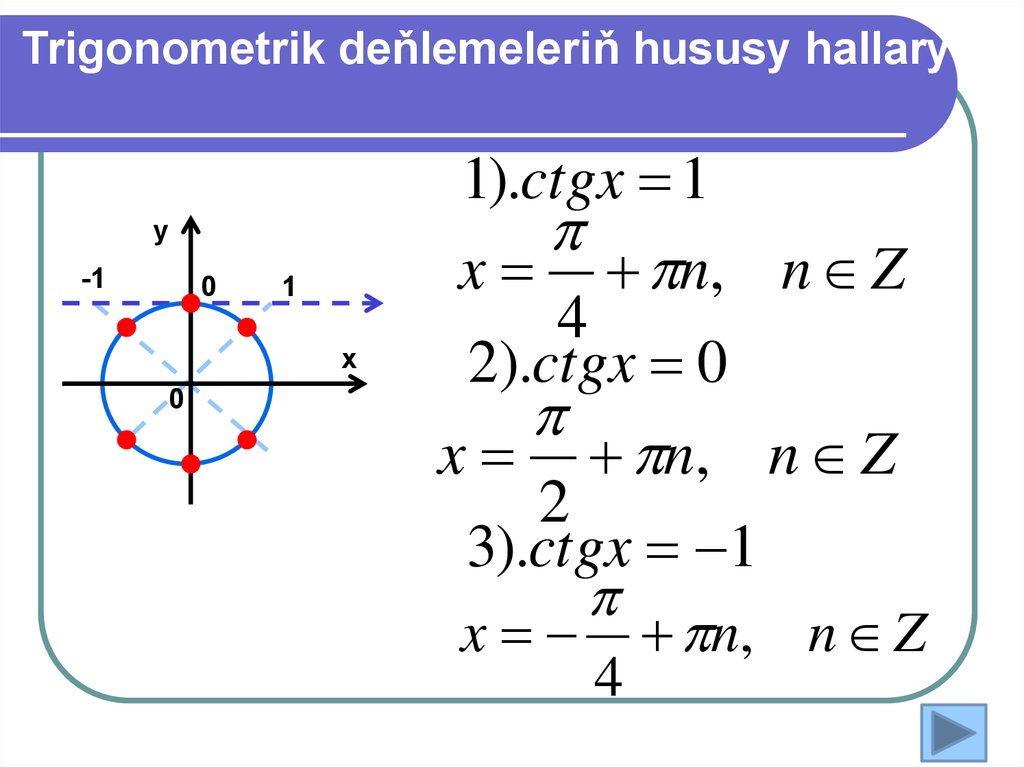
Trigonometrik deňlemeleriň hususy hallaryу
-1
0
1
х
0
1).ctgx 1
x n, n Z
4
2).ctgx 0
x n, n Z
2
3).ctgx 1
x n, n Z
4
15.
Trigonometrik funksiýalaryň bahalary№
sin
cos
tg
ctg
1
30
2
0
45
0
1
2
2
2
3
2
3
60
0
3
2
2
2
1
3
3
1
1
1
2
3
1
3











 mathematics
mathematics








